Incentivize Contribution and Learn Parameters Too: Federated Learning with Strategic Data Owners

Classical federated learning (FL) assumes that the clients have a limited amount of noisy data with which they voluntarily participate and contribute towards learning a global, more accurate model in a principled manner. The learning happens in a distributed fashion without sharing the data with the center. However, these methods do not consider the incentive of an agent for participating and contributing to the process, given that data collection and running a distributed algorithm is costly for the clients. The question of rationality of contribution has been asked recently in the literature and some results exist that consider this problem. This paper addresses the question of simultaneous parameter learning and incentivizing contribution, which distinguishes it from the extant literature. Our first mechanism incentivizes each client to contribute to the FL process at a Nash equilibrium and simultaneously learn the model parameters. However, this equilibrium outcome can be away from the optimal, where clients contribute with their full data and the algorithm learns the optimal parameters. We propose a second mechanism with monetary transfers that is budget balanced and enables the full data contribution along with optimal parameter learning. Large scale experiments with real (federated) datasets (CIFAR-10, FeMNIST, and Twitter) show that these algorithms converge quite fast in practice, yield good welfare guarantees, and better model performance for all agents.

Near Optimal Best Arm Identification for Clustered Bandits

This work investigates the problem of best arm identification for multi-agent multi-armed bandits. We consider $N$ agents grouped into $M$ clusters, where each cluster solves a stochastic bandit problem. The mapping between agents and bandits is a priori unknown. Each bandit is associated with $K$ arms, and the goal is to identify the best arm for each agent under a $\delta$-probably correct ($\delta$-PC) framework, while minimizing sample complexity and communication overhead. We propose two novel algorithms: Clustering then Best Arm Identification (Cl-BAI) and Best Arm Identification then Clustering (BAI-Cl). Cl-BAI uses a two-phase approach that first clusters agents based on the bandit problems they are learning, followed by identifying the best arm for each cluster. BAI-Cl reverses the sequence by identifying the best arms first and then clustering agents accordingly. Both algorithms leverage the successive elimination framework to ensure computational efficiency and high accuracy. We establish $\delta$-PC guarantees for both methods, derive bounds on their sample complexity, and provide a lower bound for this problem class. Moreover, when $M$ is small (a constant), we show that the sample complexity of a variant of BAI-Cl is minimax optimal in an order-wise sense. Experiments on synthetic and real-world datasets (MovieLens, Yelp) demonstrate the superior performance of the proposed algorithms in terms of sample and communication efficiency, particularly in settings where $M \ll N$.

Learning and Generalization with Mixture Data

In many, if not most, machine learning applications the training data is naturally heterogeneous (e.g. federated learning, adversarial attacks and domain adaptation in neural net training). Data heterogeneity is identified as one of the major challenges in modern day large-scale learning. A classical way to represent heterogeneous data is via a mixture model. In this paper, we study generalization performance and statistical rates when data is sampled from a mixture distribution. We first characterize the heterogeneity of the mixture in terms of the pairwise total variation distance of the sub-population distributions. Thereafter, as a central theme of this paper, we characterize the range where the mixture may be treated as a single (homogeneous) distribution for learning. In particular, we study the generalization performance under the classical PAC framework and the statistical error rates for parametric (linear regression, mixture of hyperplanes) as well as non-parametric (Lipschitz, convex and H\"older-smooth) regression problems. In order to do this, we obtain Rademacher complexity and (local) Gaussian complexity bounds with mixture data, and apply them to get the generalization and convergence rates respectively. We observe that as the (regression) function classes get more complex, the requirement on the pairwise total variation distance gets stringent, which matches our intuition. We also do a finer analysis for the case of mixed linear regression and provide a tight bound on the generalization error in terms of heterogeneity.

Leveraging a Simulator for Learning Causal Representations from Post-Treatment Covariates for CATE

Treatment effect estimation involves assessing the impact of different treatments on individual outcomes. Current methods estimate Conditional Average Treatment Effect (CATE) using observational datasets where covariates are collected before treatment assignment and outcomes are observed afterward, under assumptions like positivity and unconfoundedness. In this paper, we address a scenario where both covariates and outcomes are gathered after treatment. We show that post-treatment covariates render CATE unidentifiable, and recovering CATE requires learning treatment-independent causal representations. Prior work shows that such representations can be learned through contrastive learning if counterfactual supervision is available in observational data. However, since counterfactuals are rare, other works have explored using simulators that offer synthetic counterfactual supervision. Our goal in this paper is to systematically analyze the role of simulators in estimating CATE. We analyze the CATE error of several baselines and highlight their limitations. We then establish a generalization bound that characterizes the CATE error from jointly training on real and simulated distributions, as a function of the real-simulator mismatch. Finally, we introduce SimPONet, a novel method whose loss function is inspired from our generalization bound. We further show how SimPONet adjusts the simulator's influence on the learning objective based on the simulator's relevance to the CATE task. We experiment with various DGPs, by systematically varying the real-simulator distribution gap to evaluate SimPONet's efficacy against state-of-the-art CATE baselines.

Competing Bandits in Decentralized Large Contextual Matching Markets

Sequential learning in a multi-agent resource constrained matching market has received significant interest in the past few years. We study decentralized learning in two-sided matching markets where the demand side (aka players or agents) competes for a `large' supply side (aka arms) with potentially time-varying preferences, to obtain a stable match. Despite a long line of work in the recent past, existing learning algorithms such as Explore-Then-Commit or Upper-Confidence-Bound remain inefficient for this problem. In particular, the per-agent regret achieved by these algorithms scales linearly with the number of arms, $K$. Motivated by the linear contextual bandit framework, we assume that for each agent an arm-mean can be represented by a linear function of a known feature vector and an unknown (agent-specific) parameter. Moreover, our setup captures the essence of a dynamic (non-stationary) matching market where the preferences over arms change over time. Our proposed algorithms achieve instance-dependent logarithmic regret, scaling independently of the number of arms, $K$.

Explore-then-Commit Algorithms for Decentralized Two-Sided Matching Markets

Online learning in a decentralized two-sided matching markets, where the demand-side (players) compete to match with the supply-side (arms), has received substantial interest because it abstracts out the complex interactions in matching platforms (e.g. UpWork, TaskRabbit). However, past works assume that each arm knows their preference ranking over the players (one-sided learning), and each player aim to learn the preference over arms through successive interactions. Moreover, several (impractical) assumptions on the problem are usually made for theoretical tractability such as broadcast player-arm match Liu et al. (2020; 2021); Kong & Li (2023) or serial dictatorship Sankararaman et al. (2021); Basu et al. (2021); Ghosh et al. (2022). In this paper, we study a decentralized two-sided matching market, where we do not assume that the preference ranking over players are known to the arms apriori. Furthermore, we do not have any structural assumptions on the problem. We propose a multi-phase explore-then-commit type algorithm namely epoch-based CA-ETC (collision avoidance explore then commit) (\texttt{CA-ETC} in short) for this problem that does not require any communication across agents (players and arms) and hence decentralized. We show that for the initial epoch length of $T_{\circ}$ and subsequent epoch-lengths of $2^{l/\gamma} T_{\circ}$ (for the $l-$th epoch with $\gamma \in (0,1)$ as an input parameter to the algorithm), \texttt{CA-ETC} yields a player optimal expected regret of $\mathcal{O}\left(T_{\circ} (\frac{K \log T}{T_{\circ} \Delta^2})^{1/\gamma} + T_{\circ} (\frac{T}{T_{\circ}})^\gamma\right)$ for the $i$-th player, where $T$ is the learning horizon, $K$ is the number of arms and $\Delta$ is an appropriately defined problem gap. Furthermore, we propose a blackboard communication based baseline achieving logarithmic regret in $T$.

PairNet: Training with Observed Pairs to Estimate Individual Treatment Effect

Given a dataset of individuals each described by a covariate vector, a treatment, and an observed outcome on the treatment, the goal of the individual treatment effect (ITE) estimation task is to predict outcome changes resulting from a change in treatment. A fundamental challenge is that in the observational data, a covariate's outcome is observed only under one treatment, whereas we need to infer the difference in outcomes under two different treatments. Several existing approaches address this issue through training with inferred pseudo-outcomes, but their success relies on the quality of these pseudo-outcomes. We propose PairNet, a novel ITE estimation training strategy that minimizes losses over pairs of examples based on their factual observed outcomes. Theoretical analysis for binary treatments reveals that PairNet is a consistent estimator of ITE risk, and achieves smaller generalization error than baseline models. Empirical comparison with thirteen existing methods across eight benchmarks, covering both discrete and continuous treatments, shows that PairNet achieves significantly lower ITE error compared to the baselines. Also, it is model-agnostic and easy to implement.

Agnostic Learning of Mixed Linear Regressions with EM and AM Algorithms

Mixed linear regression is a well-studied problem in parametric statistics and machine learning. Given a set of samples, tuples of covariates and labels, the task of mixed linear regression is to find a small list of linear relationships that best fit the samples. Usually it is assumed that the label is generated stochastically by randomly selecting one of two or more linear functions, applying this chosen function to the covariates, and potentially introducing noise to the result. In that situation, the objective is to estimate the ground-truth linear functions up to some parameter error. The popular expectation maximization (EM) and alternating minimization (AM) algorithms have been previously analyzed for this. In this paper, we consider the more general problem of agnostic learning of mixed linear regression from samples, without such generative models. In particular, we show that the AM and EM algorithms, under standard conditions of separability and good initialization, lead to agnostic learning in mixed linear regression by converging to the population loss minimizers, for suitably defined loss functions. In some sense, this shows the strength of AM and EM algorithms that converges to ``optimal solutions'' even in the absence of realizable generative models.

Optimal Compression of Unit Norm Vectors in the High Distortion Regime

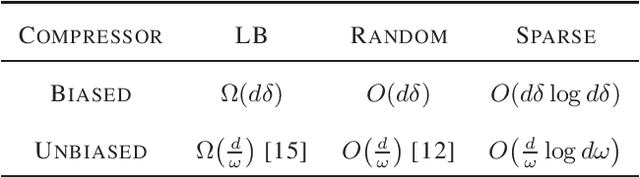
Motivated by the need for communication-efficient distributed learning, we investigate the method for compressing a unit norm vector into the minimum number of bits, while still allowing for some acceptable level of distortion in recovery. This problem has been explored in the rate-distortion/covering code literature, but our focus is exclusively on the "high-distortion" regime. We approach this problem in a worst-case scenario, without any prior information on the vector, but allowing for the use of randomized compression maps. Our study considers both biased and unbiased compression methods and determines the optimal compression rates. It turns out that simple compression schemes are nearly optimal in this scenario. While the results are a mix of new and known, they are compiled in this paper for completeness.

Exploration in Linear Bandits with Rich Action Sets and its Implications for Inference

We present a non-asymptotic lower bound on the eigenspectrum of the design matrix generated by any linear bandit algorithm with sub-linear regret when the action set has well-behaved curvature. Specifically, we show that the minimum eigenvalue of the expected design matrix grows as $\Omega(\sqrt{n})$ whenever the expected cumulative regret of the algorithm is $O(\sqrt{n})$, where $n$ is the learning horizon, and the action-space has a constant Hessian around the optimal arm. This shows that such action-spaces force a polynomial lower bound rather than a logarithmic lower bound, as shown by \cite{lattimore2017end}, in discrete (i.e., well-separated) action spaces. Furthermore, while the previous result is shown to hold only in the asymptotic regime (as $n \to \infty$), our result for these ``locally rich" action spaces is any-time. Additionally, under a mild technical assumption, we obtain a similar lower bound on the minimum eigen value holding with high probability. We apply our result to two practical scenarios -- \emph{model selection} and \emph{clustering} in linear bandits. For model selection, we show that an epoch-based linear bandit algorithm adapts to the true model complexity at a rate exponential in the number of epochs, by virtue of our novel spectral bound. For clustering, we consider a multi agent framework where we show, by leveraging the spectral result, that no forced exploration is necessary -- the agents can run a linear bandit algorithm and estimate their underlying parameters at once, and hence incur a low regret.