Solution Path of Time-varying Markov Random Fields with Discrete Regularization

We study the problem of inferring sparse time-varying Markov random fields (MRFs) with different discrete and temporal regularizations on the parameters. Due to the intractability of discrete regularization, most approaches for solving this problem rely on the so-called maximum-likelihood estimation (MLE) with relaxed regularization, which neither results in ideal statistical properties nor scale to the dimensions encountered in realistic settings. In this paper, we address these challenges by departing from the MLE paradigm and resorting to a new class of constrained optimization problems with exact, discrete regularization to promote sparsity in the estimated parameters. Despite the nonconvex and discrete nature of our formulation, we show that it can be solved efficiently and parametrically for all sparsity levels. More specifically, we show that the entire solution path of the time-varying MRF for all sparsity levels can be obtained in $\mathcal{O}(pT^3)$, where $T$ is the number of time steps and $p$ is the number of unknown parameters at any given time. The efficient and parametric characterization of the solution path renders our approach highly suitable for cross-validation, where parameter estimation is required for varying regularization values. Despite its simplicity and efficiency, we show that our proposed approach achieves provably small estimation error for different classes of time-varying MRFs, namely Gaussian and discrete MRFs, with as few as one sample per time. Utilizing our algorithm, we can recover the complete solution path for instances of time-varying MRFs featuring over 30 million variables in less than 12 minutes on a standard laptop computer. Our code is available at \url{https://sites.google.com/usc.edu/gomez/data}.

Memory-Aware Partitioning of Machine Learning Applications for Optimal Energy Use in Batteryless Systems

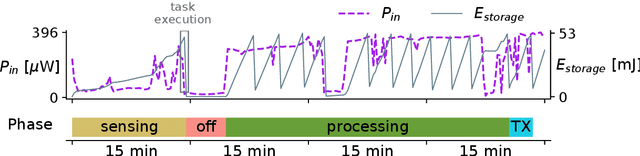
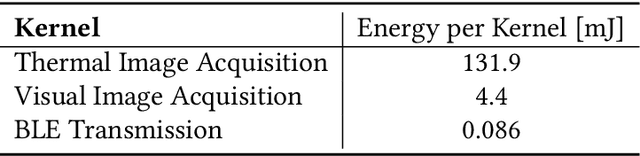
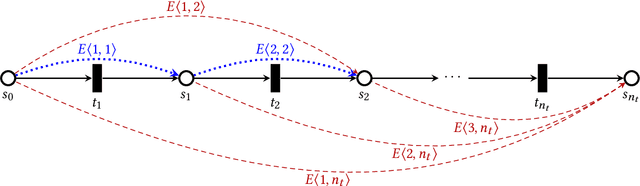
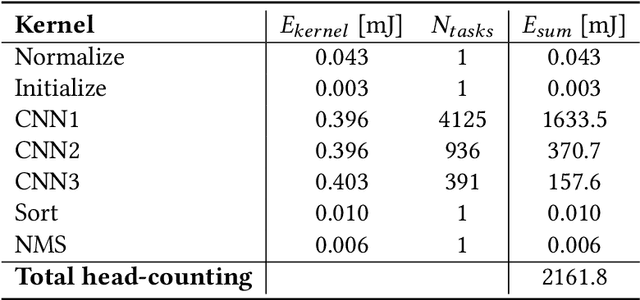
Sensing systems powered by energy harvesting have traditionally been designed to tolerate long periods without energy. As the Internet of Things (IoT) evolves towards a more transient and opportunistic execution paradigm, reducing energy storage costs will be key for its economic and ecologic viability. However, decreasing energy storage in harvesting systems introduces reliability issues. Transducers only produce intermittent energy at low voltage and current levels, making guaranteed task completion a challenge. Existing ad hoc methods overcome this by buffering enough energy either for single tasks, incurring large data-retention overheads, or for one full application cycle, requiring a large energy buffer. We present Julienning: an automated method for optimizing the total energy cost of batteryless applications. Using a custom specification model, developers can describe transient applications as a set of atomically executed kernels with explicit data dependencies. Our optimization flow can partition data- and energy-intensive applications into multiple execution cycles with bounded energy consumption. By leveraging interkernel data dependencies, these energy-bounded execution cycles minimize the number of system activations and nonvolatile data transfers, and thus the total energy overhead. We validate our methodology with two batteryless cameras running energy-intensive machine learning applications. Results demonstrate that compared to ad hoc solutions, our method can reduce the required energy storage by over 94% while only incurring a 0.12% energy overhead.

Scalable Inference of Sparsely-changing Markov Random Fields with Strong Statistical Guarantees

In this paper, we study the problem of inferring time-varying Markov random fields (MRF), where the underlying graphical model is both sparse and changes sparsely over time. Most of the existing methods for the inference of time-varying MRFs rely on the regularized maximum likelihood estimation (MLE), that typically suffer from weak statistical guarantees and high computational time. Instead, we introduce a new class of constrained optimization problems for the inference of sparsely-changing MRFs. The proposed optimization problem is formulated based on the exact $\ell_0$ regularization, and can be solved in near-linear time and memory. Moreover, we show that the proposed estimator enjoys a provably small estimation error. As a special case, we derive sharp statistical guarantees for the inference of sparsely-changing Gaussian MRFs (GMRF) in the high-dimensional regime, showing that such problems can be learned with as few as one sample per time. Our proposed method is extremely efficient in practice: it can accurately estimate sparsely-changing graphical models with more than 500 million variables in less than one hour.

Supermodularity and valid inequalities for quadratic optimization with indicators

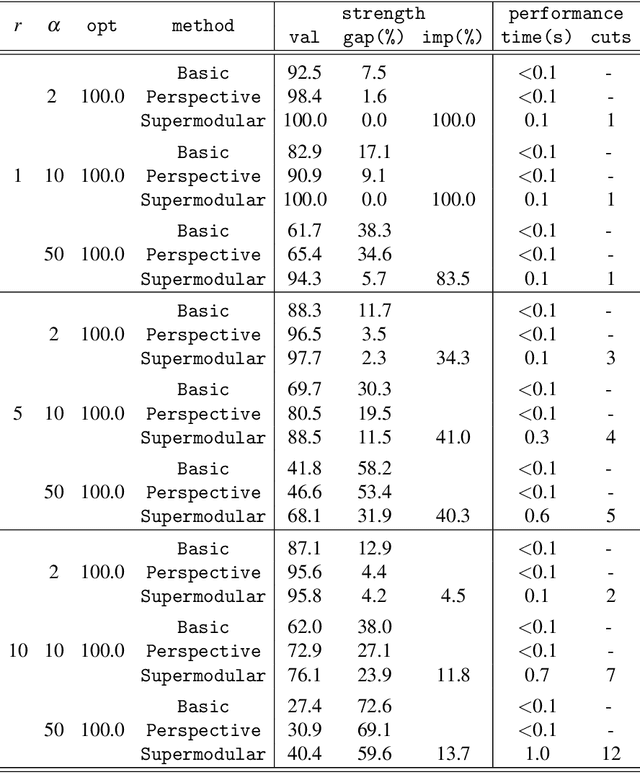
We study the minimization of a rank-one quadratic with indicators and show that the underlying set function obtained by projecting out the continuous variables is supermodular. Although supermodular minimization is, in general, difficult, the specific set function for the rank-one quadratic can be minimized in linear time. We show that the convex hull of the epigraph of the quadratic can be obtaining from inequalities for the underlying supermodular set function by lifting them into nonlinear inequalities in the original space of variables. Explicit forms of the convex-hull description are given, both in the original space of variables and in an extended formulation via conic quadratic-representable inequalities, along with a polynomial separation algorithm. Computational experiments indicate that the lifted supermodular inequalities in conic quadratic form are quite effective in reducing the integrality gap for quadratic optimization with indicators.

Ideal formulations for constrained convex optimization problems with indicator variables

Motivated by modern regression applications, in this paper, we study the convexification of a class of convex optimization problems with indicator variables and combinatorial constraints on the indicators. Unlike most of the previous work on convexification of sparse regression problems, we simultaneously consider the nonlinear non-separable objective, indicator variables, and combinatorial constraints. Specifically, we give the convex hull description of the epigraph of the composition of a one-dimensional convex function and an affine function under arbitrary combinatorial constraints. As special cases of this result, we derive ideal convexifications for problems with hierarchy, multi-collinearity, and sparsity constraints. Moreover, we also give a short proof that for a separable objective function, the perspective reformulation is ideal independent from the constraints of the problem. Our computational experiments with regression problems under hierarchy constraints on real datasets demonstrate the potential of the proposed approach in improving the relaxation quality without significant computational overhead.

Learning Optimal Classification Trees: Strong Max-Flow Formulations

We consider the problem of learning optimal binary classification trees. Literature on the topic has burgeoned in recent years, motivated both by the empirical suboptimality of heuristic approaches and the tremendous improvements in mixed-integer programming (MIP) technology. Yet, existing approaches from the literature do not leverage the power of MIP to its full extent. Indeed, they rely on weak formulations, resulting in slow convergence and large optimality gaps. To fill this gap in the literature, we propose a flow-based MIP formulation for optimal binary classification trees that has a stronger linear programming relaxation. Our formulation presents an attractive decomposable structure. We exploit this structure and max-flow/min-cut duality to derive a Benders' decomposition method, which scales to larger instances. We conduct extensive computational experiments on standard benchmark datasets on which we show that our proposed approaches are 50 times faster than state-of-the art MIP-based techniques and improve out of sample performance up to 13.8%.

Rank-one Convexification for Sparse Regression

Sparse regression models are increasingly prevalent due to their ease of interpretability and superior out-of-sample performance. However, the exact model of sparse regression with an $\ell_0$ constraint restricting the support of the estimators is a challenging non-convex optimization problem. In this paper, we derive new strong convex relaxations for sparse regression. These relaxations are based on the ideal (convex-hull) formulations for rank-one quadratic terms with indicator variables. The new relaxations can be formulated as semidefinite optimization problems in an extended space and are stronger and more general than the state-of-the-art formulations, including the perspective reformulation and formulations with the reverse Huber penalty and the minimax concave penalty functions. Furthermore, the proposed rank-one strengthening can be interpreted as a non-separable, non-convex sparsity-inducing regularizer, which dynamically adjusts its penalty according to the shape of the error function. In our computational experiments with benchmark datasets, the proposed conic formulations are solved within seconds and result in near-optimal solutions (with 0.4\% optimality gap) for non-convex $\ell_0$-problems. Moreover, the resulting estimators also outperform alternative convex approaches from a statistical viewpoint, achieving high prediction accuracy and good interpretability.

Sparse and Smooth Signal Estimation: Convexification of L0 Formulations

Signal estimation problems with smoothness and sparsity priors can be naturally modeled as quadratic optimization with $\ell_0$-"norm" constraints. Since such problems are non-convex and hard-to-solve, the standard approach is, instead, to tackle their convex surrogates based on $\ell_1$-norm relaxations. In this paper, we propose new iterative conic quadratic relaxations that exploit not only the $\ell_0$-"norm" terms, but also the fitness and smoothness functions. The iterative convexification approach substantially closes the gap between the $\ell_0$-"norm" and its $\ell_1$ surrogate. Experiments using an off-the-shelf conic quadratic solver on synthetic as well as real datasets indicate that the proposed iterative convex relaxations lead to significantly better estimators than $\ell_1$-norm while preserving the computational efficiency. In addition, the parameters of the model and the resulting estimators are easily interpretable.

Intrusion Prevention and Detection in Grid Computing - The ALICE Case

Grids allow users flexible on-demand usage of computing resources through remote communication networks. A remarkable example of a Grid in High Energy Physics (HEP) research is used in the ALICE experiment at European Organization for Nuclear Research CERN. Physicists can submit jobs used to process the huge amount of particle collision data produced by the Large Hadron Collider (LHC). Grids face complex security challenges. They are interesting targets for attackers seeking for huge computational resources. Since users can execute arbitrary code in the worker nodes on the Grid sites, special care should be put in this environment. Automatic tools to harden and monitor this scenario are required. Currently, there is no integrated solution for such requirement. This paper describes a new security framework to allow execution of job payloads in a sandboxed context. It also allows process behavior monitoring to detect intrusions, even when new attack methods or zero day vulnerabilities are exploited, by a Machine Learning approach. We plan to implement the proposed framework as a software prototype that will be tested as a component of the ALICE Grid middleware.