On Deciding the Data Complexity of Answering Linear Monadic Datalog Queries with LTL Operators(Extended Version)

Our concern is the data complexity of answering linear monadic datalog queries whose atoms in the rule bodies can be prefixed by operators of linear temporal logic LTL. We first observe that, for data complexity, answering any connected query with operators $\bigcirc/\bigcirc^-$ (at the next/previous moment) is either in AC0, or in $ACC0\!\setminus\!AC0$, or $NC^1$-complete, or LogSpace-hard and in NLogSpace. Then we show that the problem of deciding LogSpace-hardness of answering such queries is PSpace-complete, while checking membership in the classes AC0 and ACC0 as well as $NC^1$-completeness can be done in ExpSpace. Finally, we prove that membership in AC0 or in ACC0, $NC^1$-completeness, and LogSpace-hardness are undecidable for queries with operators $\Diamond_f/\Diamond_p$ (sometime in the future/past) provided that $NC^1 \ne NLogSpace$, and $LogSpace \ne NLogSpace$.

Automated Reasoning in Temporal DL-Lite

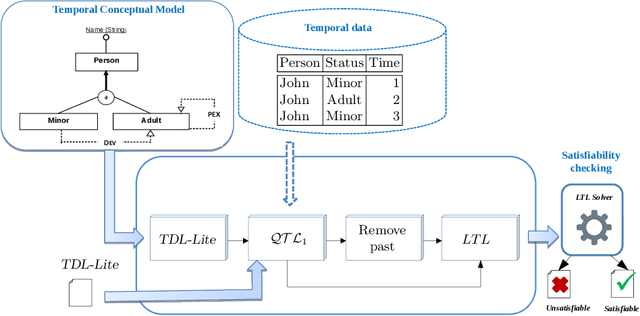
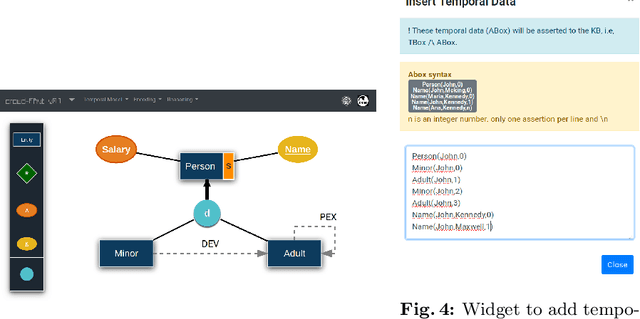
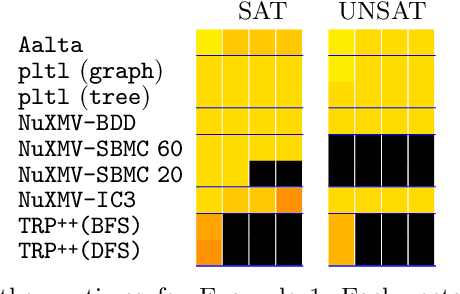
This paper investigates the feasibility of automated reasoning over temporal DL-Lite (TDL-Lite) knowledge bases (KBs). We test the usage of off-the-shelf LTL reasoners to check satisfiability of TDL-Lite KBs. In particular, we test the robustness and the scalability of reasoners when dealing with TDL-Lite TBoxes paired with a temporal ABox. We conduct various experiments to analyse the performance of different reasoners by randomly generating TDL-Lite KBs and then measuring the running time and the size of the translations. Furthermore, in an effort to make the usage of TDL-Lite KBs a reality, we present a fully fledged tool with a graphical interface to design them. Our interface is based on conceptual modelling principles and it is integrated with our translation tool and a temporal reasoner.

A Decidable Very Expressive Description Logic for Databases (Extended Version)

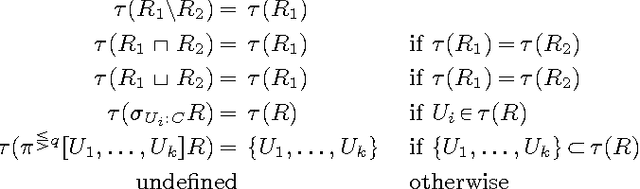
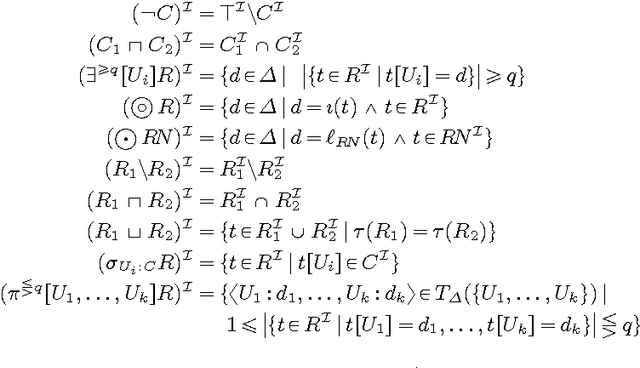
We introduce $\mathcal{DLR}^+$, an extension of the n-ary propositionally closed description logic $\mathcal{DLR}$ to deal with attribute-labelled tuples (generalising the positional notation), projections of relations, and global and local objectification of relations, able to express inclusion, functional, key, and external uniqueness dependencies. The logic is equipped with both TBox and ABox axioms. We show how a simple syntactic restriction on the appearance of projections sharing common attributes in a $\mathcal{DLR}^+$ knowledge base makes reasoning in the language decidable with the same computational complexity as $\mathcal{DLR}$. The obtained $\mathcal{DLR}^\pm$ n-ary description logic is able to encode more thoroughly conceptual data models such as EER, UML, and ORM.

Extending DLR with Labelled Tuples, Projections, Functional Dependencies and Objectification (full version)

We introduce an extension of the n-ary description logic DLR to deal with attribute-labelled tuples (generalising the positional notation), with arbitrary projections of relations (inclusion dependencies), generic functional dependencies and with global and local objectification (reifying relations or their projections). We show how a simple syntactic condition on the appearance of projections and functional dependencies in a knowledge base makes the language decidable without increasing the computational complexity of the basic DLR language.

A Cookbook for Temporal Conceptual Data Modelling with Description Logics

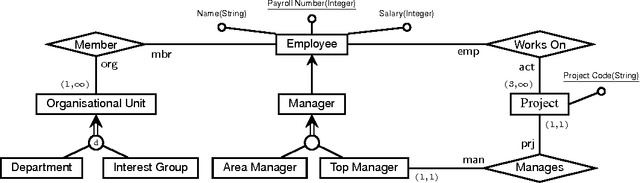
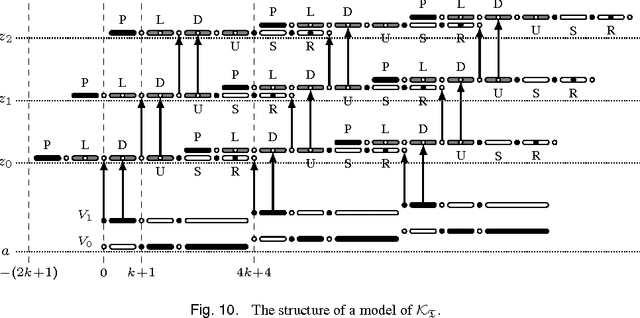
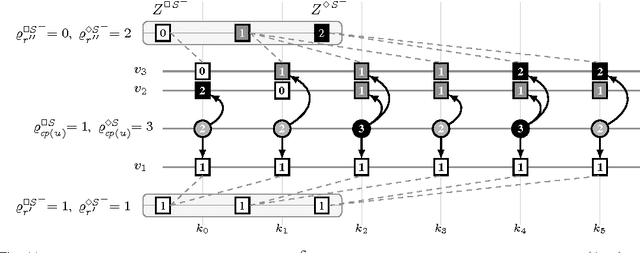
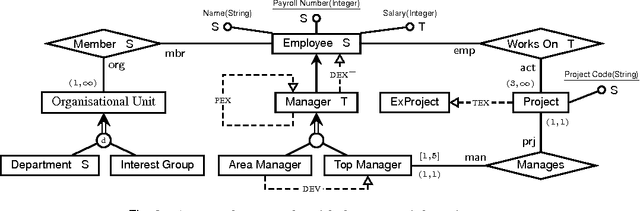
We design temporal description logics suitable for reasoning about temporal conceptual data models and investigate their computational complexity. Our formalisms are based on DL-Lite logics with three types of concept inclusions (ranging from atomic concept inclusions and disjointness to the full Booleans), as well as cardinality constraints and role inclusions. In the temporal dimension, they capture future and past temporal operators on concepts, flexible and rigid roles, the operators `always' and `some time' on roles, data assertions for particular moments of time and global concept inclusions. The logics are interpreted over the Cartesian products of object domains and the flow of time (Z,<), satisfying the constant domain assumption. We prove that the most expressive of our temporal description logics (which can capture lifespan cardinalities and either qualitative or quantitative evolution constraints) turn out to be undecidable. However, by omitting some of the temporal operators on concepts/roles or by restricting the form of concept inclusions we obtain logics whose complexity ranges between PSpace and NLogSpace. These positive results were obtained by reduction to various clausal fragments of propositional temporal logic, which opens a way to employ propositional or first-order temporal provers for reasoning about temporal data models.

The DL-Lite Family and Relations

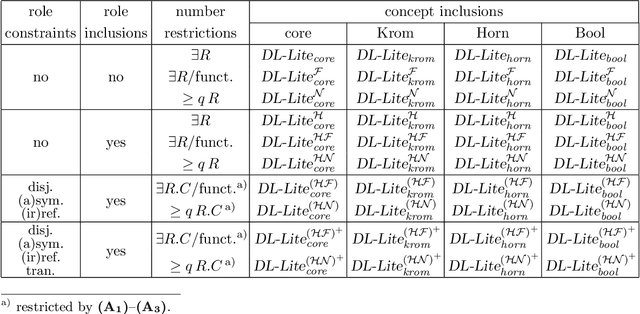
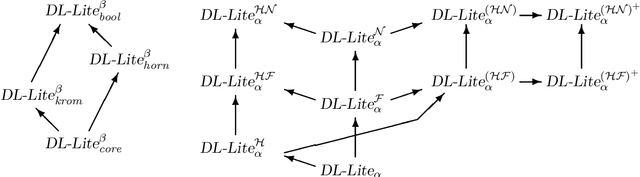
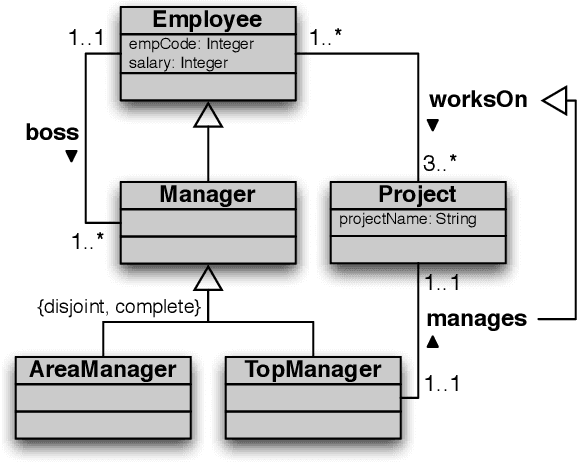
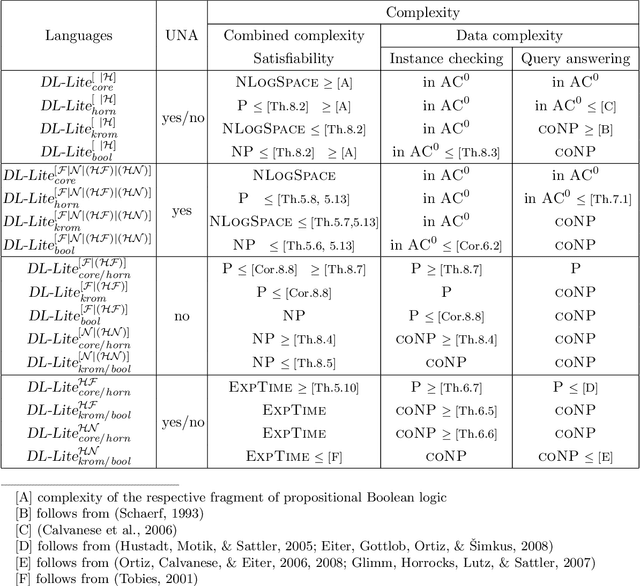
The recently introduced series of description logics under the common moniker DL-Lite has attracted attention of the description logic and semantic web communities due to the low computational complexity of inference, on the one hand, and the ability to represent conceptual modeling formalisms, on the other. The main aim of this article is to carry out a thorough and systematic investigation of inference in extensions of the original DL-Lite logics along five axes: by (i) adding the Boolean connectives and (ii) number restrictions to concept constructs, (iii) allowing role hierarchies, (iv) allowing role disjointness, symmetry, asymmetry, reflexivity, irreflexivity and transitivity constraints, and (v) adopting or dropping the unique same assumption. We analyze the combined complexity of satisfiability for the resulting logics, as well as the data complexity of instance checking and answering positive existential queries. Our approach is based on embedding DL-Lite logics in suitable fragments of the one-variable first-order logic, which provides useful insights into their properties and, in particular, computational behavior.

Temporal Description Logic for Ontology-Based Data Access (Extended Version)

Our aim is to investigate ontology-based data access over temporal data with validity time and ontologies capable of temporal conceptual modelling. To this end, we design a temporal description logic, TQL, that extends the standard ontology language OWL 2 QL, provides basic means for temporal conceptual modelling and ensures first-order rewritability of conjunctive queries for suitably defined data instances with validity time.