On Deciding the Data Complexity of Answering Linear Monadic Datalog Queries with LTL Operators(Extended Version)

Our concern is the data complexity of answering linear monadic datalog queries whose atoms in the rule bodies can be prefixed by operators of linear temporal logic LTL. We first observe that, for data complexity, answering any connected query with operators $\bigcirc/\bigcirc^-$ (at the next/previous moment) is either in AC0, or in $ACC0\!\setminus\!AC0$, or $NC^1$-complete, or LogSpace-hard and in NLogSpace. Then we show that the problem of deciding LogSpace-hardness of answering such queries is PSpace-complete, while checking membership in the classes AC0 and ACC0 as well as $NC^1$-completeness can be done in ExpSpace. Finally, we prove that membership in AC0 or in ACC0, $NC^1$-completeness, and LogSpace-hardness are undecidable for queries with operators $\Diamond_f/\Diamond_p$ (sometime in the future/past) provided that $NC^1 \ne NLogSpace$, and $LogSpace \ne NLogSpace$.

Temporalising Unique Characterisability and Learnability of Ontology-Mediated Queries

Recently, the study of the unique characterisability and learnability of database queries by means of examples has been extended to ontology-mediated queries. Here, we study in how far the obtained results can be lifted to temporalised ontology-mediated queries. We provide a systematic introduction to the relevant approaches in the non-temporal case and then show general transfer results pinpointing under which conditions existing results can be lifted to temporalised queries.

Reverse Engineering of Temporal Queries Mediated by LTL Ontologies

In reverse engineering of database queries, we aim to construct a query from a given set of answers and non-answers; it can then be used to explore the data further or as an explanation of the answers and non-answers. We investigate this query-by-example problem for queries formulated in positive fragments of linear temporal logic LTL over timestamped data, focusing on the design of suitable query languages and the combined and data complexity of deciding whether there exists a query in the given language that separates the given answers from non-answers. We consider both plain LTL queries and those mediated by LTL-ontologies.

A data complexity and rewritability tetrachotomy of ontology-mediated queries with a covering axiom

Aiming to understand the data complexity of answering conjunctive queries mediated by an axiom stating that a class is covered by the union of two other classes, we show that deciding their first-order rewritability is PSpace-hard and obtain a number of sufficient conditions for membership in AC0, L, NL, and P. Our main result is a complete syntactic AC0//NL/P/coNP tetrachotomy of path queries under the assumption that the covering classes are disjoint.

Data Complexity and Rewritability of Ontology-Mediated Queries in Metric Temporal Logic under the Event-Based Semantics (Full Version)

We investigate the data complexity of answering queries mediated by metric temporal logic ontologies under the event-based semantics assuming that data instances are finite timed words timestamped with binary fractions. We identify classes of ontology-mediated queries answering which can be done in AC0, NC1, L, NL, P, and coNP for data complexity, provide their rewritings to first-order logic and its extensions with primitive recursion, transitive closure or datalog, and establish lower complexity bounds.

Query Inseparability for ALC Ontologies

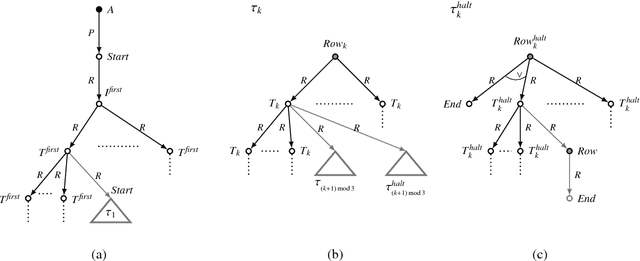
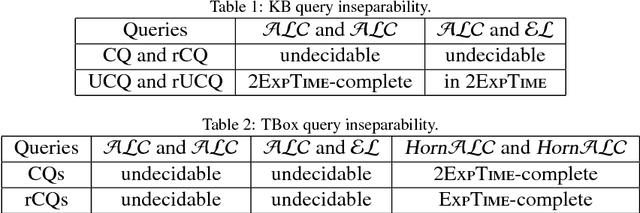
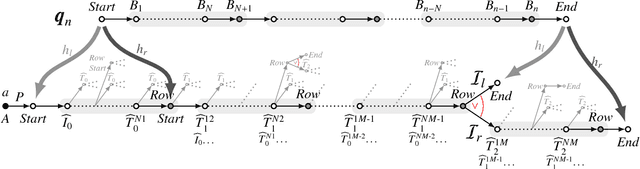
We investigate the problem whether two ALC ontologies are indistinguishable (or inseparable) by means of queries in a given signature, which is fundamental for ontology engineering tasks such as ontology versioning, modularisation, update, and forgetting. We consider both knowledge base (KB) and TBox inseparability. For KBs, we give model-theoretic criteria in terms of (finite partial) homomorphisms and products and prove that this problem is undecidable for conjunctive queries (CQs), but 2ExpTime-complete for unions of CQs (UCQs). The same results hold if (U)CQs are replaced by rooted (U)CQs, where every variable is connected to an answer variable. We also show that inseparability by CQs is still undecidable if one KB is given in the lightweight DL EL and if no restrictions are imposed on the signature of the CQs. We also consider the problem whether two ALC TBoxes give the same answers to any query over any ABox in a given signature and show that, for CQs, this problem is undecidable, too. We then develop model-theoretic criteria for Horn-ALC TBoxes and show using tree automata that, in contrast, inseparability becomes decidable and 2ExpTime-complete, even ExpTime-complete when restricted to (unions of) rooted CQs.

Inseparability and Conservative Extensions of Description Logic Ontologies: A Survey

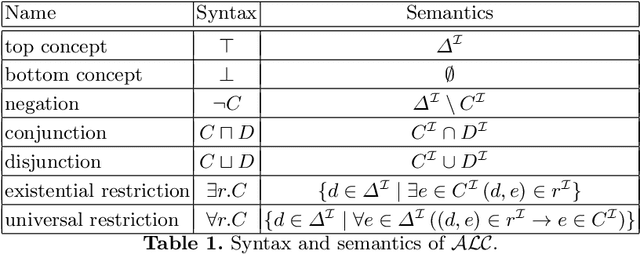
The question whether an ontology can safely be replaced by another, possibly simpler, one is fundamental for many ontology engineering and maintenance tasks. It underpins, for example, ontology versioning, ontology modularization, forgetting, and knowledge exchange. What safe replacement means depends on the intended application of the ontology. If, for example, it is used to query data, then the answers to any relevant ontology-mediated query should be the same over any relevant data set; if, in contrast, the ontology is used for conceptual reasoning, then the entailed subsumptions between concept expressions should coincide. This gives rise to different notions of ontology inseparability such as query inseparability and concept inseparability, which generalize corresponding notions of conservative extensions. We survey results on various notions of inseparability in the context of description logic ontologies, discussing their applications, useful model-theoretic characterizations, algorithms for determining whether two ontologies are inseparable (and, sometimes, for computing the difference between them if they are not), and the computational complexity of this problem.

Ontology-Mediated Queries: Combined Complexity and Succinctness of Rewritings via Circuit Complexity

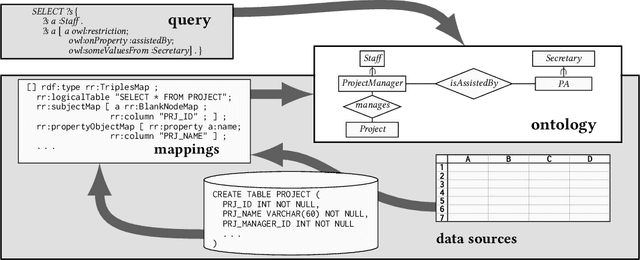
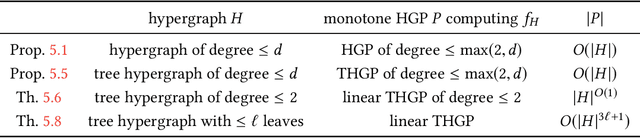
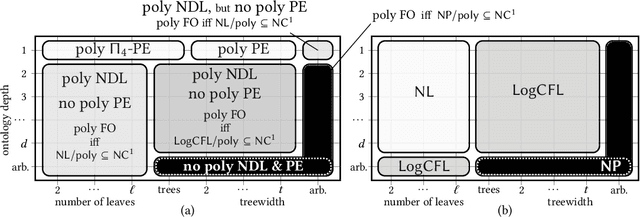
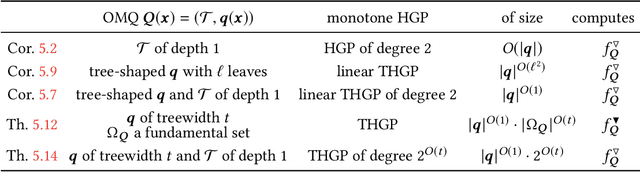
We give solutions to two fundamental computational problems in ontology-based data access with the W3C standard ontology language OWL 2 QL: the succinctness problem for first-order rewritings of ontology-mediated queries (OMQs), and the complexity problem for OMQ answering. We classify OMQs according to the shape of their conjunctive queries (treewidth, the number of leaves) and the existential depth of their ontologies. For each of these classes, we determine the combined complexity of OMQ answering, and whether all OMQs in the class have polynomial-size first-order, positive existential, and nonrecursive datalog rewritings. We obtain the succinctness results using hypergraph programs, a new computational model for Boolean functions, which makes it possible to connect the size of OMQ rewritings and circuit complexity.

A Cookbook for Temporal Conceptual Data Modelling with Description Logics

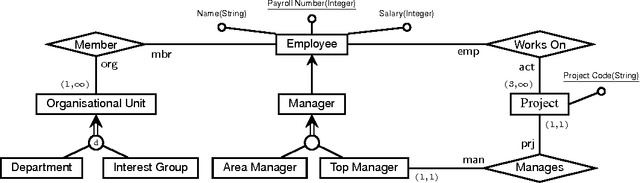
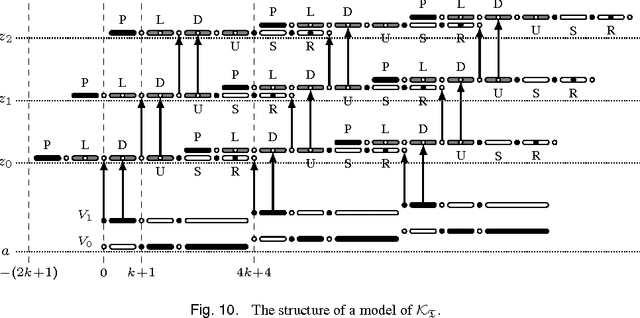
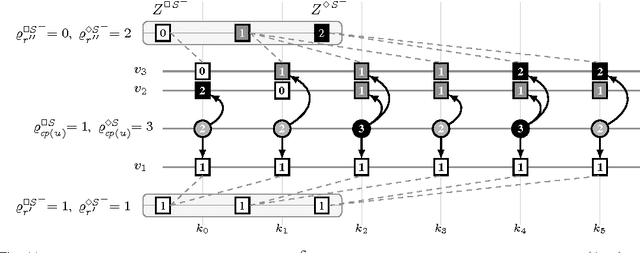
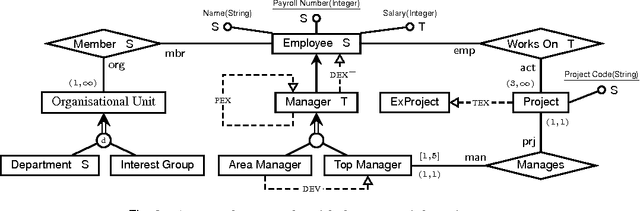
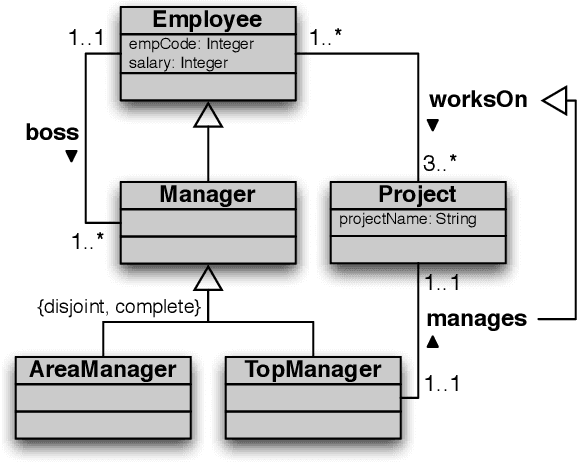
We design temporal description logics suitable for reasoning about temporal conceptual data models and investigate their computational complexity. Our formalisms are based on DL-Lite logics with three types of concept inclusions (ranging from atomic concept inclusions and disjointness to the full Booleans), as well as cardinality constraints and role inclusions. In the temporal dimension, they capture future and past temporal operators on concepts, flexible and rigid roles, the operators `always' and `some time' on roles, data assertions for particular moments of time and global concept inclusions. The logics are interpreted over the Cartesian products of object domains and the flow of time (Z,<), satisfying the constant domain assumption. We prove that the most expressive of our temporal description logics (which can capture lifespan cardinalities and either qualitative or quantitative evolution constraints) turn out to be undecidable. However, by omitting some of the temporal operators on concepts/roles or by restricting the form of concept inclusions we obtain logics whose complexity ranges between PSpace and NLogSpace. These positive results were obtained by reduction to various clausal fragments of propositional temporal logic, which opens a way to employ propositional or first-order temporal provers for reasoning about temporal data models.

The DL-Lite Family and Relations

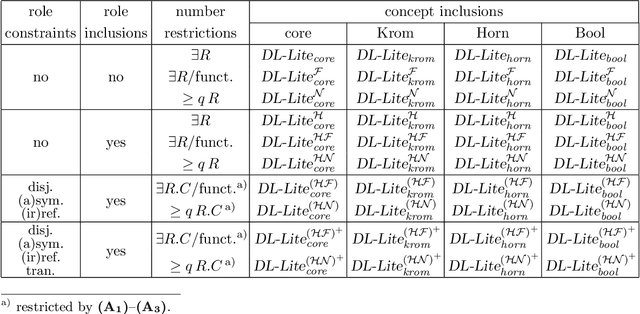
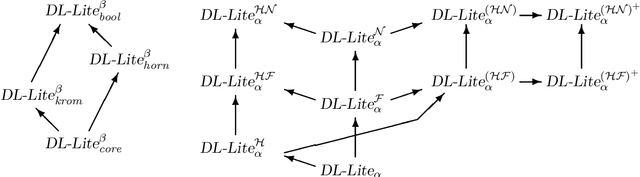
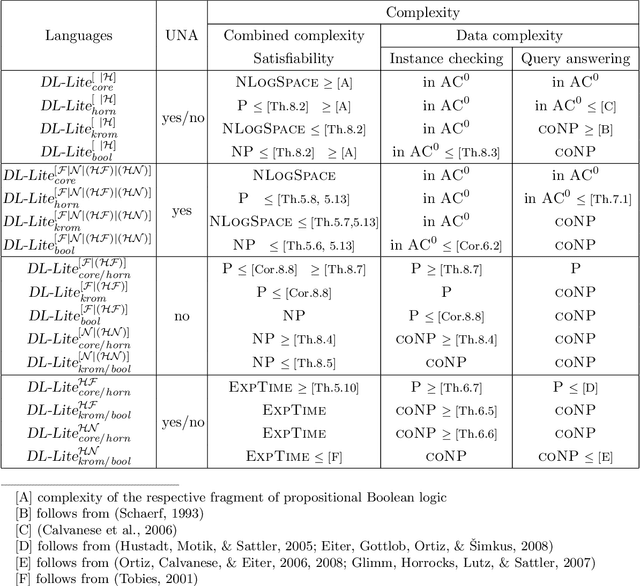
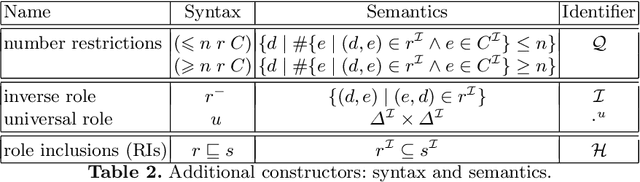
The recently introduced series of description logics under the common moniker DL-Lite has attracted attention of the description logic and semantic web communities due to the low computational complexity of inference, on the one hand, and the ability to represent conceptual modeling formalisms, on the other. The main aim of this article is to carry out a thorough and systematic investigation of inference in extensions of the original DL-Lite logics along five axes: by (i) adding the Boolean connectives and (ii) number restrictions to concept constructs, (iii) allowing role hierarchies, (iv) allowing role disjointness, symmetry, asymmetry, reflexivity, irreflexivity and transitivity constraints, and (v) adopting or dropping the unique same assumption. We analyze the combined complexity of satisfiability for the resulting logics, as well as the data complexity of instance checking and answering positive existential queries. Our approach is based on embedding DL-Lite logics in suitable fragments of the one-variable first-order logic, which provides useful insights into their properties and, in particular, computational behavior.