LayerSkip: Enabling Early Exit Inference and Self-Speculative Decoding

We present LayerSkip, an end-to-end solution to speed-up inference of large language models (LLMs). First, during training we apply layer dropout, with low dropout rates for earlier layers and higher dropout rates for later layers, and an early exit loss where all transformer layers share the same exit. Second, during inference, we show that this training recipe increases the accuracy of early exit at earlier layers, without adding any auxiliary layers or modules to the model. Third, we present a novel self-speculative decoding solution where we exit at early layers and verify and correct with remaining layers of the model. Our proposed self-speculative decoding approach has less memory footprint than other speculative decoding approaches and benefits from shared compute and activations of the draft and verification stages. We run experiments on different Llama model sizes on different types of training: pretraining from scratch, continual pretraining, finetuning on specific data domain, and finetuning on specific task. We implement our inference solution and show speedups of up to 2.16x on summarization for CNN/DM documents, 1.82x on coding, and 2.0x on TOPv2 semantic parsing task.

Simultaneous Dimensionality Reduction: A Data Efficient Approach for Multimodal Representations Learning

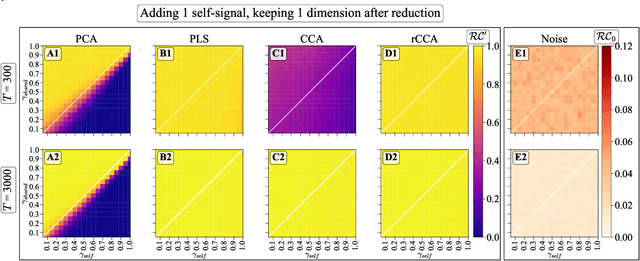
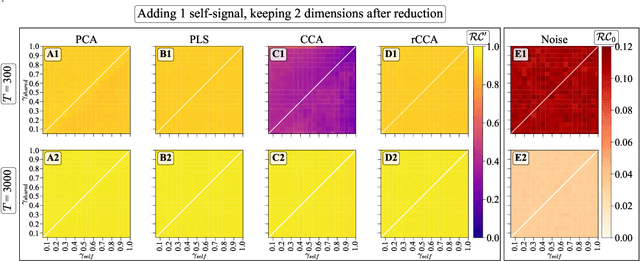
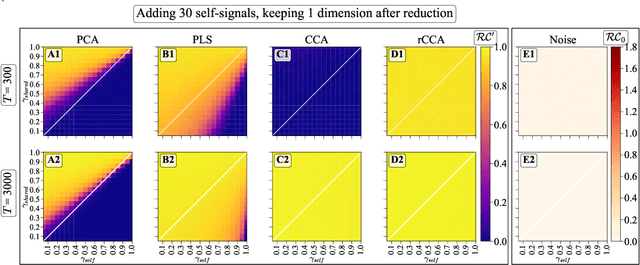
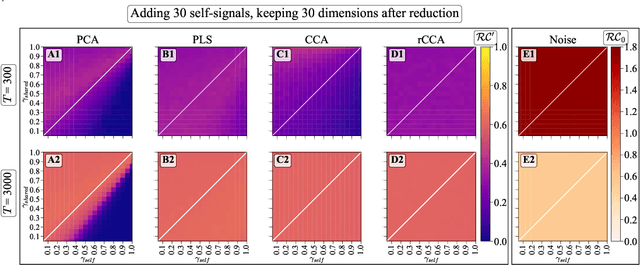
We explore two primary classes of approaches to dimensionality reduction (DR): Independent Dimensionality Reduction (IDR) and Simultaneous Dimensionality Reduction (SDR). In IDR methods, of which Principal Components Analysis is a paradigmatic example, each modality is compressed independently, striving to retain as much variation within each modality as possible. In contrast, in SDR, one simultaneously compresses the modalities to maximize the covariation between the reduced descriptions while paying less attention to how much individual variation is preserved. Paradigmatic examples include Partial Least Squares and Canonical Correlations Analysis. Even though these DR methods are a staple of statistics, their relative accuracy and data set size requirements are poorly understood. We introduce a generative linear model to synthesize multimodal data with known variance and covariance structures to examine these questions. We assess the accuracy of the reconstruction of the covariance structures as a function of the number of samples, signal-to-noise ratio, and the number of varying and covarying signals in the data. Using numerical experiments, we demonstrate that linear SDR methods consistently outperform linear IDR methods and yield higher-quality, more succinct reduced-dimensional representations with smaller datasets. Remarkably, regularized CCA can identify low-dimensional weak covarying structures even when the number of samples is much smaller than the dimensionality of the data, which is a regime challenging for all dimensionality reduction methods. Our work corroborates and explains previous observations in the literature that SDR can be more effective in detecting covariation patterns in data. These findings suggest that SDR should be preferred to IDR in real-world data analysis when detecting covariation is more important than preserving variation.