Theoretical Guarantees for Variational Inference with Fixed-Variance Mixture of Gaussians

Variational inference (VI) is a popular approach in Bayesian inference, that looks for the best approximation of the posterior distribution within a parametric family, minimizing a loss that is typically the (reverse) Kullback-Leibler (KL) divergence. Despite its empirical success, the theoretical properties of VI have only received attention recently, and mostly when the parametric family is the one of Gaussians. This work aims to contribute to the theoretical study of VI in the non-Gaussian case by investigating the setting of Mixture of Gaussians with fixed covariance and constant weights. In this view, VI over this specific family can be casted as the minimization of a Mollified relative entropy, i.e. the KL between the convolution (with respect to a Gaussian kernel) of an atomic measure supported on Diracs, and the target distribution. The support of the atomic measure corresponds to the localization of the Gaussian components. Hence, solving variational inference becomes equivalent to optimizing the positions of the Diracs (the particles), which can be done through gradient descent and takes the form of an interacting particle system. We study two sources of error of variational inference in this context when optimizing the mollified relative entropy. The first one is an optimization result, that is a descent lemma establishing that the algorithm decreases the objective at each iteration. The second one is an approximation error, that upper bounds the objective between an optimal finite mixture and the target distribution.

Central Limit Theorem for Bayesian Neural Network trained with Variational Inference

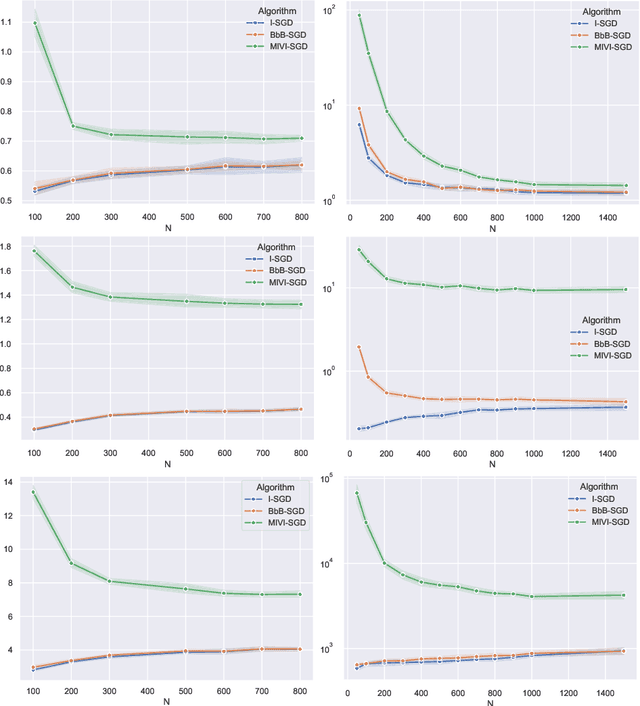
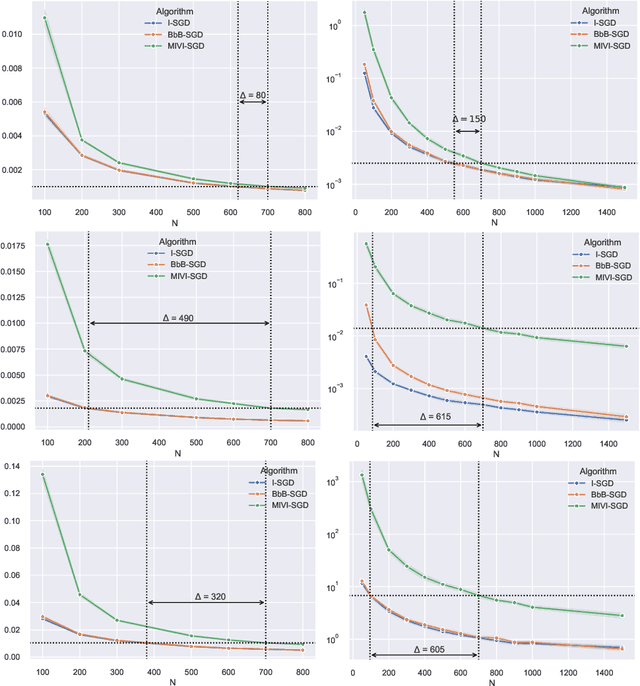
In this paper, we rigorously derive Central Limit Theorems (CLT) for Bayesian two-layerneural networks in the infinite-width limit and trained by variational inference on a regression task. The different networks are trained via different maximization schemes of the regularized evidence lower bound: (i) the idealized case with exact estimation of a multiple Gaussian integral from the reparametrization trick, (ii) a minibatch scheme using Monte Carlo sampling, commonly known as Bayes-by-Backprop, and (iii) a computationally cheaper algorithm named Minimal VI. The latter was recently introduced by leveraging the information obtained at the level of the mean-field limit. Laws of large numbers are already rigorously proven for the three schemes that admits the same asymptotic limit. By deriving CLT, this work shows that the idealized and Bayes-by-Backprop schemes have similar fluctuation behavior, that is different from the Minimal VI one. Numerical experiments then illustrate that the Minimal VI scheme is still more efficient, in spite of bigger variances, thanks to its important gain in computational complexity.

Variational inference, Mixture of Gaussians, Bayesian Machine Learning

Variational inference (VI) is a popular approach in Bayesian inference, that looks for the best approximation of the posterior distribution within a parametric family, minimizing a loss that is typically the (reverse) Kullback-Leibler (KL) divergence. Despite its empirical success, the theoretical properties of VI have only received attention recently, and mostly when the parametric family is the one of Gaussians. This work aims to contribute to the theoretical study of VI in the non-Gaussian case by investigating the setting of Mixture of Gaussians with fixed covariance and constant weights. In this view, VI over this specific family can be casted as the minimization of a Mollified relative entropy, i.e. the KL between the convolution (with respect to a Gaussian kernel) of an atomic measure supported on Diracs, and the target distribution. The support of the atomic measure corresponds to the localization of the Gaussian components. Hence, solving variational inference becomes equivalent to optimizing the positions of the Diracs (the particles), which can be done through gradient descent and takes the form of an interacting particle system. We study two sources of error of variational inference in this context when optimizing the mollified relative entropy. The first one is an optimization result, that is a descent lemma establishing that the algorithm decreases the objective at each iteration. The second one is an approximation error, that upper bounds the objective between an optimal finite mixture and the target distribution.

VITS : Variational Inference Thomson Sampling for contextual bandits

In this paper, we introduce and analyze a variant of the Thompson sampling (TS) algorithm for contextual bandits. At each round, traditional TS requires samples from the current posterior distribution, which is usually intractable. To circumvent this issue, approximate inference techniques can be used and provide samples with distribution close to the posteriors. However, current approximate techniques yield to either poor estimation (Laplace approximation) or can be computationally expensive (MCMC methods, Ensemble sampling...). In this paper, we propose a new algorithm, Varational Inference Thompson sampling VITS, based on Gaussian Variational Inference. This scheme provides powerful posterior approximations which are easy to sample from, and is computationally efficient, making it an ideal choice for TS. In addition, we show that VITS achieves a sub-linear regret bound of the same order in the dimension and number of round as traditional TS for linear contextual bandit. Finally, we demonstrate experimentally the effectiveness of VITS on both synthetic and real world datasets.

Law of Large Numbers for Bayesian two-layer Neural Network trained with Variational Inference

We provide a rigorous analysis of training by variational inference (VI) of Bayesian neural networks in the two-layer and infinite-width case. We consider a regression problem with a regularized evidence lower bound (ELBO) which is decomposed into the expected log-likelihood of the data and the Kullback-Leibler (KL) divergence between the a priori distribution and the variational posterior. With an appropriate weighting of the KL, we prove a law of large numbers for three different training schemes: (i) the idealized case with exact estimation of a multiple Gaussian integral from the reparametrization trick, (ii) a minibatch scheme using Monte Carlo sampling, commonly known as Bayes by Backprop, and (iii) a new and computationally cheaper algorithm which we introduce as Minimal VI. An important result is that all methods converge to the same mean-field limit. Finally, we illustrate our results numerically and discuss the need for the derivation of a central limit theorem.

Variational Inference of overparameterized Bayesian Neural Networks: a theoretical and empirical study

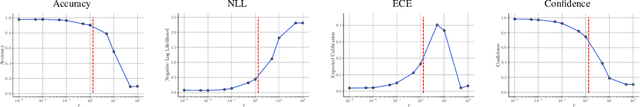
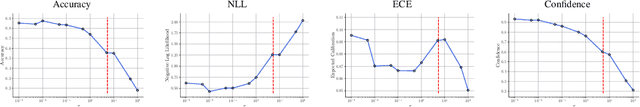
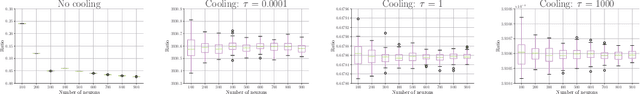
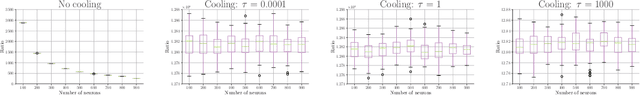
This paper studies the Variational Inference (VI) used for training Bayesian Neural Networks (BNN) in the overparameterized regime, i.e., when the number of neurons tends to infinity. More specifically, we consider overparameterized two-layer BNN and point out a critical issue in the mean-field VI training. This problem arises from the decomposition of the lower bound on the evidence (ELBO) into two terms: one corresponding to the likelihood function of the model and the second to the Kullback-Leibler (KL) divergence between the prior distribution and the variational posterior. In particular, we show both theoretically and empirically that there is a trade-off between these two terms in the overparameterized regime only when the KL is appropriately re-scaled with respect to the ratio between the the number of observations and neurons. We also illustrate our theoretical results with numerical experiments that highlight the critical choice of this ratio.