Combined Scheduling, Memory Allocation and Tensor Replacement for Minimizing Off-Chip Data Accesses of DNN Accelerators

Specialized hardware accelerators have been extensively used for Deep Neural Networks (DNNs) to provide power/performance benefits. These accelerators contain specialized hardware that supports DNN operators, and scratchpad memory for storing the tensor operands. Often, the size of the scratchpad is insufficient to store all the tensors needed for the computation, and additional data accesses are needed to move tensors back and forth from host memory during the computation with significant power/performance overhead. The volume of these additional data accesses depends on the operator schedule, and memory allocation (specific locations selected for the tensors in the scratchpad). We propose an optimization framework, named COSMA, for mapping DNNs to an accelerator that finds the optimal operator schedule, memory allocation and tensor replacement that minimizes the additional data accesses. COSMA provides an Integer Linear Programming (ILP) formulation to generate the optimal solution for mapping a DNN to the accelerator for a given scratchpad size. We demonstrate that, using an off-the-shelf ILP solver, COSMA obtains the optimal solution in seconds for a wide-range of state-of-the-art DNNs for different applications. Further, it out-performs existing methods by reducing on average 84% of the non-compulsory data accesses. We further propose a divide-and-conquer heuristic to scale up to certain complex DNNs generated by Neural Architecture Search, and this heuristic solution reduces on average 85% data accesses compared with other works.

Constrained Sampling and Counting: Universal Hashing Meets SAT Solving

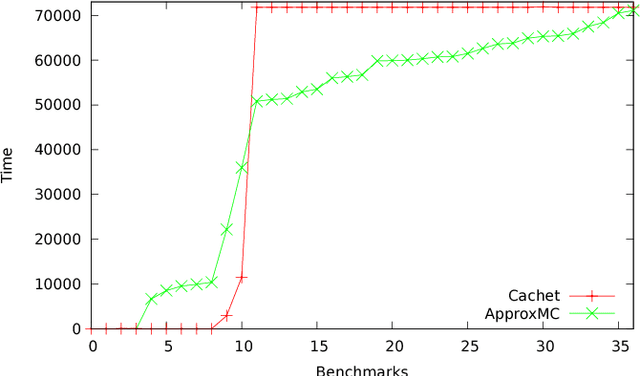
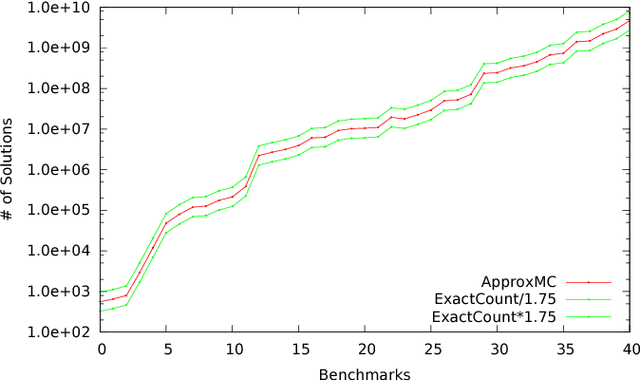
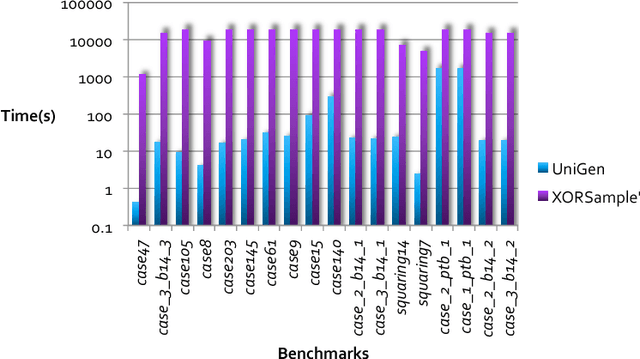
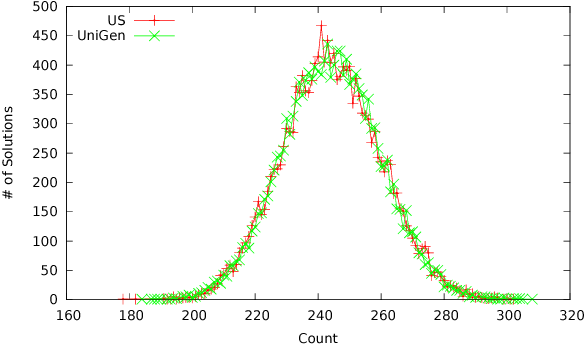
Constrained sampling and counting are two fundamental problems in artificial intelligence with a diverse range of applications, spanning probabilistic reasoning and planning to constrained-random verification. While the theory of these problems was thoroughly investigated in the 1980s, prior work either did not scale to industrial size instances or gave up correctness guarantees to achieve scalability. Recently, we proposed a novel approach that combines universal hashing and SAT solving and scales to formulas with hundreds of thousands of variables without giving up correctness guarantees. This paper provides an overview of the key ingredients of the approach and discusses challenges that need to be overcome to handle larger real-world instances.