Exploiting Invariance in Training Deep Neural Networks

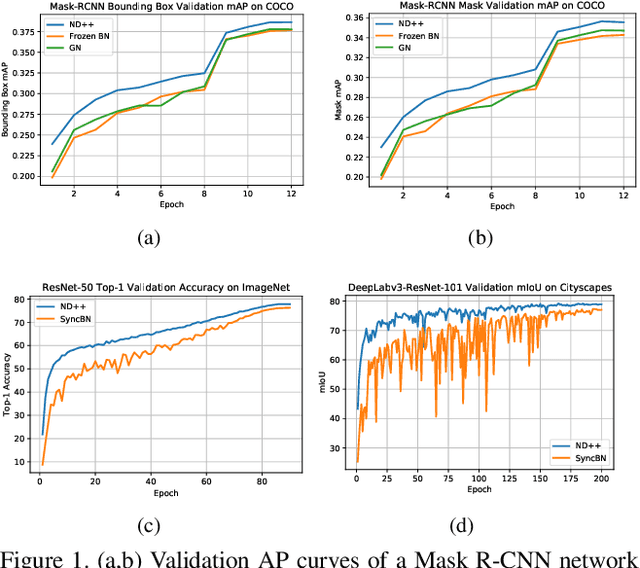
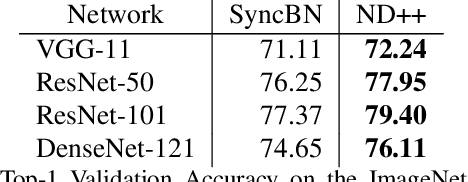
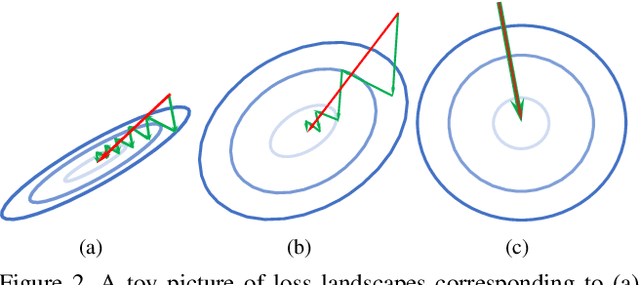
Inspired by two basic mechanisms in animal visual systems, we introduce a feature transform technique that imposes invariance properties in the training of deep neural networks. The resulting algorithm requires less parameter tuning, trains well with an initial learning rate 1.0, and easily generalizes to different tasks. We enforce scale invariance with local statistics in the data to align similar samples generated in diverse situations. To accelerate convergence, we enforce a GL(n)-invariance property with global statistics extracted from a batch that the gradient descent solution should remain invariant under basis change. Tested on ImageNet, MS COCO, and Cityscapes datasets, our proposed technique requires fewer iterations to train, surpasses all baselines by a large margin, seamlessly works on both small and large batch size training, and applies to different computer vision tasks of image classification, object detection, and semantic segmentation.

DaSGD: Squeezing SGD Parallelization Performance in Distributed Training Using Delayed Averaging

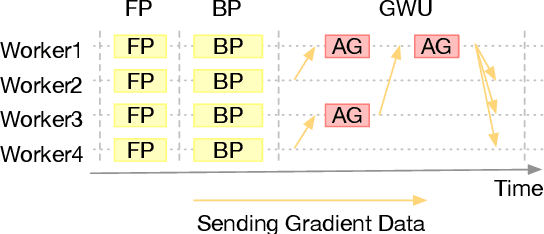
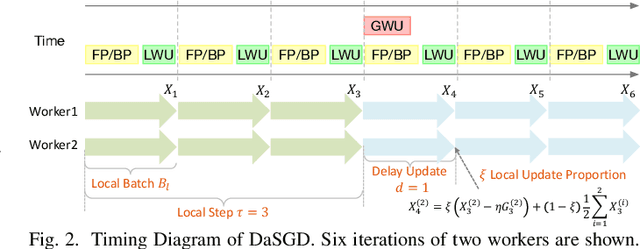
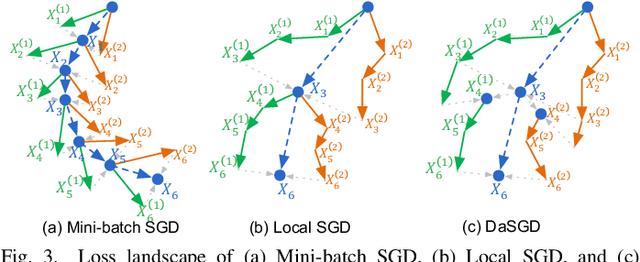
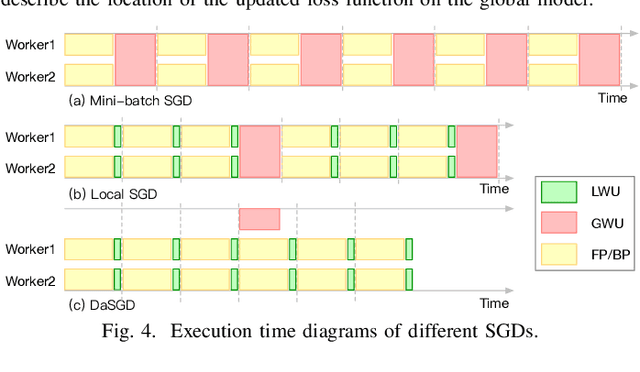
The state-of-the-art deep learning algorithms rely on distributed training systems to tackle the increasing sizes of models and training data sets. Minibatch stochastic gradient descent (SGD) algorithm requires workers to halt forward/back propagations, to wait for gradients aggregated from all workers, and to receive weight updates before the next batch of tasks. This synchronous execution model exposes the overheads of gradient/weight communication among a large number of workers in a distributed training system. We propose a new SGD algorithm, DaSGD (Local SGD with Delayed Averaging), which parallelizes SGD and forward/back propagations to hide 100% of the communication overhead. By adjusting the gradient update scheme, this algorithm uses hardware resources more efficiently and reduces the reliance on the low-latency and high-throughput inter-connects. The theoretical analysis and the experimental results show its convergence rate O(1/sqrt(K)), the same as SGD. The performance evaluation demonstrates it enables a linear performance scale-up with the cluster size.