Does Differentially Private Synthetic Data Lead to Synthetic Discoveries?

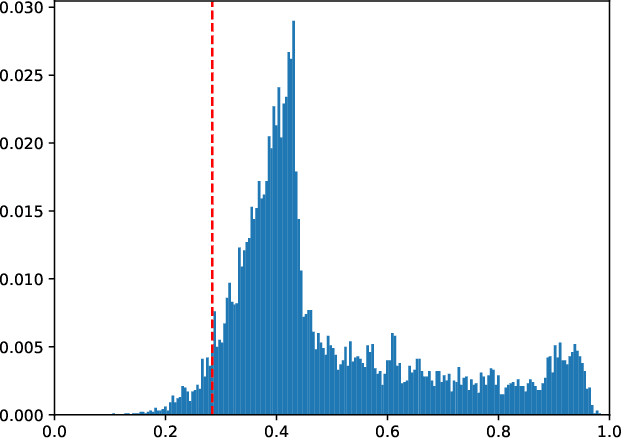
Background: Synthetic data has been proposed as a solution for sharing anonymized versions of sensitive biomedical datasets. Ideally, synthetic data should preserve the structure and statistical properties of the original data, while protecting the privacy of the individual subjects. Differential privacy (DP) is currently considered the gold standard approach for balancing this trade-off. Objectives: The aim of this study is to evaluate the Mann-Whitney U test on DP-synthetic biomedical data in terms of Type I and Type II errors, in order to establish whether statistical hypothesis testing performed on privacy preserving synthetic data is likely to lead to loss of test's validity or decreased power. Methods: We evaluate the Mann-Whitney U test on DP-synthetic data generated from real-world data, including a prostate cancer dataset (n=500) and a cardiovascular dataset (n=70 000), as well as on data drawn from two Gaussian distributions. Five different DP-synthetic data generation methods are evaluated, including two basic DP histogram release methods and MWEM, Private-PGM, and DP GAN algorithms. Conclusion: Most of the tested DP-synthetic data generation methods showed inflated Type I error, especially at privacy budget levels of $\epsilon\leq 1$. This result calls for caution when releasing and analyzing DP-synthetic data: low p-values may be obtained in statistical tests simply as a byproduct of the noise added to protect privacy. A DP smoothed histogram-based synthetic data generation method was shown to produce valid Type I error for all privacy levels tested but required a large original dataset size and a modest privacy budget ($\epsilon\geq 5$) in order to have reasonable Type II error levels.

A Link between Coding Theory and Cross-Validation with Applications

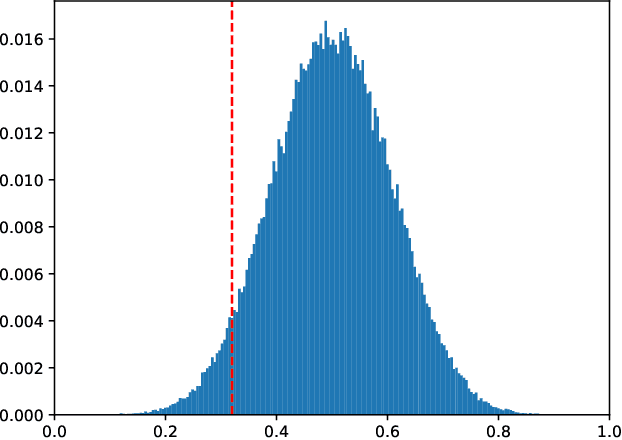
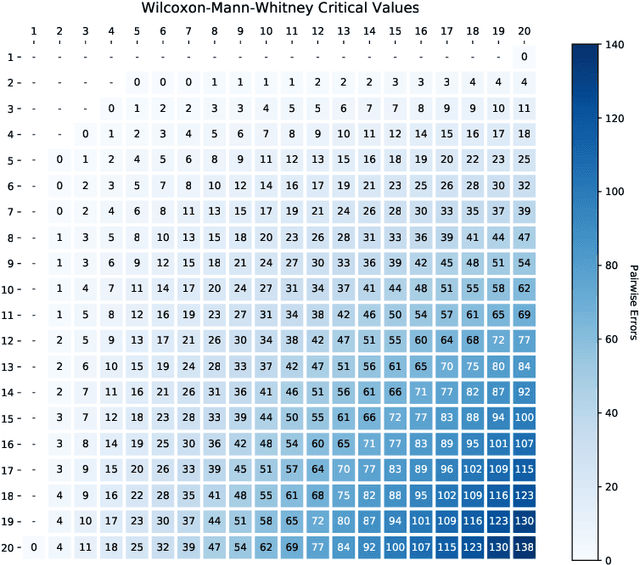
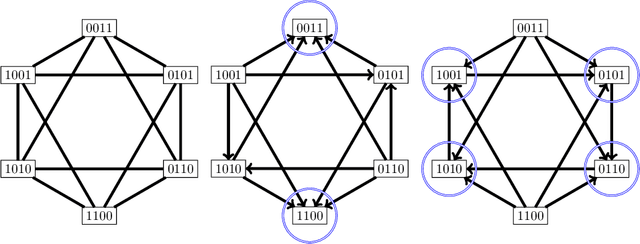
We study the combinatorics of cross-validation based AUC estimation under the null hypothesis that the binary class labels are exchangeable, that is, the data are randomly assigned into two classes given a fixed class proportion. In particular, we study how the estimators based on leave-pair-out cross-validation (LPOCV), in which every possible pair of data with different class labels is held out from the training set at a time, behave under the null without any prior assumptions of the learning algorithm or the data. It is shown that the maximal number of different fixed proportion label assignments on a sample of data, for which a learning algorithm can achieve zero LPOCV error, is the maximal size of a constant weight error correcting code, whose length is the sample size, weight is the number of data labeled with one, and the Hamming distance between code words is four. We then introduce the concept of a light constant weight code and show similar results for nonzero LPOCV errors. We also prove both upper and lower bounds on the maximal sizes of the light constant weight codes that are similar to the classical results for contant weight codes. These results pave the way towards the design of new LPOCV based statistical tests for the learning algorithms ability of distinguishing two classes from each other that are analogous to the classical Wilcoxon-Mann-Whitney U test for fixed functions. Behavior of some representative examples of learning algorithms and data are simulated in an experimental case study.

Long-Term Autonomy in Forest Environment using Self-Corrective SLAM

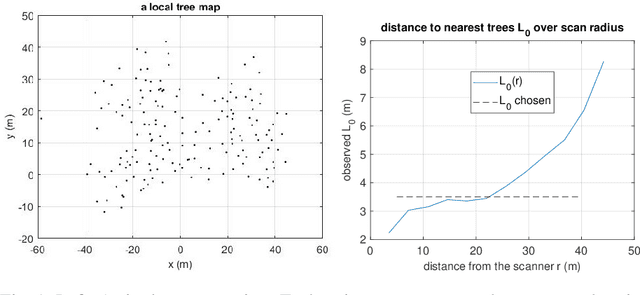
Vehicles with prolonged autonomous missions have to maintain environment awareness by simultaneous localization and mapping (SLAM). Closed loop correction is substituted by interpolation in rigid body transformation space in order to systematically reduce the accumulated error over different scales. The computation is divided to an edge computed lightweight SLAM and iterative corrections in the cloud environment. Tree locations in the forest environment are sent via a potentially limited communication bandwidths. Data from a real forest site is used in the verification of the proposed algorithm. The algorithm adds new iterative closest point (ICP) cases to the initial SLAM and measures the resulting map quality by the mean of the root mean squared error (RMSE) of individual tree clusters. Adding 4% more match cases yields the mean RMSE 0.15 m on a large site with 180 m odometric distance.