Statistical inference for quantum singular models

Deep learning has seen substantial achievements, with numerical and theoretical evidence suggesting that singularities of statistical models are considered a contributing factor to its performance. From this remarkable success of classical statistical models, it is naturally expected that quantum singular models will play a vital role in many quantum statistical tasks. However, while the theory of quantum statistical models in regular cases has been established, theoretical understanding of quantum singular models is still limited. To investigate the statistical properties of quantum singular models, we focus on two prominent tasks in quantum statistical inference: quantum state estimation and model selection. In particular, we base our study on classical singular learning theory and seek to extend it within the framework of Bayesian quantum state estimation. To this end, we define quantum generalization and training loss functions and give their asymptotic expansions through algebraic geometrical methods. The key idea of the proof is the introduction of a quantum analog of the likelihood function using classical shadows. Consequently, we construct an asymptotically unbiased estimator of the quantum generalization loss, the quantum widely applicable information criterion (QWAIC), as a computable model selection metric from given measurement outcomes.

Quantum Inception Score

Motivated by the great success of classical generative models in machine learning, enthusiastic exploration of their quantum version has recently started. To depart on this journey, it is important to develop a relevant metric to evaluate the quality of quantum generative models; in the classical case, one such examples is the inception score. In this paper, we propose the quantum inception score, which relates the quality to the classical capacity of the quantum channel that classifies a given dataset. We prove that, under this proposed measure, the quantum generative models provide better quality than their classical counterparts because of the presence of quantum coherence and entanglement. Finally, we harness the quantum fluctuation theorem to characterize the physical limitation of the quality of quantum generative models.

Quantum Noise-Induced Reservoir Computing

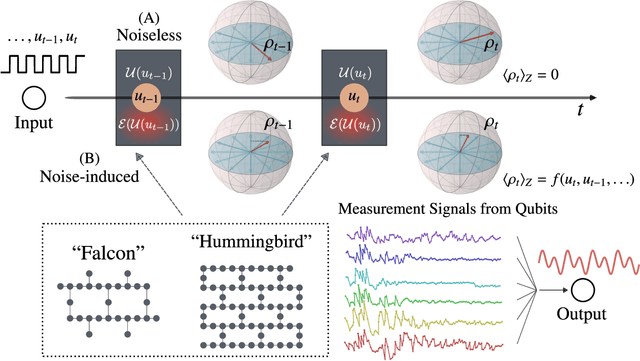
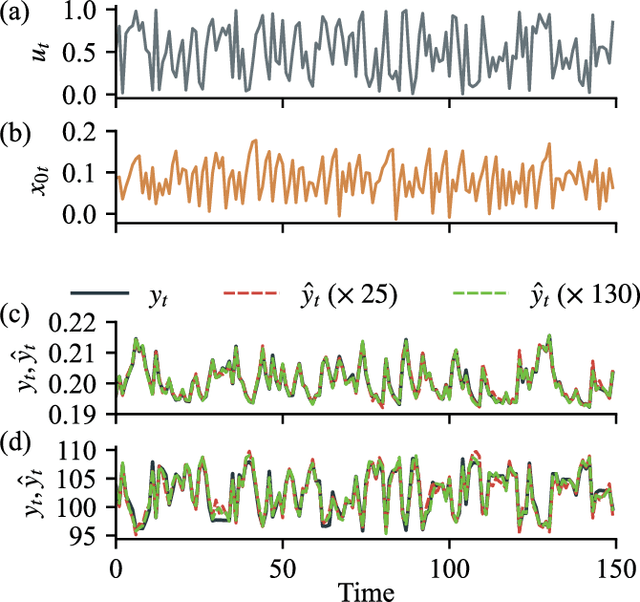
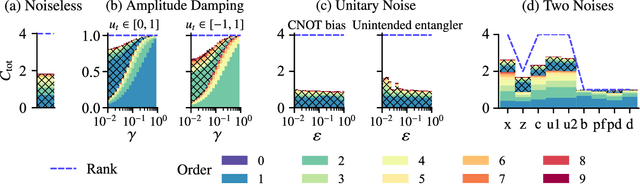
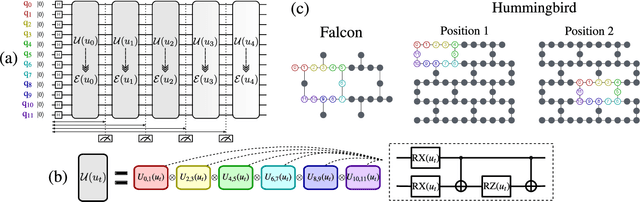
Quantum computing has been moving from a theoretical phase to practical one, presenting daunting challenges in implementing physical qubits, which are subjected to noises from the surrounding environment. These quantum noises are ubiquitous in quantum devices and generate adverse effects in the quantum computational model, leading to extensive research on their correction and mitigation techniques. But do these quantum noises always provide disadvantages? We tackle this issue by proposing a framework called quantum noise-induced reservoir computing and show that some abstract quantum noise models can induce useful information processing capabilities for temporal input data. We demonstrate this ability in several typical benchmarks and investigate the information processing capacity to clarify the framework's processing mechanism and memory profile. We verified our perspective by implementing the framework in a number of IBM quantum processors and obtained similar characteristic memory profiles with model analyses. As a surprising result, information processing capacity increased with quantum devices' higher noise levels and error rates. Our study opens up a novel path for diverting useful information from quantum computer noises into a more sophisticated information processor.

Overfitting in quantum machine learning and entangling dropout

The ultimate goal in machine learning is to construct a model function that has a generalization capability for unseen dataset, based on given training dataset. If the model function has too much expressibility power, then it may overfit to the training data and as a result lose the generalization capability. To avoid such overfitting issue, several techniques have been developed in the classical machine learning regime, and the dropout is one such effective method. This paper proposes a straightforward analogue of this technique in the quantum machine learning regime, the entangling dropout, meaning that some entangling gates in a given parametrized quantum circuit are randomly removed during the training process to reduce the expressibility of the circuit. Some simple case studies are given to show that this technique actually suppresses the overfitting.

Model-based approach for analyzing prevalence of nuclear cataracts in elderly residents

Recent epidemiological studies have hypothesized that the prevalence of cortical cataracts is closely related to ultraviolet radiation. However, the prevalence of nuclear cataracts is higher in elderly people in tropical areas than in temperate areas. The dominant factors inducing nuclear cataracts have been widely debated. In this study, the temperature increase in the lens due to exposure to ambient conditions was computationally quantified in subjects of 50-60 years of age in tropical and temperate areas, accounting for differences in thermoregulation. A thermoregulatory response model was extended to consider elderly people in tropical areas. The time course of lens temperature for different weather conditions in five cities in Asia was computed. The temperature was higher around the mid and posterior part of the lens, which coincides with the position of the nuclear cataract. The duration of higher temperatures in the lens varied, although the daily maximum temperatures were comparable. A strong correlation (adjusted R2 > 0.85) was observed between the prevalence of nuclear cataract and the computed cumulative thermal dose in the lens. We propose the use of a cumulative thermal dose to assess the prevalence of nuclear cataracts. Cumulative wet-bulb globe temperature, a new metric computed from weather data, would be useful for practical assessment in different cities.

Temporal Information Processing on Noisy Quantum Computers

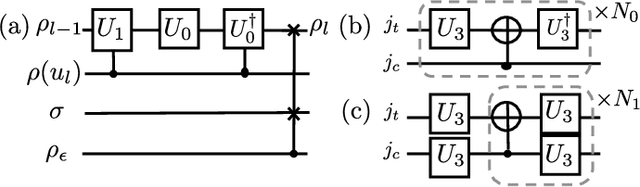
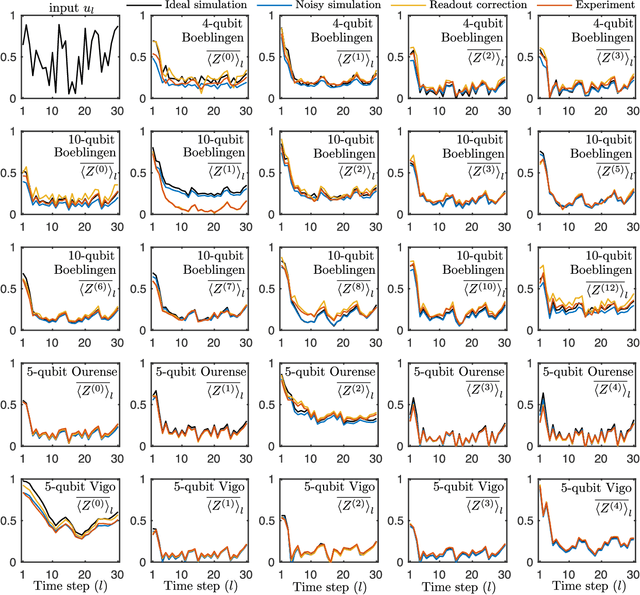
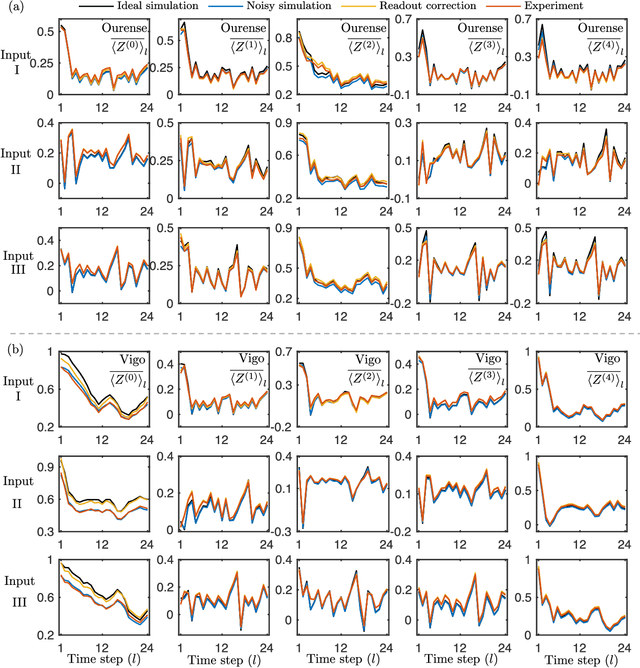
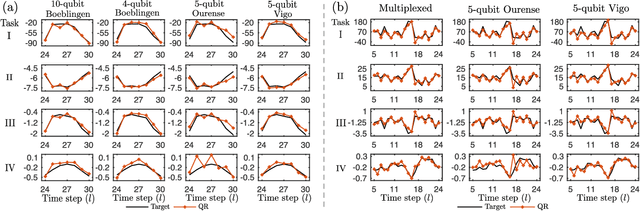
The combination of machine learning and quantum computing has emerged as a promising approach for addressing previously untenable problems. Reservoir computing is a state-of-the-art machine learning paradigm that utilizes nonlinear dynamical systems for temporal information processing, whose state-space dimension plays a key role in the performance. Here we propose a quantum reservoir system that harnesses complex dissipative quantum dynamics and the exponentially large quantum state-space. Our proposal is readily implementable on available noisy gate-model quantum processors and possesses universal computational power for approximating nonlinear short-term memory maps, important in applications such as neural modeling, speech recognition and natural language processing. We experimentally demonstrate on superconducting quantum computers that small and noisy quantum reservoirs can tackle high-order nonlinear temporal tasks. Our theoretical and experimental results pave the way for attractive temporal processing applications of near-term gate-model quantum computers of increasing fidelity but without quantum error correction, signifying the potential of these devices for wider applications beyond static classification and regression tasks in interdisciplinary areas.