Evaluating Unsupervised Denoising Requires Unsupervised Metrics

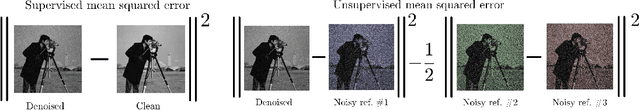
Unsupervised denoising is a crucial challenge in real-world imaging applications. Unsupervised deep-learning methods have demonstrated impressive performance on benchmarks based on synthetic noise. However, no metrics are available to evaluate these methods in an unsupervised fashion. This is highly problematic for the many practical applications where ground-truth clean images are not available. In this work, we propose two novel metrics: the unsupervised mean squared error (MSE) and the unsupervised peak signal-to-noise ratio (PSNR), which are computed using only noisy data. We provide a theoretical analysis of these metrics, showing that they are asymptotically consistent estimators of the supervised MSE and PSNR. Controlled numerical experiments with synthetic noise confirm that they provide accurate approximations in practice. We validate our approach on real-world data from two imaging modalities: videos in raw format and transmission electron microscopy. Our results demonstrate that the proposed metrics enable unsupervised evaluation of denoising methods based exclusively on noisy data.

Deep Probability Estimation

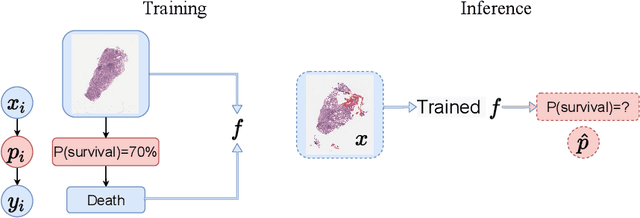
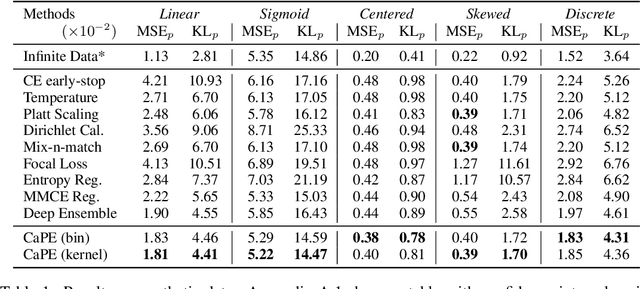
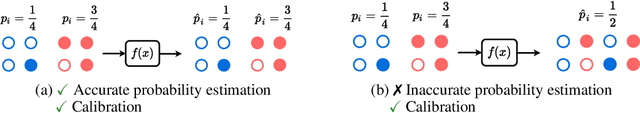
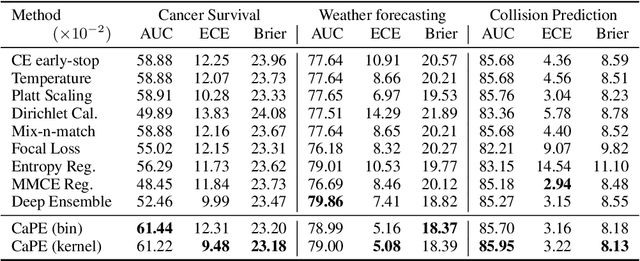
Reliable probability estimation is of crucial importance in many real-world applications where there is inherent uncertainty, such as weather forecasting, medical prognosis, or collision avoidance in autonomous vehicles. Probability-estimation models are trained on observed outcomes (e.g. whether it has rained or not, or whether a patient has died or not), because the ground-truth probabilities of the events of interest are typically unknown. The problem is therefore analogous to binary classification, with the important difference that the objective is to estimate probabilities rather than predicting the specific outcome. The goal of this work is to investigate probability estimation from high-dimensional data using deep neural networks. There exist several methods to improve the probabilities generated by these models but they mostly focus on classification problems where the probabilities are related to model uncertainty. In the case of problems with inherent uncertainty, it is challenging to evaluate performance without access to ground-truth probabilities. To address this, we build a synthetic dataset to study and compare different computable metrics. We evaluate existing methods on the synthetic data as well as on three real-world probability estimation tasks, all of which involve inherent uncertainty: precipitation forecasting from radar images, predicting cancer patient survival from histopathology images, and predicting car crashes from dashcam videos. Finally, we also propose a new method for probability estimation using neural networks, which modifies the training process to promote output probabilities that are consistent with empirical probabilities computed from the data. The method outperforms existing approaches on most metrics on the simulated as well as real-world data.

Correlation based Imaging for rotating satellites

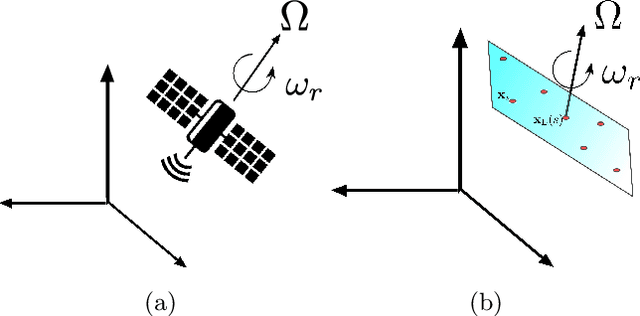
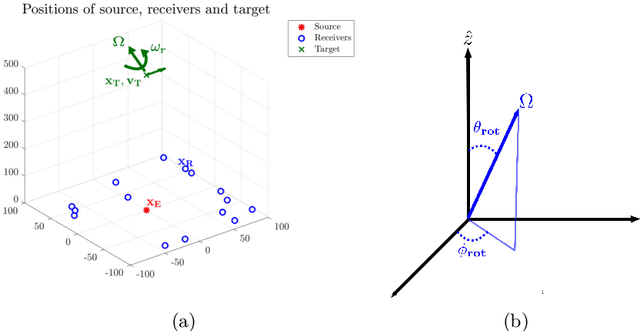
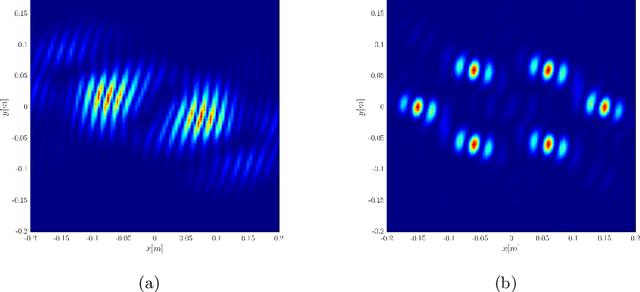
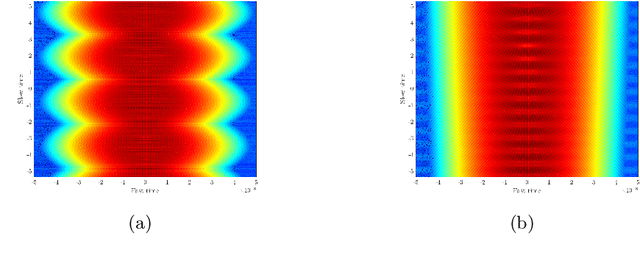
We consider imaging of fast moving small objects in space, such as low earth orbit satellites, which are also rotating around a fixed axis. The imaging system consists of ground based, asynchronous sources of radiation and several passive receivers above the dense atmosphere. We use the cross-correlation of the received signals to reduce distortions from ambient medium fluctuations. Imaging with correlations also has the advantage of not requiring any knowledge about the probing pulse and depends weakly on the emitter positions. We account for the target's orbital velocity by introducing the necessary Doppler compensation. To image a fast rotating object we also need to compensate for the rotation. We show that the rotation parameters can be extracted directly from the auto-correlation of the data before the formation of the image. We then investigate and analyze an imaging method that relies on backpropagating the cross-correlation data structure to two points rather than one, thus forming an interference matrix. The proposed imaging method consists of estimating the reflectivity as the top eigenvector of the migrated cross-correlation data interference matrix. We call this the rank-1 image and show that it provides superior image resolution compared to the usual single-point migration scheme for fast moving and rotating objects. Moreover, we observe a significant improvement in resolution due to the rotation leading to a diffraction limited resolution. We carry out a theoretical analysis that illustrates the role of the two point migration method as well as that of the inverse aperture and rotation in improving resolution. Extensive numerical simulations support the theoretical results.