Boolean Observation Games

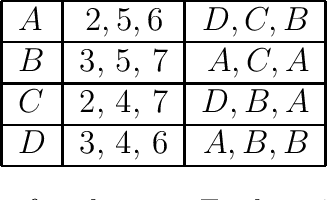
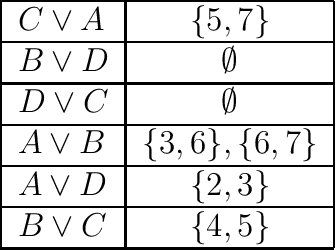
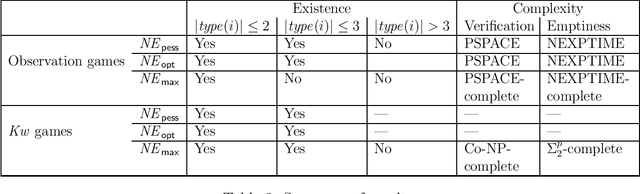
We introduce Boolean Observation Games, a subclass of multi-player finite strategic games with incomplete information and qualitative objectives. In Boolean observation games, each player is associated with a finite set of propositional variables of which only it can observe the value, and it controls whether and to whom it can reveal that value. It does not control the given, fixed, value of variables. Boolean observation games are a generalization of Boolean games, a well-studied subclass of strategic games but with complete information, and wherein each player controls the value of its variables. In Boolean observation games player goals describe multi-agent knowledge of variables. As in classical strategic games, players choose their strategies simultaneously and therefore observation games capture aspects of both imperfect and incomplete information. They require reasoning about sets of outcomes given sets of indistinguishable valuations of variables. What a Nash equilibrium is, depends on an outcome relation between such sets. We present various outcome relations, including a qualitative variant of ex-post equilibrium. We identify conditions under which, given an outcome relation, Nash equilibria are guaranteed to exist. We also study the complexity of checking for the existence of Nash equilibria and of verifying if a strategy profile is a Nash equilibrium. We further study the subclass of Boolean observation games with `knowing whether' goal formulas, for which the satisfaction does not depend on the value of variables. We show that each such Boolean observation game corresponds to a Boolean game and vice versa, by a different correspondence, and that both correspondences are precise in terms of existence of Nash equilibria.

Everyone Knows that Everyone Knows: Gossip Protocols for Super Experts

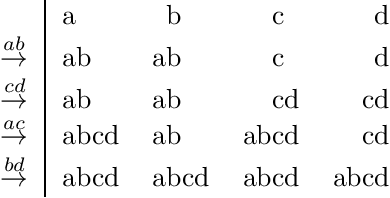
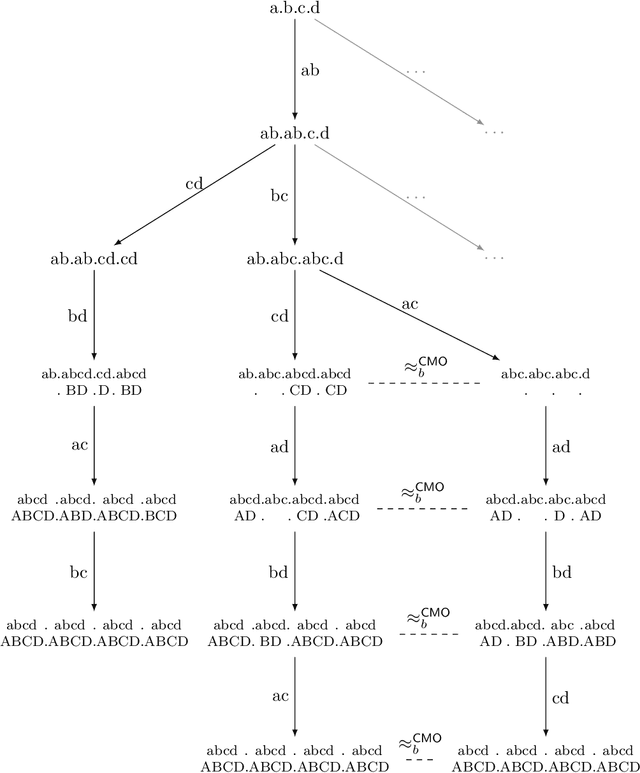
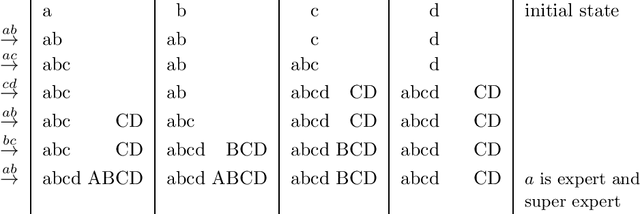
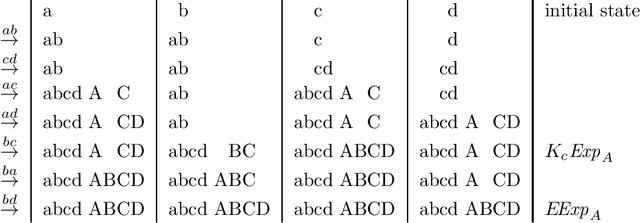
A gossip protocol is a procedure for sharing secrets in a network. The basic action in a gossip protocol is a telephone call wherein the calling agents exchange all the secrets they know. An agent who knows all secrets is an expert. The usual termination condition is that all agents are experts. Instead, we explore protocols wherein the termination condition is that all agents know that all agents are experts. We call such agents super experts. Additionally, we model that agents who are super experts do not make and do not answer calls. Such agents are called engaged agents. We also model that such gossip protocols are common knowledge among the agents. We investigate conditions under which protocols terminate, both in the synchronous case, where there is a global clock, and in the asynchronous case, where there is not. We show that a commonly known protocol with engaged agents may terminate faster than the same protocol without engaged agents.

Quantifying Notes Revisited

In this survey we review several dynamic epistemic logics with modalities representing quantification over information change. Of such logics we present typical axioms involving the relation between knowledge or belief and informative action, their relative expressivity, directions for applications, and what is known on the decidability and complexity of model checking and satisfiability. We focus on open problems and new directions for research.

A Logic for Global and Local Announcements

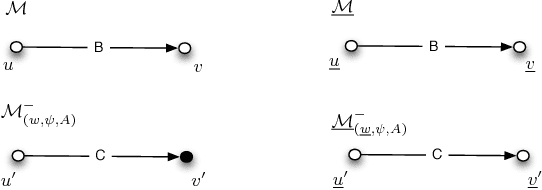
In this paper we introduce {\em global and local announcement logic} (GLAL), a dynamic epistemic logic with two distinct announcement operators -- $[\phi]^+_A$ and $[\phi]^-_A$ indexed to a subset $A$ of the set $Ag$ of all agents -- for global and local announcements respectively. The boundary case $[\phi]^+_{Ag}$ corresponds to the public announcement of $\phi$, as known from the literature. Unlike standard public announcements, which are {\em model transformers}, the global and local announcements are {\em pointed model transformers}. In particular, the update induced by the announcement may be different in different states of the model. Therefore, the resulting computations are trees of models, rather than the typical sequences. A consequence of our semantics is that modally bisimilar states may be distinguished in our logic. Then, we provide a stronger notion of bisimilarity and we show that it preserves modal equivalence in GLAL. Additionally, we show that GLAL is strictly more expressive than public announcement logic with common knowledge. We prove a wide range of validities for GLAL involving the interaction between dynamics and knowledge, and show that the satisfiability problem for GLAL is decidable. We illustrate the formal machinery by means of detailed epistemic scenarios.

Cheryl's Birthday

We present four logic puzzles and after that their solutions. Joseph Yeo designed 'Cheryl's Birthday'. Mike Hartley came up with a novel solution for 'One Hundred Prisoners and a Light Bulb'. Jonathan Welton designed 'A Blind Guess' and 'Abby's Birthday'. Hans van Ditmarsch and Barteld Kooi authored the puzzlebook 'One Hundred Prisoners and a Light Bulb' that contains other knowledge puzzles, and that can also be found on the webpage http://personal.us.es/hvd/lightbulb.html dedicated to the book.

Asynchronous Announcements

We propose a logic of asynchronous announcements, where truthful announcements are publicly sent but individually received by agents. Additional to epistemic modalities, the logic therefore contains two types of dynamic modalities, for sending messages and for receiving messages. The semantics defines truth relative to the current state of reception of messages for all agents. This means that knowledge need not be truthful, because some messages may not have been received by the knowing agent. Messages that are announcements may also result in partial synchronization, namely when an agent learns from receiving an announcement that other announcements must already have been received by other agents. We give detailed examples of the semantics, and prove several semantic results, including that: after an announcement an agent knows that a proposition is true, if and only if on condition of the truth of that announcement, the agent knows that after that announcement and after any number of other agents also receiving it, the proposition is true. We show that on multi-agent epistemic models, each formula in asynchronous announcement logic is equivalent to a formula in epistemic logic.

True Lies

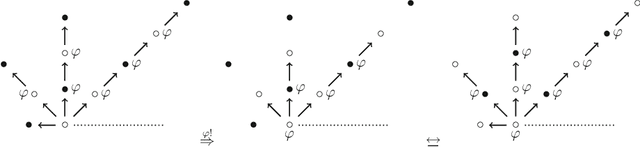
A true lie is a lie that becomes true when announced. In a logic of announcements, where the announcing agent is not modelled, a true lie is a formula (that is false and) that becomes true when announced. We investigate true lies and other types of interaction between announced formulas, their preconditions and their postconditions, in the setting of Gerbrandy's logic of believed announcements, wherein agents may have or obtain incorrect beliefs. Our results are on the satisfiability and validity of instantiations of these semantically defined categories, on iterated announcements, including arbitrarily often iterated announcements, and on syntactic characterization. We close with results for iterated announcements in the logic of knowledge (instead of belief), and for lying as private announcements (instead of public announcements) to different agents. Detailed examples illustrate our lying concepts.

Bisimulation and expressivity for conditional belief, degrees of belief, and safe belief

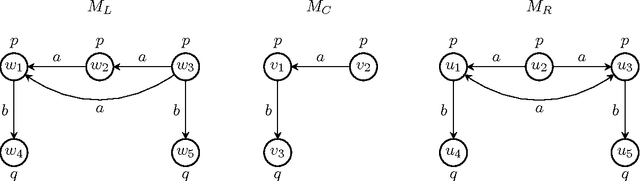
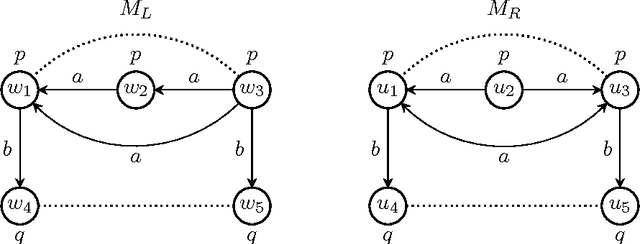
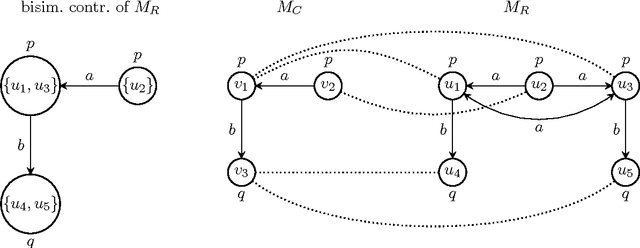
Plausibility models are Kripke models that agents use to reason about knowledge and belief, both of themselves and of each other. Such models are used to interpret the notions of conditional belief, degrees of belief, and safe belief. The logic of conditional belief contains that modality and also the knowledge modality, and similarly for the logic of degrees of belief and the logic of safe belief. With respect to these logics, plausibility models may contain too much information. A proper notion of bisimulation is required that characterises them. We define that notion of bisimulation and prove the required characterisations: on the class of image-finite and preimage-finite models (with respect to the plausibility relation), two pointed Kripke models are modally equivalent in either of the three logics, if and only if they are bisimilar. As a result, the information content of such a model can be similarly expressed in the logic of conditional belief, or the logic of degrees of belief, or that of safe belief. This, we found a surprising result. Still, that does not mean that the logics are equally expressive: the logics of conditional and degrees of belief are incomparable, the logics of degrees of belief and safe belief are incomparable, while the logic of safe belief is more expressive than the logic of conditional belief. In view of the result on bisimulation characterisation, this is an equally surprising result. We hope our insights may contribute to the growing community of formal epistemology and on the relation between qualitative and quantitative modelling.

An Introduction to Logics of Knowledge and Belief

This chapter provides an introduction to some basic concepts of epistemic logic, basic formal languages, their semantics, and proof systems. It also contains an overview of the handbook, and a brief history of epistemic logic and pointers to the literature.

Refinement Modal Logic

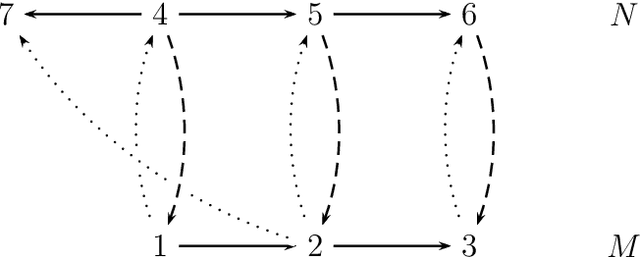
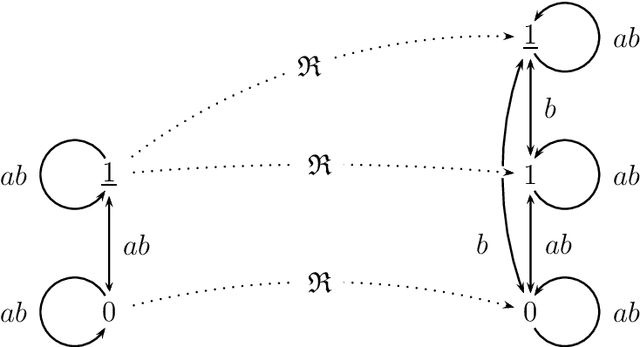
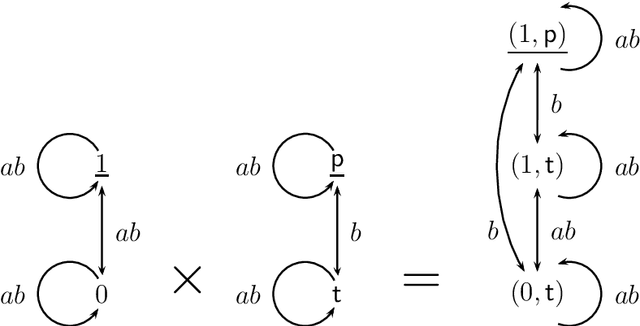
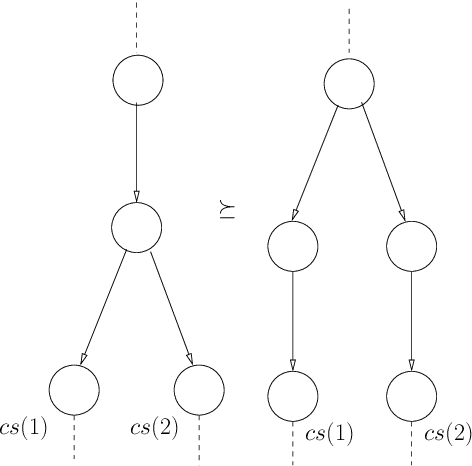
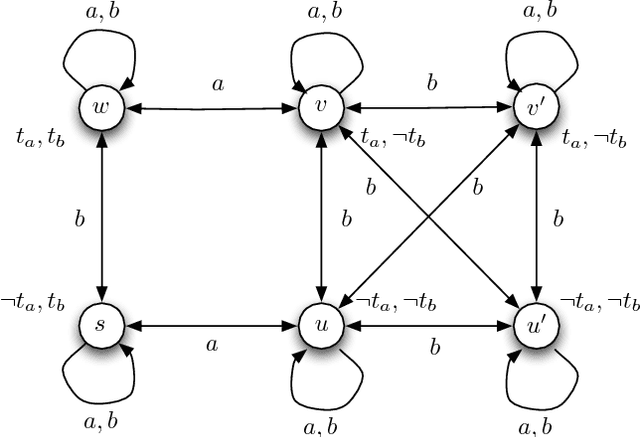
In this paper we present {\em refinement modal logic}. A refinement is like a bisimulation, except that from the three relational requirements only `atoms' and `back' need to be satisfied. Our logic contains a new operator 'all' in addition to the standard modalities 'box' for each agent. The operator 'all' acts as a quantifier over the set of all refinements of a given model. As a variation on a bisimulation quantifier, this refinement operator or refinement quantifier 'all' can be seen as quantifying over a variable not occurring in the formula bound by it. The logic combines the simplicity of multi-agent modal logic with some powers of monadic second-order quantification. We present a sound and complete axiomatization of multi-agent refinement modal logic. We also present an extension of the logic to the modal mu-calculus, and an axiomatization for the single-agent version of this logic. Examples and applications are also discussed: to software verification and design (the set of agents can also be seen as a set of actions), and to dynamic epistemic logic. We further give detailed results on the complexity of satisfiability, and on succinctness.