Efficient and Universal Neural-Network Decoder for Stabilizer-Based Quantum Error Correction

Quantum error correction is crucial for large-scale quantum computing, but the absence of efficient decoders for new codes like quantum low-density parity-check (QLDPC) codes has hindered progress. Here we introduce a universal decoder based on linear attention sequence modeling and graph neural network that operates directly on any stabilizer code's graph structure. Our numerical experiments demonstrate that this decoder outperforms specialized algorithms in both accuracy and speed across diverse stabilizer codes, including surface codes, color codes, and QLDPC codes. The decoder maintains linear time scaling with syndrome measurements and requires no structural modifications between different codes. For the Bivariate Bicycle code with distance 12, our approach achieves a 39.4% lower logical error rate than previous best decoders while requiring only ~1% of the decoding time. These results provide a practical, universal solution for quantum error correction, eliminating the need for code-specific decoders.

Experimental Quantum Generative Adversarial Networks for Image Generation

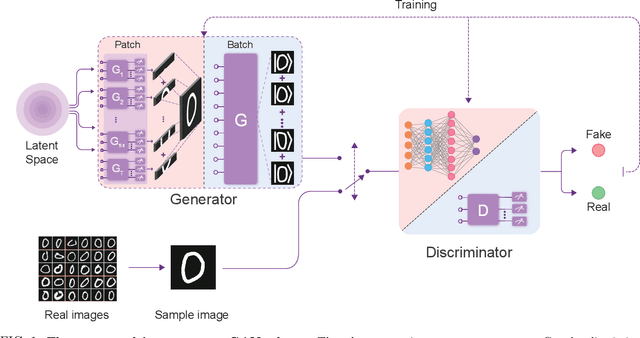
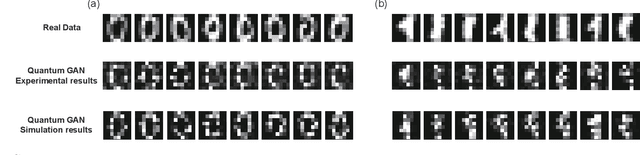
Quantum machine learning is expected to be one of the first practical applications of near-term quantum devices. Pioneer theoretical works suggest that quantum generative adversarial networks (GANs) may exhibit a potential exponential advantage over classical GANs, thus attracting widespread attention. However, it remains elusive whether quantum GANs implemented on near-term quantum devices can actually solve real-world learning tasks. Here, we devise a flexible quantum GAN scheme to narrow this knowledge gap, which could accomplish image generation with arbitrarily high-dimensional features, and could also take advantage of quantum superposition to train multiple examples in parallel. For the first time, we experimentally achieve the learning and generation of real-world hand-written digit images on a superconducting quantum processor. Moreover, we utilize a gray-scale bar dataset to exhibit the competitive performance between quantum GANs and the classical GANs based on multilayer perceptron and convolutional neural network architectures, respectively, benchmarked by the Fr\'echet Distance score. Our work provides guidance for developing advanced quantum generative models on near-term quantum devices and opens up an avenue for exploring quantum advantages in various GAN-related learning tasks.

Demonstration of Topological Data Analysis on a Quantum Processor

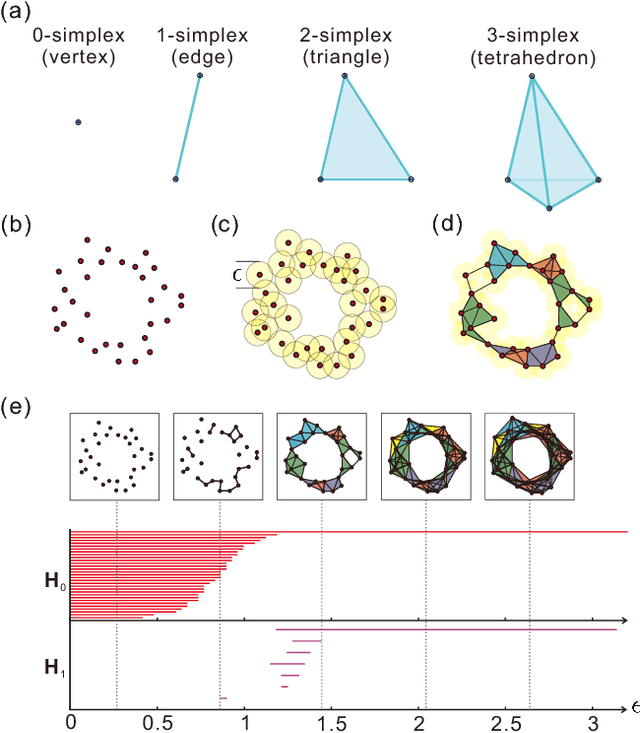
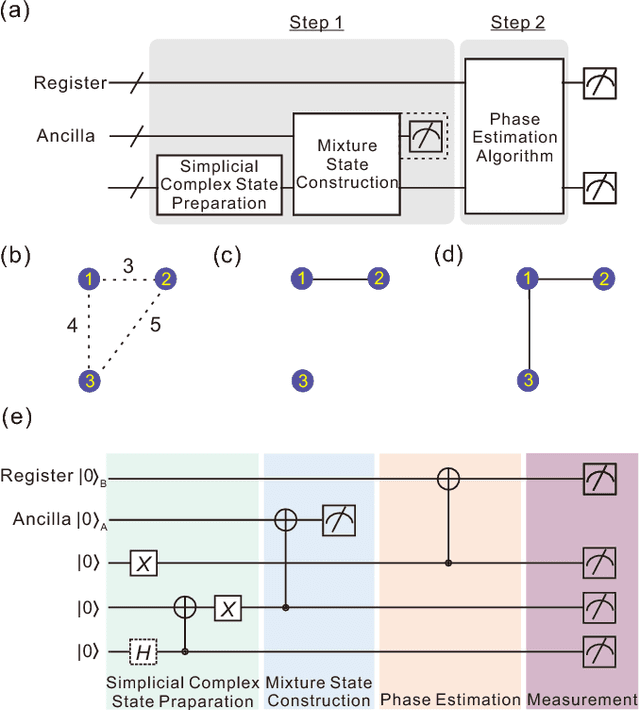
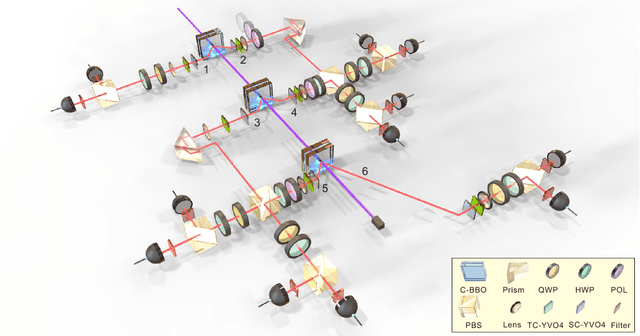
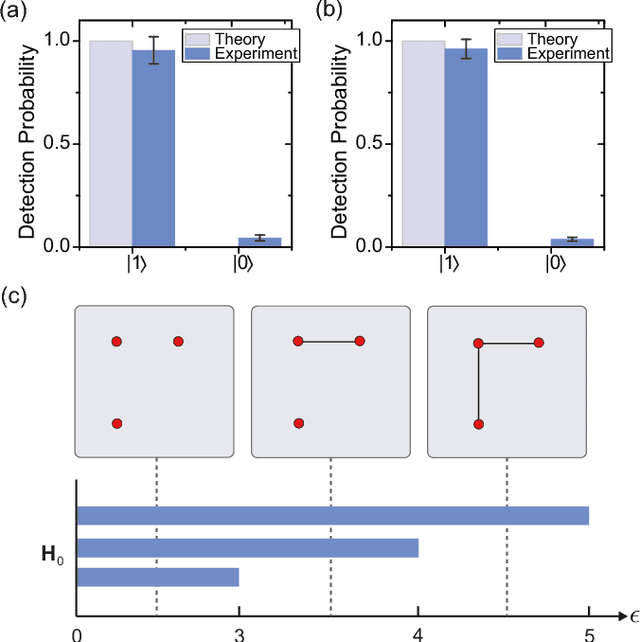
Topological data analysis offers a robust way to extract useful information from noisy, unstructured data by identifying its underlying structure. Recently, an efficient quantum algorithm was proposed [Lloyd, Garnerone, Zanardi, Nat. Commun. 7, 10138 (2016)] for calculating Betti numbers of data points -- topological features that count the number of topological holes of various dimensions in a scatterplot. Here, we implement a proof-of-principle demonstration of this quantum algorithm by employing a six-photon quantum processor to successfully analyze the topological features of Betti numbers of a network including three data points, providing new insights into data analysis in the era of quantum computing.