NeurTV: Total Variation on the Neural Domain

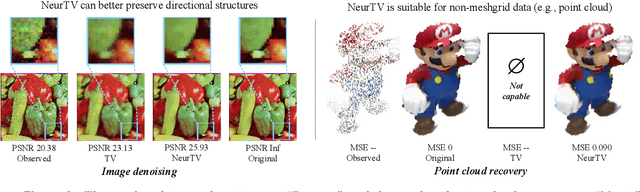
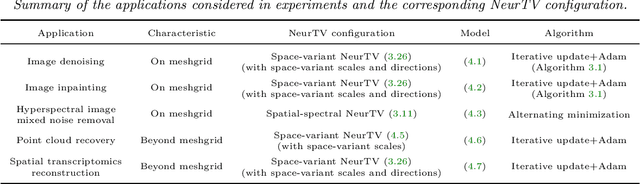
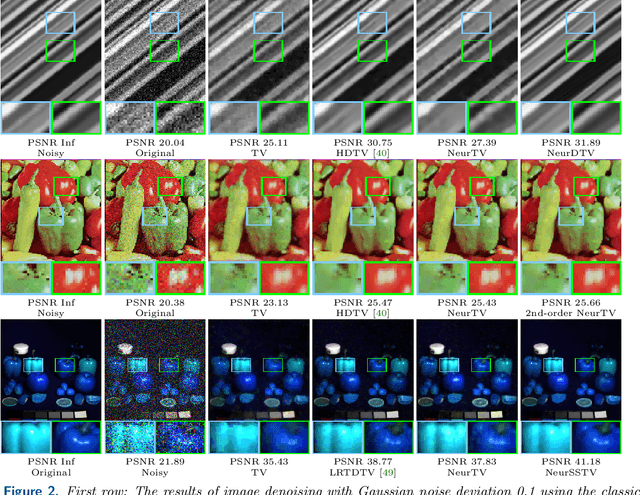
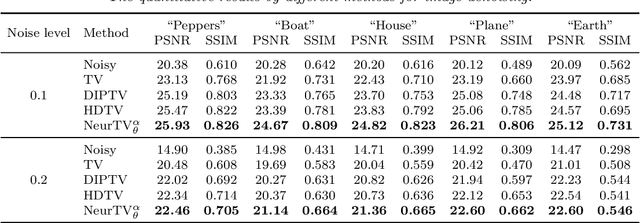
Recently, we have witnessed the success of total variation (TV) for many imaging applications. However, traditional TV is defined on the original pixel domain, which limits its potential. In this work, we suggest a new TV regularization defined on the neural domain. Concretely, the discrete data is continuously and implicitly represented by a deep neural network (DNN), and we use the derivatives of DNN outputs w.r.t. input coordinates to capture local correlations of data. As compared with classical TV on the original domain, the proposed TV on the neural domain (termed NeurTV) enjoys two advantages. First, NeurTV is not limited to meshgrid but is suitable for both meshgrid and non-meshgrid data. Second, NeurTV can more exactly capture local correlations across data for any direction and any order of derivatives attributed to the implicit and continuous nature of neural domain. We theoretically reinterpret NeurTV under the variational approximation framework, which allows us to build the connection between classical TV and NeurTV and inspires us to develop variants (e.g., NeurTV with arbitrary resolution and space-variant NeurTV). Extensive numerical experiments with meshgrid data (e.g., color and hyperspectral images) and non-meshgrid data (e.g., point clouds and spatial transcriptomics) showcase the effectiveness of the proposed methods.

Revisiting Nonlocal Self-Similarity from Continuous Representation

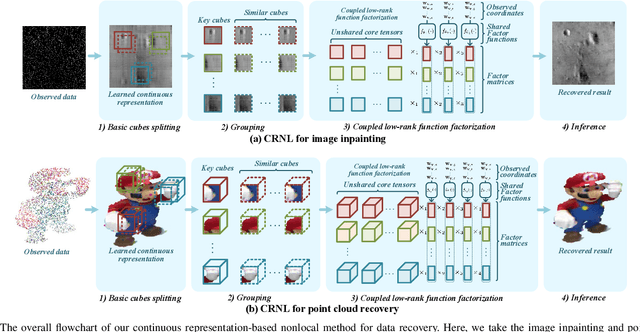
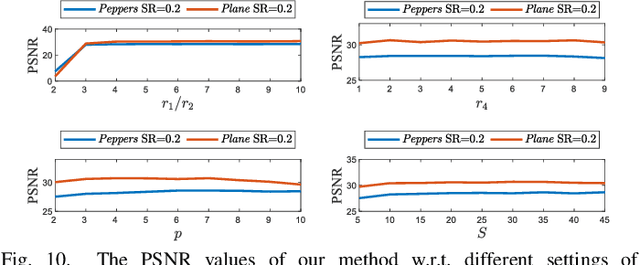
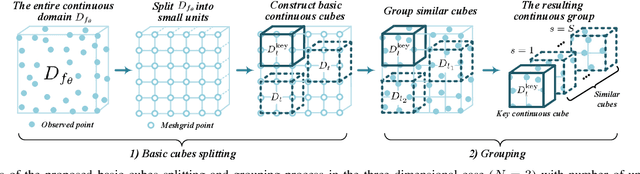
Nonlocal self-similarity (NSS) is an important prior that has been successfully applied in multi-dimensional data processing tasks, e.g., image and video recovery. However, existing NSS-based methods are solely suitable for meshgrid data such as images and videos, but are not suitable for emerging off-meshgrid data, e.g., point cloud and climate data. In this work, we revisit the NSS from the continuous representation perspective and propose a novel Continuous Representation-based NonLocal method (termed as CRNL), which has two innovative features as compared with classical nonlocal methods. First, based on the continuous representation, our CRNL unifies the measure of self-similarity for on-meshgrid and off-meshgrid data and thus is naturally suitable for both of them. Second, the nonlocal continuous groups can be more compactly and efficiently represented by the coupled low-rank function factorization, which simultaneously exploits the similarity within each group and across different groups, while classical nonlocal methods neglect the similarity across groups. This elaborately designed coupled mechanism allows our method to enjoy favorable performance over conventional NSS methods in terms of both effectiveness and efficiency. Extensive multi-dimensional data processing experiments on-meshgrid (e.g., image inpainting and image denoising) and off-meshgrid (e.g., climate data prediction and point cloud recovery) validate the versatility, effectiveness, and efficiency of our CRNL as compared with state-of-the-art methods.

H2TF for Hyperspectral Image Denoising: Where Hierarchical Nonlinear Transform Meets Hierarchical Matrix Factorization

Recently, tensor singular value decomposition (t-SVD) has emerged as a promising tool for hyperspectral image (HSI) processing. In the t-SVD, there are two key building blocks: (i) the low-rank enhanced transform and (ii) the accompanying low-rank characterization of transformed frontal slices. Previous t-SVD methods mainly focus on the developments of (i), while neglecting the other important aspect, i.e., the exact characterization of transformed frontal slices. In this letter, we exploit the potentiality in both building blocks by leveraging the \underline{\bf H}ierarchical nonlinear transform and the \underline{\bf H}ierarchical matrix factorization to establish a new \underline{\bf T}ensor \underline{\bf F}actorization (termed as H2TF). Compared to shallow counter partners, e.g., low-rank matrix factorization or its convex surrogates, H2TF can better capture complex structures of transformed frontal slices due to its hierarchical modeling abilities. We then suggest the H2TF-based HSI denoising model and develop an alternating direction method of multipliers-based algorithm to address the resultant model. Extensive experiments validate the superiority of our method over state-of-the-art HSI denoising methods.

S2S-WTV: Seismic Data Noise Attenuation Using Weighted Total Variation Regularized Self-Supervised Learning

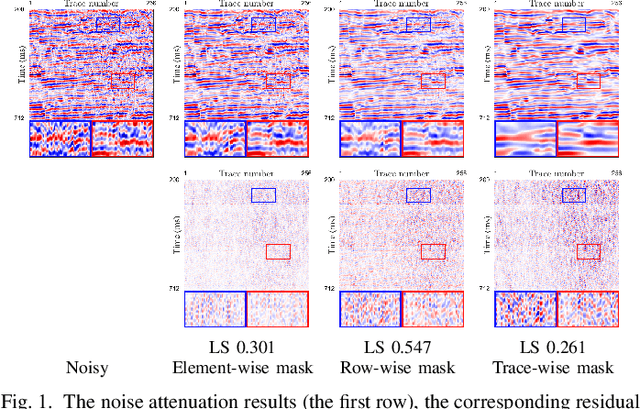
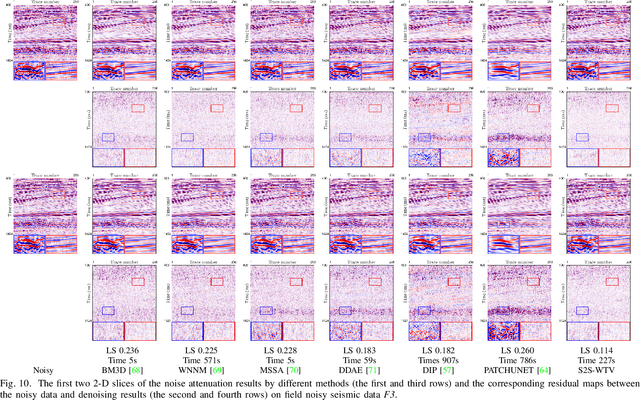
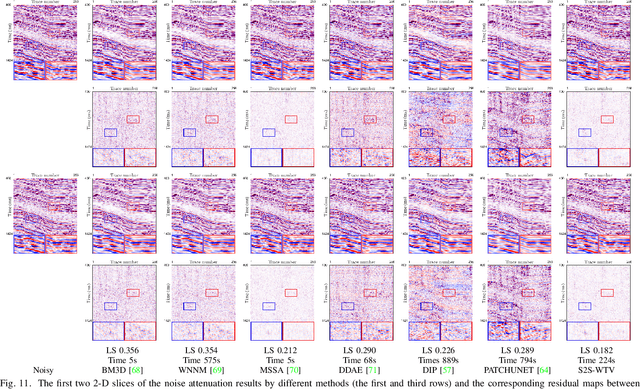
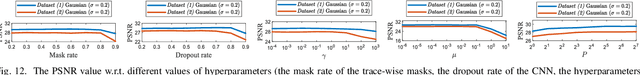
Seismic data often undergoes severe noise due to environmental factors, which seriously affects subsequent applications. Traditional hand-crafted denoisers such as filters and regularizations utilize interpretable domain knowledge to design generalizable denoising techniques, while their representation capacities may be inferior to deep learning denoisers, which can learn complex and representative denoising mappings from abundant training pairs. However, due to the scarcity of high-quality training pairs, deep learning denoisers may sustain some generalization issues over various scenarios. In this work, we propose a self-supervised method that combines the capacities of deep denoiser and the generalization abilities of hand-crafted regularization for seismic data random noise attenuation. Specifically, we leverage the Self2Self (S2S) learning framework with a trace-wise masking strategy for seismic data denoising by solely using the observed noisy data. Parallelly, we suggest the weighted total variation (WTV) to further capture the horizontal local smooth structure of seismic data. Our method, dubbed as S2S-WTV, enjoys both high representation abilities brought from the self-supervised deep network and good generalization abilities of the hand-crafted WTV regularizer and the self-supervised nature. Therefore, our method can more effectively and stably remove the random noise and preserve the details and edges of the clean signal. To tackle the S2S-WTV optimization model, we introduce an alternating direction multiplier method (ADMM)-based algorithm. Extensive experiments on synthetic and field noisy seismic data demonstrate the effectiveness of our method as compared with state-of-the-art traditional and deep learning-based seismic data denoising methods.

Low-Rank Tensor Function Representation for Multi-Dimensional Data Recovery

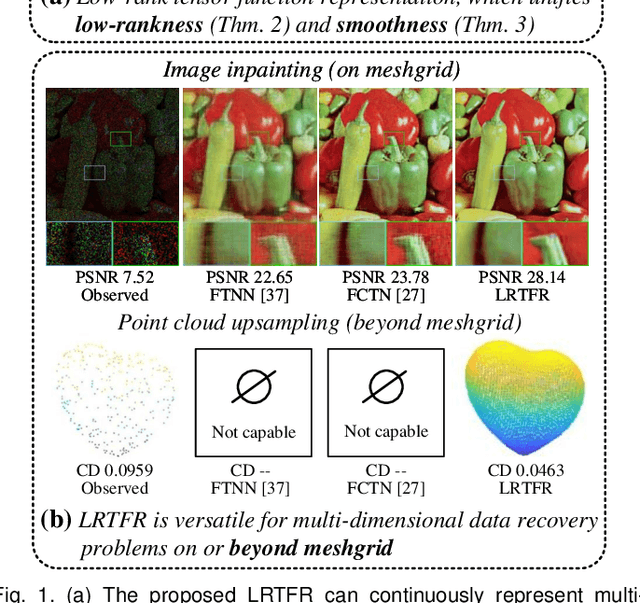
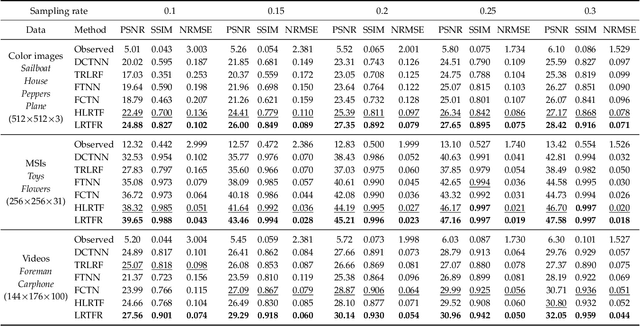
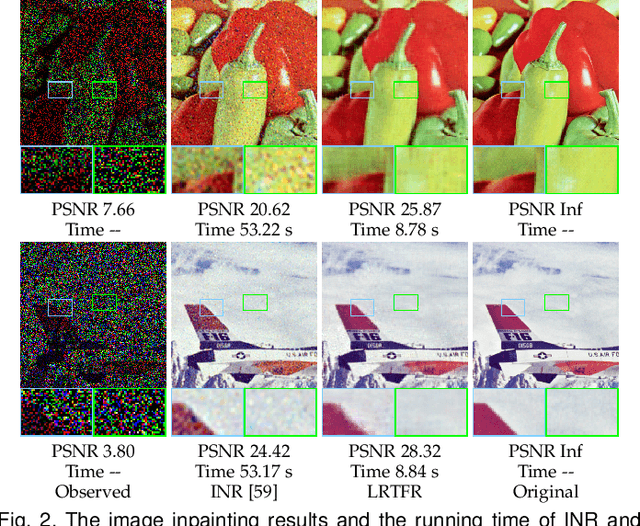
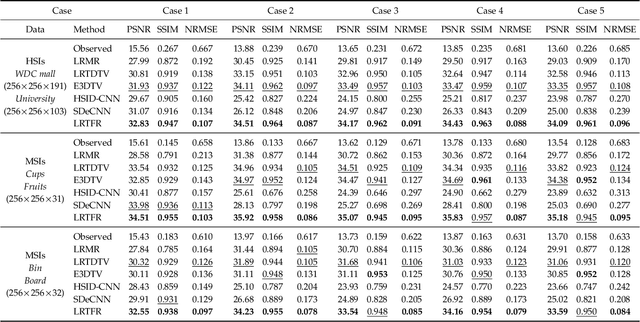
Since higher-order tensors are naturally suitable for representing multi-dimensional data in real-world, e.g., color images and videos, low-rank tensor representation has become one of the emerging areas in machine learning and computer vision. However, classical low-rank tensor representations can only represent data on finite meshgrid due to their intrinsical discrete nature, which hinders their potential applicability in many scenarios beyond meshgrid. To break this barrier, we propose a low-rank tensor function representation (LRTFR), which can continuously represent data beyond meshgrid with infinite resolution. Specifically, the suggested tensor function, which maps an arbitrary coordinate to the corresponding value, can continuously represent data in an infinite real space. Parallel to discrete tensors, we develop two fundamental concepts for tensor functions, i.e., the tensor function rank and low-rank tensor function factorization. We theoretically justify that both low-rank and smooth regularizations are harmoniously unified in the LRTFR, which leads to high effectiveness and efficiency for data continuous representation. Extensive multi-dimensional data recovery applications arising from image processing (image inpainting and denoising), machine learning (hyperparameter optimization), and computer graphics (point cloud upsampling) substantiate the superiority and versatility of our method as compared with state-of-the-art methods. Especially, the experiments beyond the original meshgrid resolution (hyperparameter optimization) or even beyond meshgrid (point cloud upsampling) validate the favorable performances of our method for continuous representation.