AI Algorithm for Predicting and Optimizing Trajectory of UAV Swarm

This paper explores the application of Artificial Intelligence (AI) techniques for generating the trajectories of fleets of Unmanned Aerial Vehicles (UAVs). The two main challenges addressed include accurately predicting the paths of UAVs and efficiently avoiding collisions between them. Firstly, the paper systematically applies a diverse set of activation functions to a Feedforward Neural Network (FFNN) with a single hidden layer, which enhances the accuracy of the predicted path compared to previous work. Secondly, we introduce a novel activation function, AdaptoSwelliGauss, which is a sophisticated fusion of Swish and Elliott activations, seamlessly integrated with a scaled and shifted Gaussian component. Swish facilitates smooth transitions, Elliott captures abrupt trajectory changes, and the scaled and shifted Gaussian enhances robustness against noise. This dynamic combination is specifically designed to excel in capturing the complexities of UAV trajectory prediction. This new activation function gives substantially better accuracy than all existing activation functions. Thirdly, we propose a novel Integrated Collision Detection, Avoidance, and Batching (ICDAB) strategy that merges two complementary UAV collision avoidance techniques: changing UAV trajectories and altering their starting times, also referred to as batching. This integration helps overcome the disadvantages of both - reduction in the number of trajectory manipulations, which avoids overly convoluted paths in the first technique, and smaller batch sizes, which reduce overall takeoff time in the second.

Cube Sampled K-Prototype Clustering for Featured Data

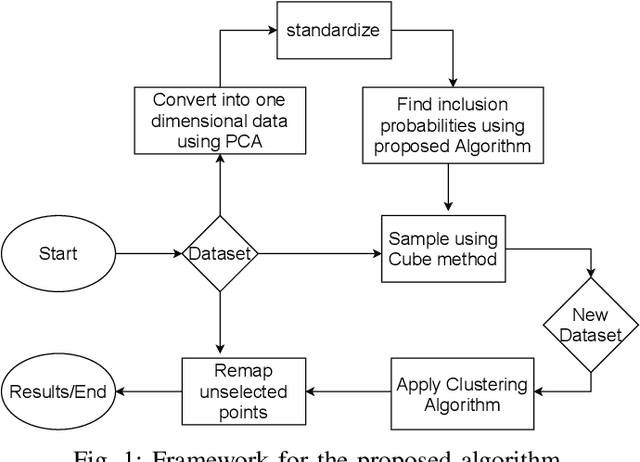
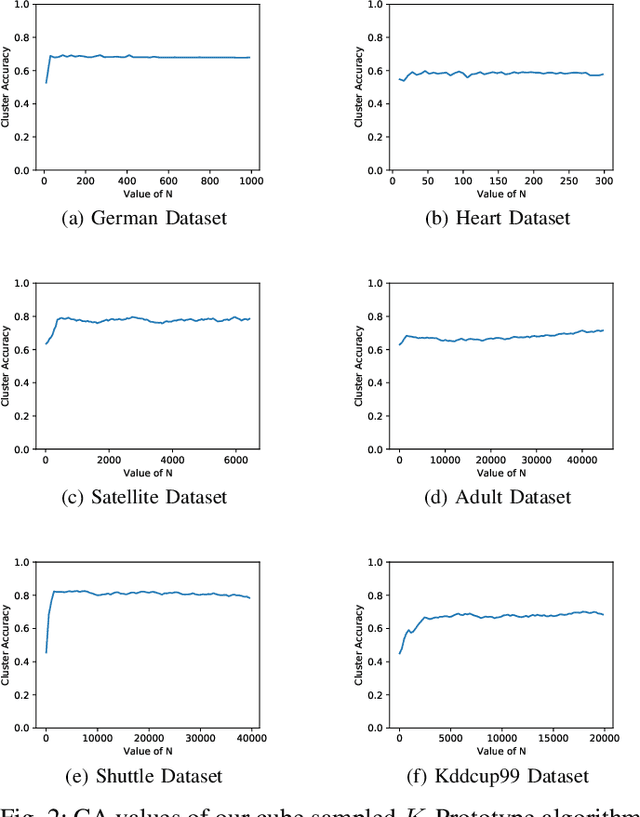
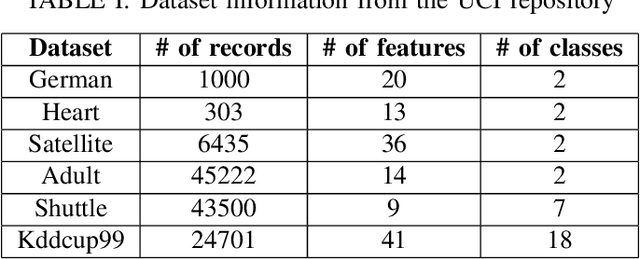
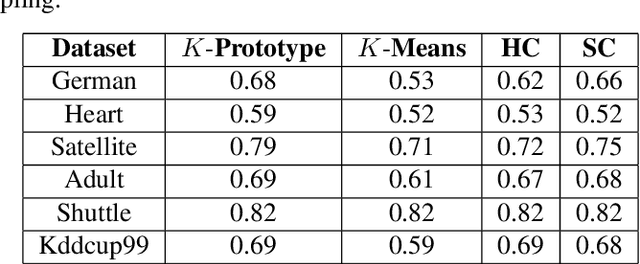
Clustering large amount of data is becoming increasingly important in the current times. Due to the large sizes of data, clustering algorithm often take too much time. Sampling this data before clustering is commonly used to reduce this time. In this work, we propose a probabilistic sampling technique called cube sampling along with K-Prototype clustering. Cube sampling is used because of its accurate sample selection. K-Prototype is most frequently used clustering algorithm when the data is numerical as well as categorical (very common in today's time). The novelty of this work is in obtaining the crucial inclusion probabilities for cube sampling using Principal Component Analysis (PCA). Experiments on multiple datasets from the UCI repository demonstrate that cube sampled K-Prototype algorithm gives the best clustering accuracy among similarly sampled other popular clustering algorithms (K-Means, Hierarchical Clustering (HC), Spectral Clustering (SC)). When compared with unsampled K-Prototype, K-Means, HC and SC, it still has the best accuracy with the added advantage of reduced computational complexity (due to reduced data size).

Probabilistically Sampled and Spectrally Clustered Plant Genotypes using Phenotypic Characteristics

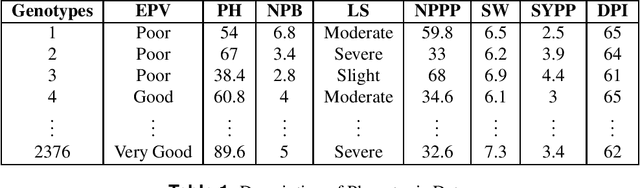
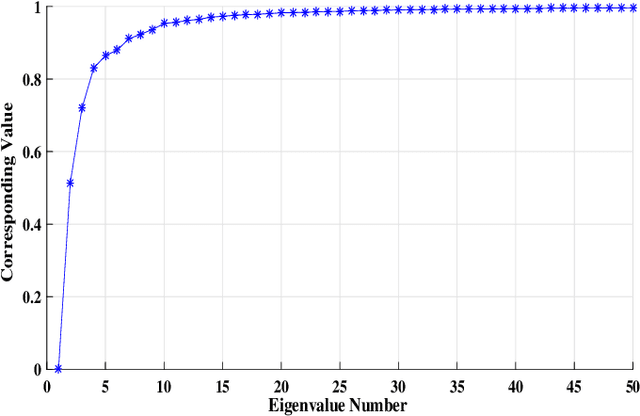
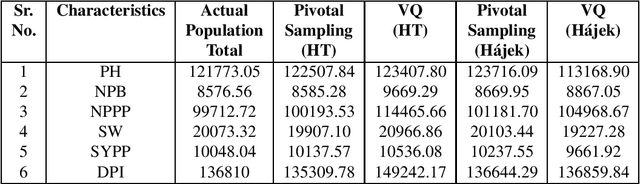
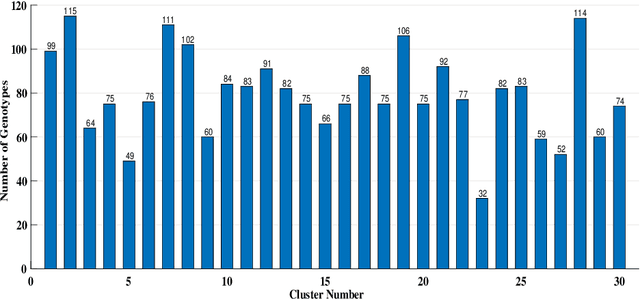
Clustering genotypes based upon their phenotypic characteristics is used to obtain diverse sets of parents that are useful in their breeding programs. The Hierarchical Clustering (HC) algorithm is the current standard in clustering of phenotypic data. This algorithm suffers from low accuracy and high computational complexity issues. To address the accuracy challenge, we propose the use of Spectral Clustering (SC) algorithm. To make the algorithm computationally cheap, we propose using sampling, specifically, Pivotal Sampling that is probability based. Since application of samplings to phenotypic data has not been explored much, for effective comparison, another sampling technique called Vector Quantization (VQ) is adapted for this data as well. VQ has recently given promising results for genome data. The novelty of our SC with Pivotal Sampling algorithm is in constructing the crucial similarity matrix for the clustering algorithm and defining probabilities for the sampling technique. Although our algorithm can be applied to any plant genotypes, we test it on the phenotypic data obtained from about 2400 Soybean genotypes. SC with Pivotal Sampling achieves substantially more accuracy (in terms of Silhouette Values) than all the other proposed competitive clustering with sampling algorithms (i.e. SC with VQ, HC with Pivotal Sampling, and HC with VQ). The complexities of our SC with Pivotal Sampling algorithm and these three variants are almost same because of the involved sampling. In addition to this, SC with Pivotal Sampling outperforms the standard HC algorithm in both accuracy and computational complexity. We experimentally show that we are up to 45% more accurate than HC in terms of clustering accuracy. The computational complexity of our algorithm is more than a magnitude lesser than HC.