Architecture-Aware Minimization (A$^2$M): How to Find Flat Minima in Neural Architecture Search

Neural Architecture Search (NAS) has become an essential tool for designing effective and efficient neural networks. In this paper, we investigate the geometric properties of neural architecture spaces commonly used in differentiable NAS methods, specifically NAS-Bench-201 and DARTS. By defining flatness metrics such as neighborhoods and loss barriers along paths in architecture space, we reveal locality and flatness characteristics analogous to the well-known properties of neural network loss landscapes in weight space. In particular, we find that highly accurate architectures cluster together in flat regions, while suboptimal architectures remain isolated, unveiling the detailed geometrical structure of the architecture search landscape. Building on these insights, we propose Architecture-Aware Minimization (A$^2$M), a novel analytically derived algorithmic framework that explicitly biases, for the first time, the gradient of differentiable NAS methods towards flat minima in architecture space. A$^2$M consistently improves generalization over state-of-the-art DARTS-based algorithms on benchmark datasets including CIFAR-10, CIFAR-100, and ImageNet16-120, across both NAS-Bench-201 and DARTS search spaces. Notably, A$^2$M is able to increase the test accuracy, on average across different differentiable NAS methods, by +3.60\% on CIFAR-10, +4.60\% on CIFAR-100, and +3.64\% on ImageNet16-120, demonstrating its superior effectiveness in practice. A$^2$M can be easily integrated into existing differentiable NAS frameworks, offering a versatile tool for future research and applications in automated machine learning. We open-source our code at https://github.com/AI-Tech-Research-Lab/AsquaredM.

ZO-DARTS++: An Efficient and Size-Variable Zeroth-Order Neural Architecture Search Algorithm

Differentiable Neural Architecture Search (NAS) provides a promising avenue for automating the complex design of deep learning (DL) models. However, current differentiable NAS methods often face constraints in efficiency, operation selection, and adaptability under varying resource limitations. We introduce ZO-DARTS++, a novel NAS method that effectively balances performance and resource constraints. By integrating a zeroth-order approximation for efficient gradient handling, employing a sparsemax function with temperature annealing for clearer and more interpretable architecture distributions, and adopting a size-variable search scheme for generating compact yet accurate architectures, ZO-DARTS++ establishes a new balance between model complexity and performance. In extensive tests on medical imaging datasets, ZO-DARTS++ improves the average accuracy by up to 1.8\% over standard DARTS-based methods and shortens search time by approximately 38.6\%. Additionally, its resource-constrained variants can reduce the number of parameters by more than 35\% while maintaining competitive accuracy levels. Thus, ZO-DARTS++ offers a versatile and efficient framework for generating high-quality, resource-aware DL models suitable for real-world medical applications.

A Lightweight Neural Architecture Search Model for Medical Image Classification

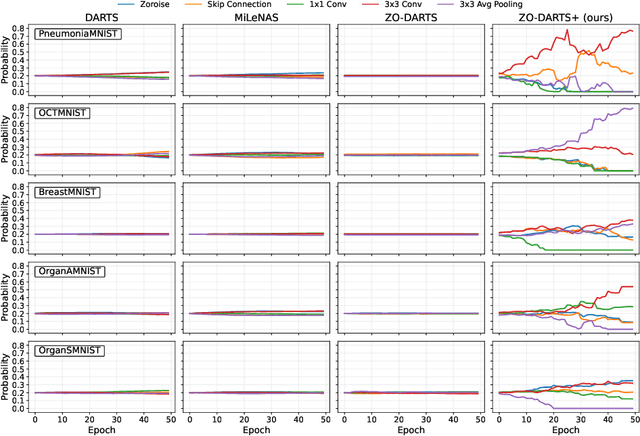
Accurate classification of medical images is essential for modern diagnostics. Deep learning advancements led clinicians to increasingly use sophisticated models to make faster and more accurate decisions, sometimes replacing human judgment. However, model development is costly and repetitive. Neural Architecture Search (NAS) provides solutions by automating the design of deep learning architectures. This paper presents ZO-DARTS+, a differentiable NAS algorithm that improves search efficiency through a novel method of generating sparse probabilities by bi-level optimization. Experiments on five public medical datasets show that ZO-DARTS+ matches the accuracy of state-of-the-art solutions while reducing search times by up to three times.

FlatNAS: optimizing Flatness in Neural Architecture Search for Out-of-Distribution Robustness

Neural Architecture Search (NAS) paves the way for the automatic definition of Neural Network (NN) architectures, attracting increasing research attention and offering solutions in various scenarios. This study introduces a novel NAS solution, called Flat Neural Architecture Search (FlatNAS), which explores the interplay between a novel figure of merit based on robustness to weight perturbations and single NN optimization with Sharpness-Aware Minimization (SAM). FlatNAS is the first work in the literature to systematically explore flat regions in the loss landscape of NNs in a NAS procedure, while jointly optimizing their performance on in-distribution data, their out-of-distribution (OOD) robustness, and constraining the number of parameters in their architecture. Differently from current studies primarily concentrating on OOD algorithms, FlatNAS successfully evaluates the impact of NN architectures on OOD robustness, a crucial aspect in real-world applications of machine and deep learning. FlatNAS achieves a good trade-off between performance, OOD generalization, and the number of parameters, by using only in-distribution data in the NAS exploration. The OOD robustness of the NAS-designed models is evaluated by focusing on robustness to input data corruptions, using popular benchmark datasets in the literature.

NACHOS: Neural Architecture Search for Hardware Constrained Early Exit Neural Networks

Early Exit Neural Networks (EENNs) endow astandard Deep Neural Network (DNN) with Early Exit Classifiers (EECs), to provide predictions at intermediate points of the processing when enough confidence in classification is achieved. This leads to many benefits in terms of effectiveness and efficiency. Currently, the design of EENNs is carried out manually by experts, a complex and time-consuming task that requires accounting for many aspects, including the correct placement, the thresholding, and the computational overhead of the EECs. For this reason, the research is exploring the use of Neural Architecture Search (NAS) to automatize the design of EENNs. Currently, few comprehensive NAS solutions for EENNs have been proposed in the literature, and a fully automated, joint design strategy taking into consideration both the backbone and the EECs remains an open problem. To this end, this work presents Neural Architecture Search for Hardware Constrained Early Exit Neural Networks (NACHOS), the first NAS framework for the design of optimal EENNs satisfying constraints on the accuracy and the number of Multiply and Accumulate (MAC) operations performed by the EENNs at inference time. In particular, this provides the joint design of backbone and EECs to select a set of admissible (i.e., respecting the constraints) Pareto Optimal Solutions in terms of best tradeoff between the accuracy and number of MACs. The results show that the models designed by NACHOS are competitive with the state-of-the-art EENNs. Additionally, this work investigates the effectiveness of two novel regularization terms designed for the optimization of the auxiliary classifiers of the EENN