List Learning with Attribute Noise

We introduce and study the model of list learning with attribute noise. Learning with attribute noise was introduced by Shackelford and Volper (COLT 1988) as a variant of PAC learning, in which the algorithm has access to noisy examples and uncorrupted labels, and the goal is to recover an accurate hypothesis. Sloan (COLT 1988) and Goldman and Sloan (Algorithmica 1995) discovered information-theoretic limits to learning in this model, which have impeded further progress. In this article we extend the model to that of list learning, drawing inspiration from the list-decoding model in coding theory, and its recent variant studied in the context of learning. On the positive side, we show that sparse conjunctions can be efficiently list learned under some assumptions on the underlying ground-truth distribution. On the negative side, our results show that even in the list-learning model, efficient learning of parities and majorities is not possible regardless of the representation used.

Nearly Optimal Deterministic Algorithm for Sparse Walsh-Hadamard Transform

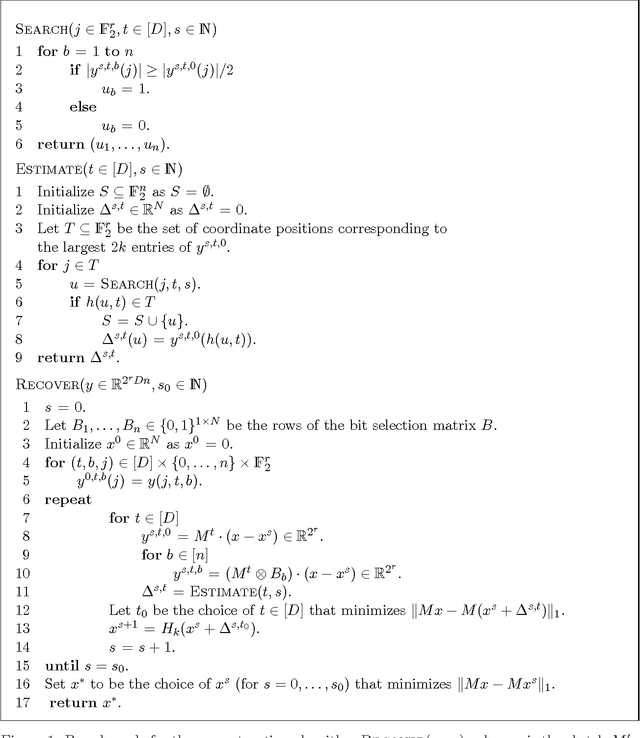
For every fixed constant $\alpha > 0$, we design an algorithm for computing the $k$-sparse Walsh-Hadamard transform of an $N$-dimensional vector $x \in \mathbb{R}^N$ in time $k^{1+\alpha} (\log N)^{O(1)}$. Specifically, the algorithm is given query access to $x$ and computes a $k$-sparse $\tilde{x} \in \mathbb{R}^N$ satisfying $\|\tilde{x} - \hat{x}\|_1 \leq c \|\hat{x} - H_k(\hat{x})\|_1$, for an absolute constant $c > 0$, where $\hat{x}$ is the transform of $x$ and $H_k(\hat{x})$ is its best $k$-sparse approximation. Our algorithm is fully deterministic and only uses non-adaptive queries to $x$ (i.e., all queries are determined and performed in parallel when the algorithm starts). An important technical tool that we use is a construction of nearly optimal and linear lossless condensers which is a careful instantiation of the GUV condenser (Guruswami, Umans, Vadhan, JACM 2009). Moreover, we design a deterministic and non-adaptive $\ell_1/\ell_1$ compressed sensing scheme based on general lossless condensers that is equipped with a fast reconstruction algorithm running in time $k^{1+\alpha} (\log N)^{O(1)}$ (for the GUV-based condenser) and is of independent interest. Our scheme significantly simplifies and improves an earlier expander-based construction due to Berinde, Gilbert, Indyk, Karloff, Strauss (Allerton 2008). Our methods use linear lossless condensers in a black box fashion; therefore, any future improvement on explicit constructions of such condensers would immediately translate to improved parameters in our framework (potentially leading to $k (\log N)^{O(1)}$ reconstruction time with a reduced exponent in the poly-logarithmic factor, and eliminating the extra parameter $\alpha$). Finally, by allowing the algorithm to use randomness, while still using non-adaptive queries, the running time of the algorithm can be improved to $\tilde{O}(k \log^3 N)$.

Submodular Functions Are Noise Stable

We show that all non-negative submodular functions have high {\em noise-stability}. As a consequence, we obtain a polynomial-time learning algorithm for this class with respect to any product distribution on $\{-1,1\}^n$ (for any constant accuracy parameter $\epsilon$). Our algorithm also succeeds in the agnostic setting. Previous work on learning submodular functions required either query access or strong assumptions about the types of submodular functions to be learned (and did not hold in the agnostic setting).