D-Tracker: Modeling Interest Diffusion in Social Activity Tensor Data Streams

Large quantities of social activity data, such as weekly web search volumes and the number of new infections with infectious diseases, reflect peoples' interests and activities. It is important to discover temporal patterns from such data and to forecast future activities accurately. However, modeling and forecasting social activity data streams is difficult because they are high-dimensional and composed of multiple time-varying dynamics such as trends, seasonality, and interest diffusion. In this paper, we propose D-Tracker, a method for continuously capturing time-varying temporal patterns within social activity tensor data streams and forecasting future activities. Our proposed method has the following properties: (a) Interpretable: it incorporates the partial differential equation into a tensor decomposition framework and captures time-varying temporal patterns such as trends, seasonality, and interest diffusion between locations in an interpretable manner; (b) Automatic: it has no hyperparameters and continuously models tensor data streams fully automatically; (c) Scalable: the computation time of D-Tracker is independent of the time series length. Experiments using web search volume data obtained from GoogleTrends, and COVID-19 infection data obtained from COVID-19 Open Data Repository show that our method can achieve higher forecasting accuracy in less computation time than existing methods while extracting the interest diffusion between locations. Our source code and datasets are available at {https://github.com/Higashiguchi-Shingo/D-Tracker.

Mining of Switching Sparse Networks for Missing Value Imputation in Multivariate Time Series

Multivariate time series data suffer from the problem of missing values, which hinders the application of many analytical methods. To achieve the accurate imputation of these missing values, exploiting inter-correlation by employing the relationships between sequences (i.e., a network) is as important as the use of temporal dependency, since a sequence normally correlates with other sequences. Moreover, exploiting an adequate network depending on time is also necessary since the network varies over time. However, in real-world scenarios, we normally know neither the network structure nor when the network changes beforehand. Here, we propose a missing value imputation method for multivariate time series, namely MissNet, that is designed to exploit temporal dependency with a state-space model and inter-correlation by switching sparse networks. The network encodes conditional independence between features, which helps us understand the important relationships for imputation visually. Our algorithm, which scales linearly with reference to the length of the data, alternatively infers networks and fills in missing values using the networks while discovering the switching of the networks. Extensive experiments demonstrate that MissNet outperforms the state-of-the-art algorithms for multivariate time series imputation and provides interpretable results.

Dynamic Multi-Network Mining of Tensor Time Series

Subsequence clustering of time series is an essential task in data mining, and interpreting the resulting clusters is also crucial since we generally do not have prior knowledge of the data. Thus, given a large collection of tensor time series consisting of multiple modes, including timestamps, how can we achieve subsequence clustering for tensor time series and provide interpretable insights? In this paper, we propose a new method, Dynamic Multi-network Mining (DMM), that converts a tensor time series into a set of segment groups of various lengths (i.e., clusters) characterized by a dependency network constrained with l1-norm. Our method has the following properties. (a) Interpretable: it characterizes the cluster with multiple networks, each of which is a sparse dependency network of a corresponding non-temporal mode, and thus provides visible and interpretable insights into the key relationships. (b) Accurate: it discovers the clusters with distinct networks from tensor time series according to the minimum description length (MDL). (c) Scalable: it scales linearly in terms of the input data size when solving a non-convex problem to optimize the number of segments and clusters, and thus it is applicable to long-range and high-dimensional tensors. Extensive experiments with synthetic datasets confirm that our method outperforms the state-of-the-art methods in terms of clustering accuracy. We then use real datasets to demonstrate that DMM is useful for providing interpretable insights from tensor time series.

Fast and Multi-aspect Mining of Complex Time-stamped Event Streams

Given a huge, online stream of time-evolving events with multiple attributes, such as online shopping logs: (item, price, brand, time), and local mobility activities: (pick-up and drop-off locations, time), how can we summarize large, dynamic high-order tensor streams? How can we see any hidden patterns, rules, and anomalies? Our answer is to focus on two types of patterns, i.e., ''regimes'' and ''components'', for which we present CubeScope, an efficient and effective method over high-order tensor streams. Specifically, it identifies any sudden discontinuity and recognizes distinct dynamical patterns, ''regimes'' (e.g., weekday/weekend/holiday patterns). In each regime, it also performs multi-way summarization for all attributes (e.g., item, price, brand, and time) and discovers hidden ''components'' representing latent groups (e.g., item/brand groups) and their relationship. Thanks to its concise but effective summarization, CubeScope can also detect the sudden appearance of anomalies and identify the types of anomalies that occur in practice. Our proposed method has the following properties: (a) Effective: it captures dynamical multi-aspect patterns, i.e., regimes and components, and statistically summarizes all the events; (b) General: it is practical for successful application to data compression, pattern discovery, and anomaly detection on various types of tensor streams; (c) Scalable: our algorithm does not depend on the length of the data stream and its dimensionality. Extensive experiments on real datasets demonstrate that CubeScope finds meaningful patterns and anomalies correctly, and consistently outperforms the state-of-the-art methods as regards accuracy and execution speed.

SSMF: Shifting Seasonal Matrix Factorization

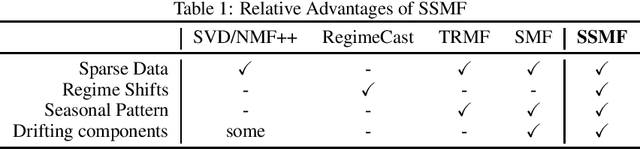
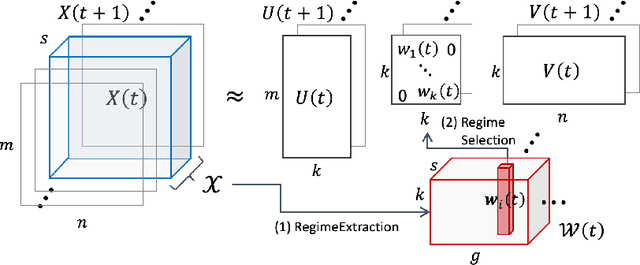
Given taxi-ride counts information between departure and destination locations, how can we forecast their future demands? In general, given a data stream of events with seasonal patterns that innovate over time, how can we effectively and efficiently forecast future events? In this paper, we propose Shifting Seasonal Matrix Factorization approach, namely SSMF, that can adaptively learn multiple seasonal patterns (called regimes), as well as switching between them. Our proposed method has the following properties: (a) it accurately forecasts future events by detecting regime shifts in seasonal patterns as the data stream evolves; (b) it works in an online setting, i.e., processes each observation in constant time and memory; (c) it effectively realizes regime shifts without human intervention by using a lossless data compression scheme. We demonstrate that our algorithm outperforms state-of-the-art baseline methods by accurately forecasting upcoming events on three real-world data streams.