Computational Identification of Regulatory Statements in EU Legislation

Identifying regulatory statements in legislation is useful for developing metrics to measure the regulatory density and strictness of legislation. A computational method is valuable for scaling the identification of such statements from a growing body of EU legislation, constituting approximately 180,000 published legal acts between 1952 and 2023. Past work on extraction of these statements varies in the permissiveness of their definitions for what constitutes a regulatory statement. In this work, we provide a specific definition for our purposes based on the institutional grammar tool. We develop and compare two contrasting approaches for automatically identifying such statements in EU legislation, one based on dependency parsing, and the other on a transformer-based machine learning model. We found both approaches performed similarly well with accuracies of 80% and 84% respectively and a K alpha of 0.58. The high accuracies and not exceedingly high agreement suggests potential for combining strengths of both approaches.

TAPS Responsibility Matrix: A tool for responsible data science by design

Data science is an interdisciplinary research area where scientists are typically working with data coming from different fields. When using and analyzing data, the scientists implicitly agree to follow standards, procedures, and rules set in these fields. However, guidance on the responsibilities of the data scientists and the other involved actors in a data science project is typically missing. While literature shows that novel frameworks and tools are being proposed in support of open-science, data reuse, and research data management, there are currently no frameworks that can fully express responsibilities of a data science project. In this paper, we describe the Transparency, Accountability, Privacy, and Societal Responsibility Matrix (TAPS-RM) as framework to explore social, legal, and ethical aspects of data science projects. TAPS-RM acts as a tool to provide users with a holistic view of their project beyond key outcomes and clarifies the responsibilities of actors. We map the developed model of TAPS-RM with well-known initiatives for open data (such as FACT, FAIR and Datasheets for datasets). We conclude that TAPS-RM is a tool to reflect on responsibilities at a data science project level and can be used to advance responsible data science by design.

Theoretical Foundations of Defeasible Description Logics

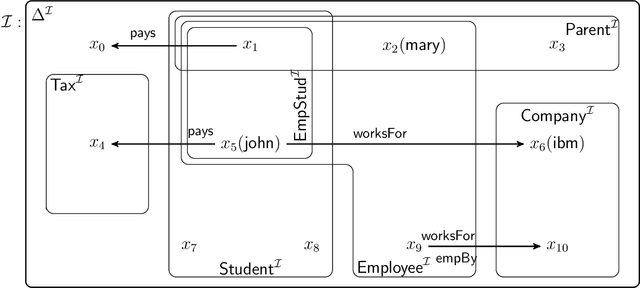
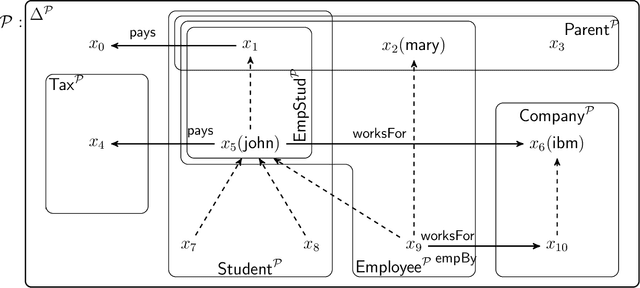
We extend description logics (DLs) with non-monotonic reasoning features. We start by investigating a notion of defeasible subsumption in the spirit of defeasible conditionals as studied by Kraus, Lehmann and Magidor in the propositional case. In particular, we consider a natural and intuitive semantics for defeasible subsumption, and investigate KLM-style syntactic properties for both preferential and rational subsumption. Our contribution includes two representation results linking our semantic constructions to the set of preferential and rational properties considered. Besides showing that our semantics is appropriate, these results pave the way for more effective decision procedures for defeasible reasoning in DLs. Indeed, we also analyse the problem of non-monotonic reasoning in DLs at the level of entailment and present an algorithm for the computation of rational closure of a defeasible ontology. Importantly, our algorithm relies completely on classical entailment and shows that the computational complexity of reasoning over defeasible ontologies is no worse than that of reasoning in the underlying classical DL ALC.