Detecting and Diagnosing Terrestrial Gravitational-Wave Mimics Through Feature Learning

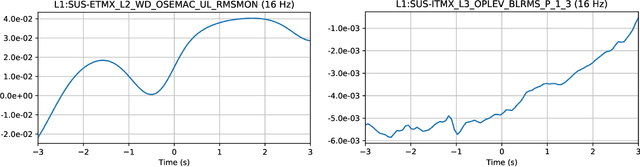
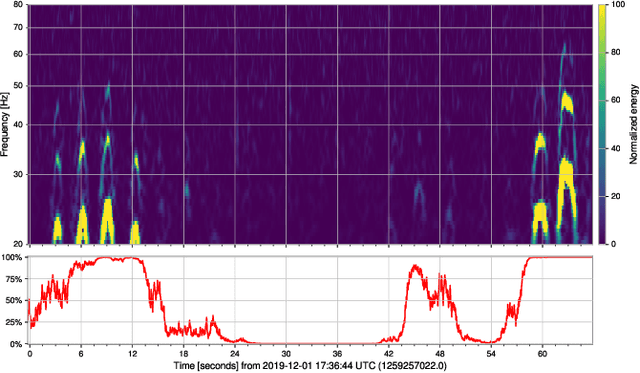
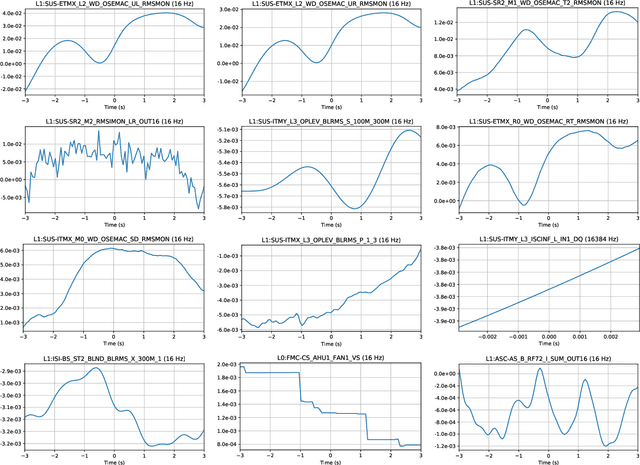
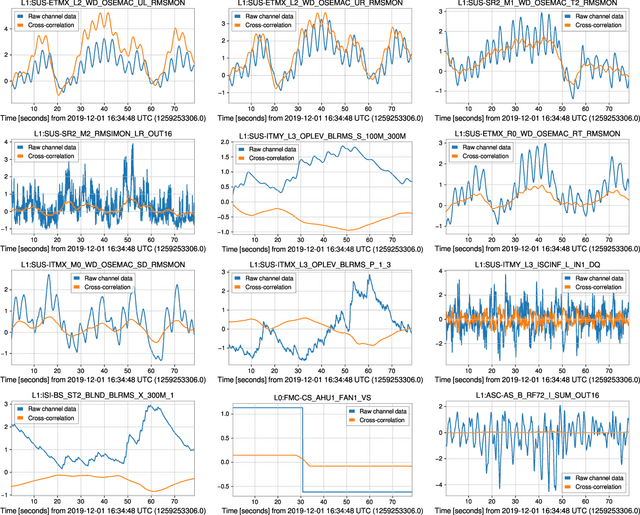
As engineered systems grow in complexity, there is an increasing need for automatic methods that can detect, diagnose, and even correct transient anomalies that inevitably arise and can be difficult or impossible to diagnose and fix manually. Among the most sensitive and complex systems of our civilization are the detectors that search for incredibly small variations in distance caused by gravitational waves -- phenomena originally predicted by Albert Einstein to emerge and propagate through the universe as the result of collisions between black holes and other massive objects in deep space. The extreme complexity and precision of such detectors causes them to be subject to transient noise issues that can significantly limit their sensitivity and effectiveness. In this work, we present a demonstration of a method that can detect and characterize emergent transient anomalies of such massively complex systems. We illustrate the performance, precision, and adaptability of the automated solution via one of the prevalent issues limiting gravitational-wave discoveries: noise artifacts of terrestrial origin that contaminate gravitational wave observatories' highly sensitive measurements and can obscure or even mimic the faint astrophysical signals for which they are listening. Specifically, we demonstrate how a highly interpretable convolutional classifier can automatically learn to detect transient anomalies from auxiliary detector data without needing to observe the anomalies themselves. We also illustrate several other useful features of the model, including how it performs automatic variable selection to reduce tens of thousands of auxiliary data channels to only a few relevant ones; how it identifies behavioral signatures predictive of anomalies in those channels; and how it can be used to investigate individual anomalies and the channels associated with them.

Architectural Optimization and Feature Learning for High-Dimensional Time Series Datasets

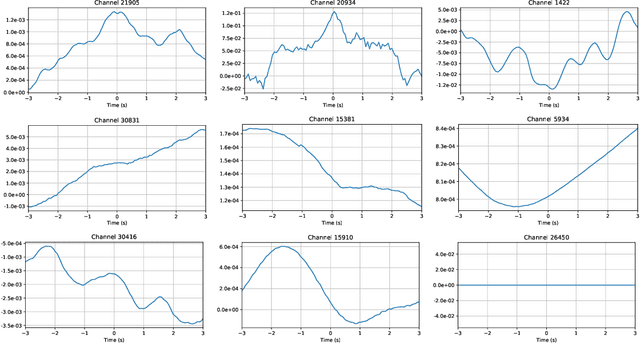
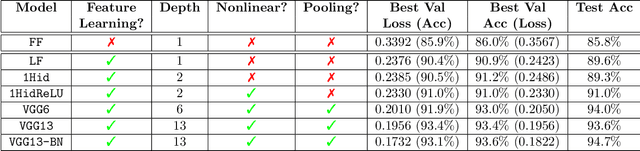
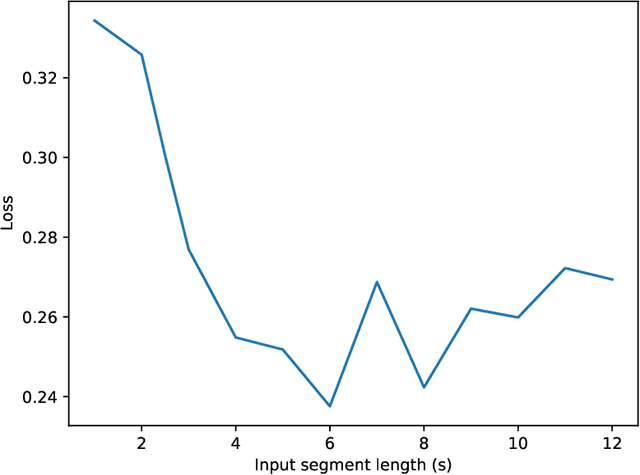
As our ability to sense increases, we are experiencing a transition from data-poor problems, in which the central issue is a lack of relevant data, to data-rich problems, in which the central issue is to identify a few relevant features in a sea of observations. Motivated by applications in gravitational-wave astrophysics, we study the problem of predicting the presence of transient noise artifacts in a gravitational wave detector from a rich collection of measurements from the detector and its environment. We argue that feature learning--in which relevant features are optimized from data--is critical to achieving high accuracy. We introduce models that reduce the error rate by over 60\% compared to the previous state of the art, which used fixed, hand-crafted features. Feature learning is useful not only because it improves performance on prediction tasks; the results provide valuable information about patterns associated with phenomena of interest that would otherwise be undiscoverable. In our application, features found to be associated with transient noise provide diagnostic information about its origin and suggest mitigation strategies. Learning in high-dimensional settings is challenging. Through experiments with a variety of architectures, we identify two key factors in successful models: sparsity, for selecting relevant variables within the high-dimensional observations; and depth, which confers flexibility for handling complex interactions and robustness with respect to temporal variations. We illustrate their significance through systematic experiments on real detector data. Our results provide experimental corroboration of common assumptions in the machine-learning community and have direct applicability to improving our ability to sense gravitational waves, as well as to many other problem settings with similarly high-dimensional, noisy, or partly irrelevant data.

Compressed Sensing Microscopy with Scanning Line Probes

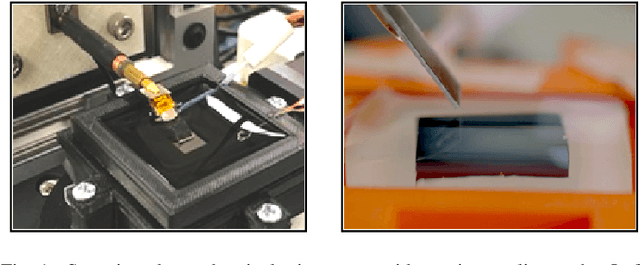
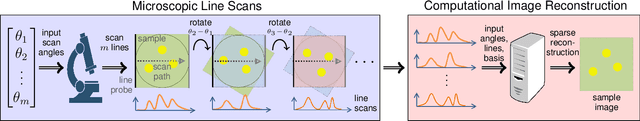
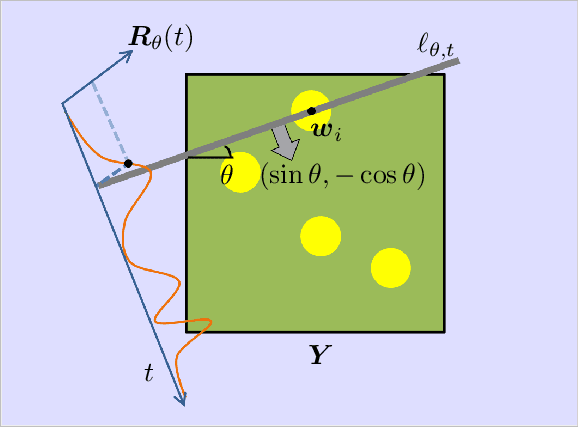
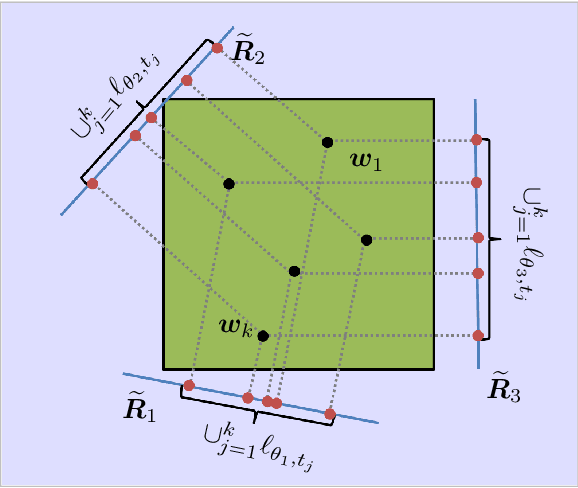
In applications of scanning probe microscopy, images are acquired by raster scanning a point probe across a sample. Viewed from the perspective of compressed sensing (CS), this pointwise sampling scheme is inefficient, especially when the target image is structured. While replacing point measurements with delocalized, incoherent measurements has the potential to yield order-of-magnitude improvements in scan time, implementing the delocalized measurements of CS theory is challenging. In this paper we study a partially delocalized probe construction, in which the point probe is replaced with a continuous line, creating a sensor which essentially acquires line integrals of the target image. We show through simulations, rudimentary theoretical analysis, and experiments, that these line measurements can image sparse samples far more efficiently than traditional point measurements, provided the local features in the sample are enough separated. Despite this promise, practical reconstruction from line measurements poses additional difficulties: the measurements are partially coherent, and real measurements exhibit nonidealities. We show how to overcome these limitations using natural strategies (reweighting to cope with coherence, blind calibration for nonidealities), culminating in an end-to-end demonstration.

Dictionary Learning in Fourier Transform Scanning Tunneling Spectroscopy

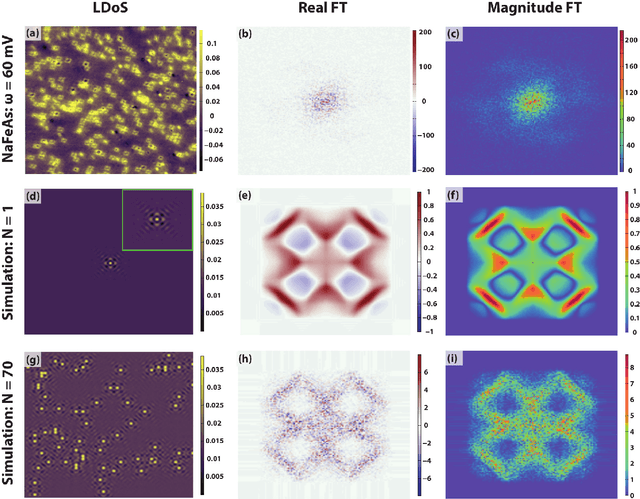
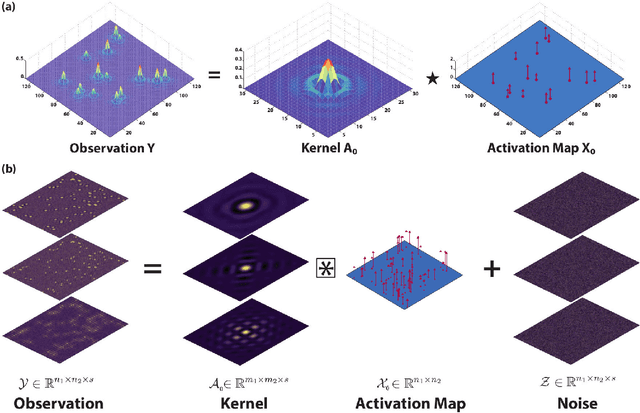
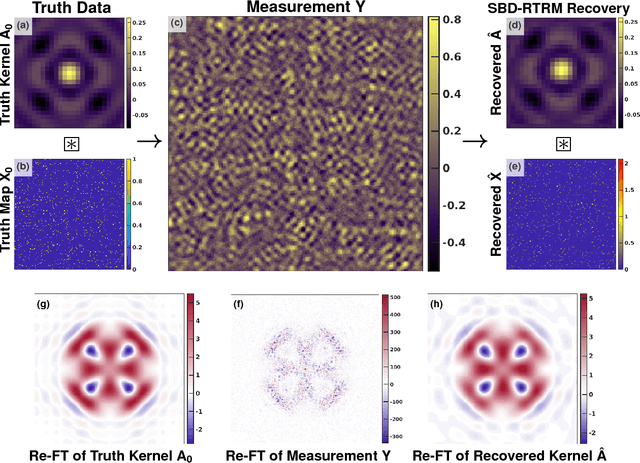
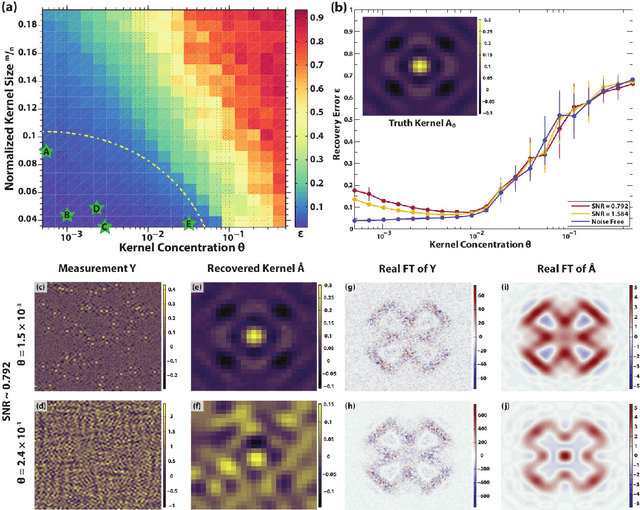
Modern high-resolution microscopes, such as the scanning tunneling microscope, are commonly used to study specimens that have dense and aperiodic spatial structure. Extracting meaningful information from images obtained from such microscopes remains a formidable challenge. Fourier analysis is commonly used to analyze the underlying structure of fundamental motifs present in an image. However, the Fourier transform fundamentally suffers from severe phase noise when applied to aperiodic images. Here, we report the development of a new algorithm based on nonconvex optimization, applicable to any microscopy modality, that directly uncovers the fundamental motifs present in a real-space image. Apart from being quantitatively superior to traditional Fourier analysis, we show that this novel algorithm also uncovers phase sensitive information about the underlying motif structure. We demonstrate its usefulness by studying scanning tunneling microscopy images of a Co-doped iron arsenide superconductor and prove that the application of the algorithm allows for the complete recovery of quasiparticle interference in this material. Our phase sensitive quasiparticle interference imaging results indicate that the pairing symmetry in optimally doped NaFeAs is consistent with a sign-changing s+- order parameter.