CrackCLF: Automatic Pavement Crack Detection based on Closed-Loop Feedback

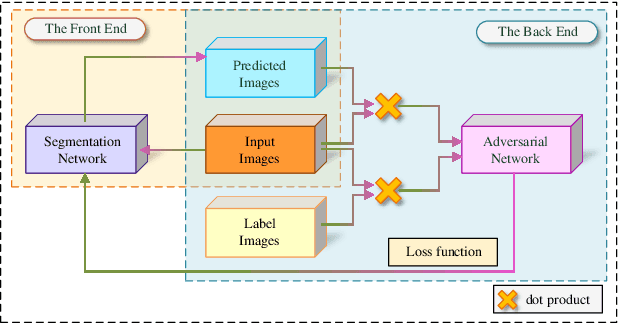
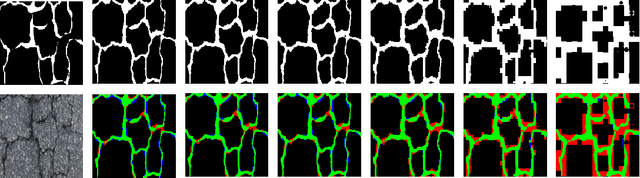
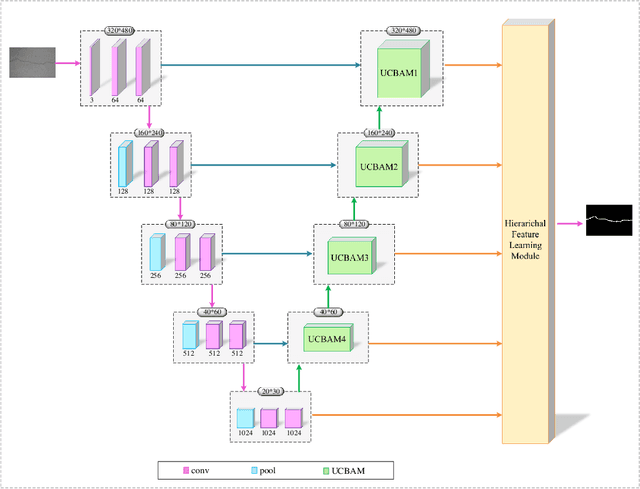
Automatic pavement crack detection is an important task to ensure the functional performances of pavements during their service life. Inspired by deep learning (DL), the encoder-decoder framework is a powerful tool for crack detection. However, these models are usually open-loop (OL) systems that tend to treat thin cracks as the background. Meanwhile, these models can not automatically correct errors in the prediction, nor can it adapt to the changes of the environment to automatically extract and detect thin cracks. To tackle this problem, we embed closed-loop feedback (CLF) into the neural network so that the model could learn to correct errors on its own, based on generative adversarial networks (GAN). The resulting model is called CrackCLF and includes the front and back ends, i.e. segmentation and adversarial network. The front end with U-shape framework is employed to generate crack maps, and the back end with a multi-scale loss function is used to correct higher-order inconsistencies between labels and crack maps (generated by the front end) to address open-loop system issues. Empirical results show that the proposed CrackCLF outperforms others methods on three public datasets. Moreover, the proposed CLF can be defined as a plug and play module, which can be embedded into different neural network models to improve their performances.

A New Repair Operator for Multi-objective Evolutionary Algorithm in Constrained Optimization Problems

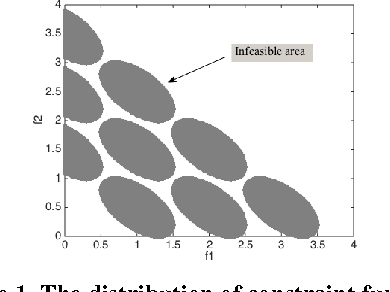
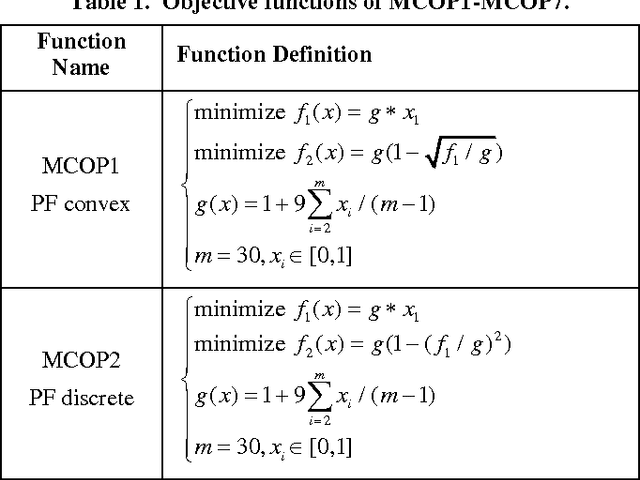
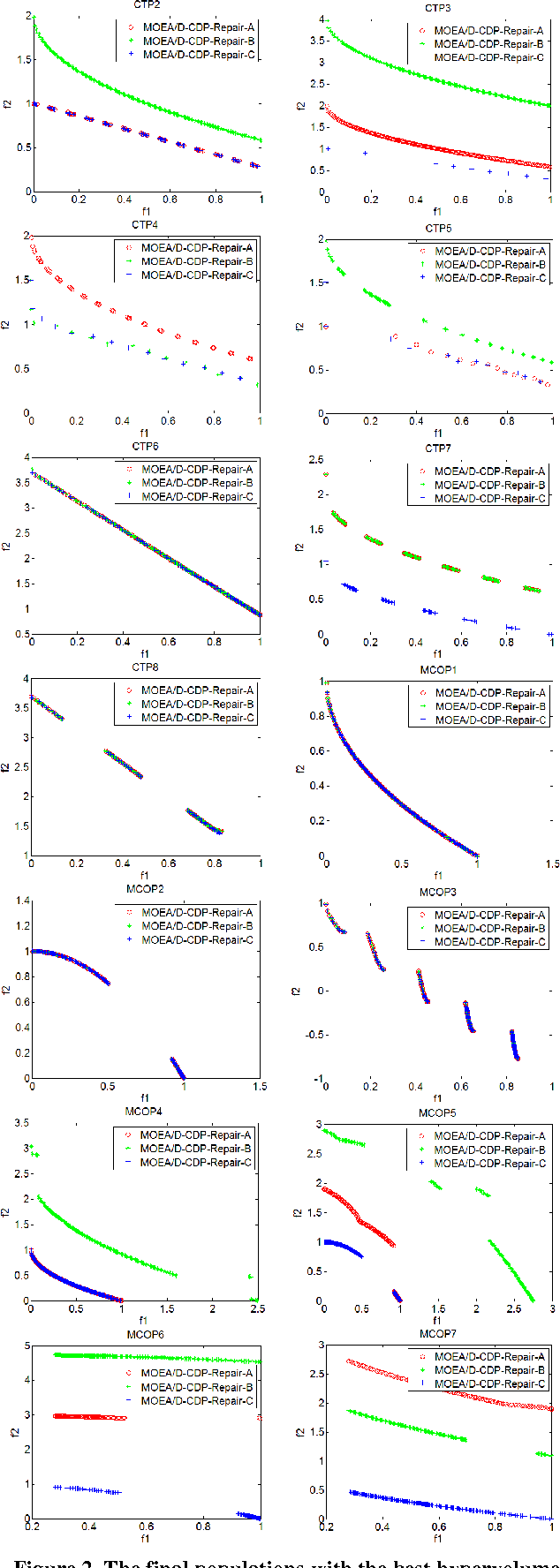
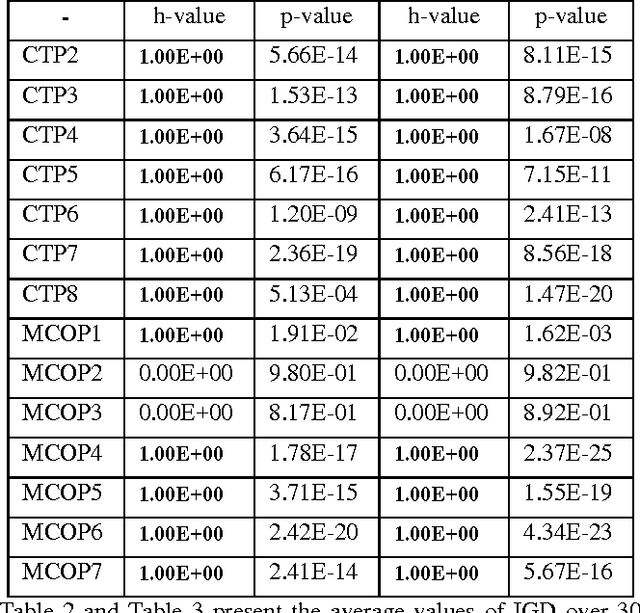
In this paper, we design a set of multi-objective constrained optimization problems (MCOPs) and propose a new repair operator to address them. The proposed repair operator is used to fix the solutions that violate the box constraints. More specifically, it employs a reversed correction strategy that can effectively avoid the population falling into local optimum. In addition, we integrate the proposed repair operator into two classical multi-objective evolutionary algorithms MOEA/D and NSGA-II. The proposed repair operator is compared with other two kinds of commonly used repair operators on benchmark problems CTPs and MCOPs. The experiment results demonstrate that our proposed approach is very effective in terms of convergence and diversity.