Defensive Alliances in Signed Networks

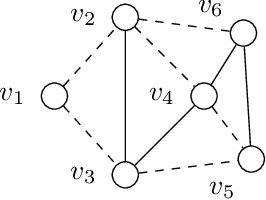
The analysis of (social) networks and multi-agent systems is a central theme in Artificial Intelligence. Some line of research deals with finding groups of agents that could work together to achieve a certain goal. To this end, different notions of so-called clusters or communities have been introduced in the literature of graphs and networks. Among these, defensive alliance is a kind of quantitative group structure. However, all studies on the alliance so for have ignored one aspect that is central to the formation of alliances on a very intuitive level, assuming that the agents are preconditioned concerning their attitude towards other agents: they prefer to be in some group (alliance) together with the agents they like, so that they are happy to help each other towards their common aim, possibly then working against the agents outside of their group that they dislike. Signed networks were introduced in the psychology literature to model liking and disliking between agents, generalizing graphs in a natural way. Hence, we propose the novel notion of a defensive alliance in the context of signed networks. We then investigate several natural algorithmic questions related to this notion. These, and also combinatorial findings, connect our notion to that of correlation clustering, which is a well-established idea of finding groups of agents within a signed network. Also, we introduce a new structural parameter for signed graphs, signed neighborhood diversity snd, and exhibit a parameterized algorithm that finds a smallest defensive alliance in a signed graph.

Unlabeled Multi-Robot Motion Planning with Tighter Separation Bounds

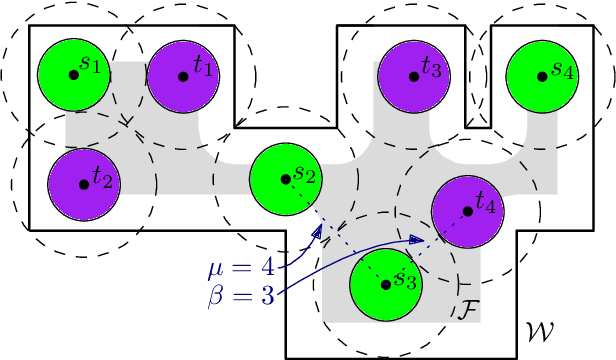
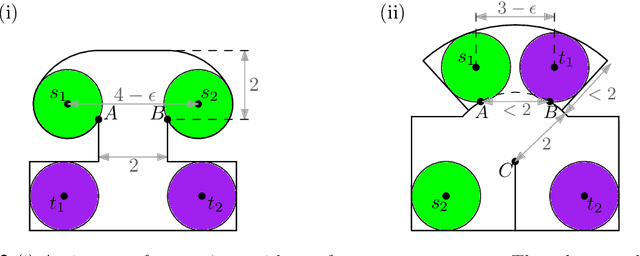
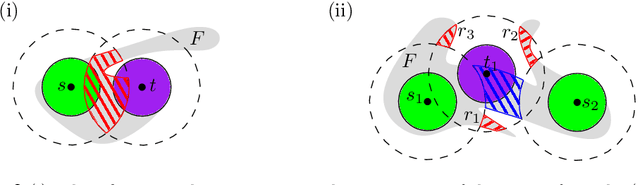
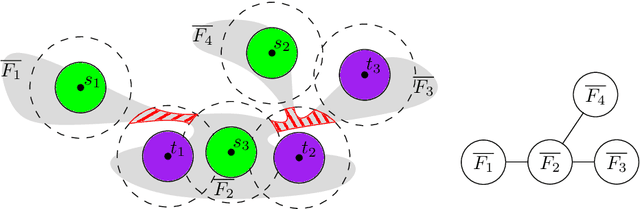
We consider the unlabeled motion-planning problem of $m$ unit-disc robots moving in a simple polygonal workspace of $n$ edges. The goal is to find a motion plan that moves the robots to a given set of $m$ target positions. For the unlabeled variant, it does not matter which robot reaches which target position as long as all target positions are occupied in the end. If the workspace has narrow passages such that the robots cannot fit through them, then the free configuration space, representing all possible unobstructed positions of the robots, will consist of multiple connected components. Even if in each component of the free space the number of targets matches the number of start positions, the motion-planning problem does not always have a solution when the robots and their targets are positioned very densely. In this paper, we prove tight bounds on how much separation between start and target positions is necessary to always guarantee a solution. Moreover, we describe an algorithm that always finds a solution in time $O(n \log n + mn + m^2)$ if the separation bounds are met. Specifically, we prove that the following separation is sufficient: any two start positions are at least distance $4$ apart, any two target positions are at least distance $4$ apart, and any pair of a start and a target positions is at least distance $3$ apart. We further show that when the free space consists of a single connected component, the separation between start and target positions is not necessary.

Diversity in Kemeny Rank Aggregation: A Parameterized Approach

In its most traditional setting, the main concern of optimization theory is the search for optimal solutions for instances of a given computational problem. A recent trend of research in artificial intelligence, called solution diversity, has focused on the development of notions of optimality that may be more appropriate in settings where subjectivity is essential. The idea is that instead of aiming at the development of algorithms that output a single optimal solution, the goal is to investigate algorithms that output a small set of sufficiently good solutions that are sufficiently diverse from one another. In this way, the user has the opportunity to choose the solution that is most appropriate to the context at hand. It also displays the richness of the solution space. When combined with techniques from parameterized complexity theory, the paradigm of diversity of solutions offers a powerful algorithmic framework to address problems of practical relevance. In this work, we investigate the impact of this combination in the field of Kemeny Rank Aggregation, a well-studied class of problems lying in the intersection of order theory and social choice theory and also in the field of order theory itself. In particular, we show that the Kemeny Rank Aggregation problem is fixed-parameter tractable with respect to natural parameters providing natural formalizations of the notions of diversity and of the notion of a sufficiently good solution. Our main results work both when considering the traditional setting of aggregation over linearly ordered votes, and in the more general setting where votes are partially ordered.

Regular Intersection Emptiness of Graph Problems: Finding a Needle in a Haystack of Graphs with the Help of Automata

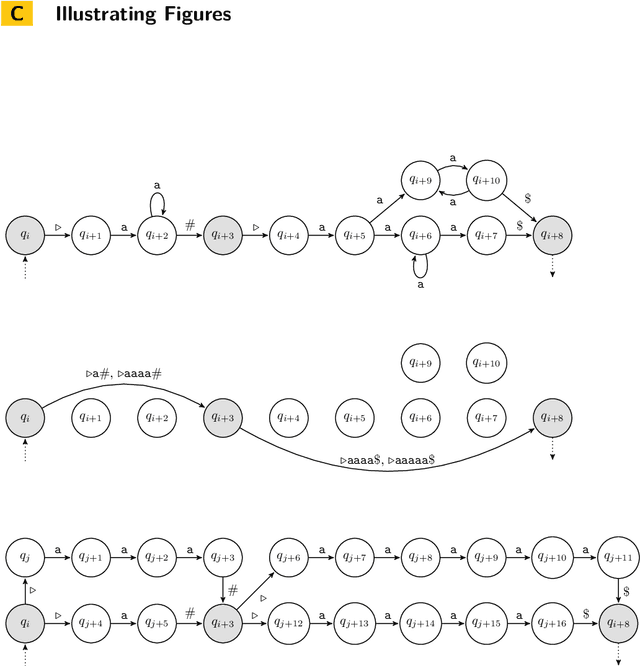
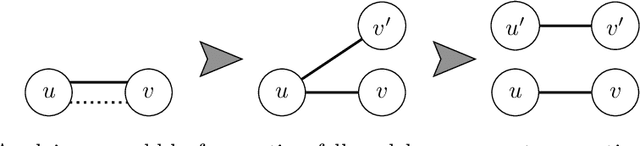
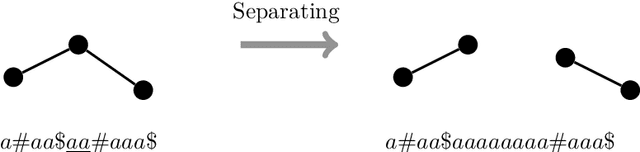
The Int_reg-problem of a combinatorial problem P asks, given a nondeterministic automaton M as input, whether the language L(M) accepted by M contains any positive instance of the problem P. We consider the Int_reg-problem for a number of different graph problems and give general criteria that give decision procedures for these Int_reg-problems. To achieve this goal, we consider a natural graph encoding so that the language of all graph encodings is regular. Then, we draw the connection between classical pumping- and interchange-arguments from the field of formal language theory with the graph operations induced on the encoded graph. Our techniques apply among others to the Int_reg-problem of well-known graph problems like Vertex Cover and Independent Set, as well as to subgraph problems, graph-edit problems and graph-partitioning problems, including coloring problems.