Synthesis of Reward Machines for Multi-Agent Equilibrium Design (Full Version)

Mechanism design is a well-established game-theoretic paradigm for designing games to achieve desired outcomes. This paper addresses a closely related but distinct concept, equilibrium design. Unlike mechanism design, the designer's authority in equilibrium design is more constrained; she can only modify the incentive structures in a given game to achieve certain outcomes without the ability to create the game from scratch. We study the problem of equilibrium design using dynamic incentive structures, known as reward machines. We use weighted concurrent game structures for the game model, with goals (for the players and the designer) defined as mean-payoff objectives. We show how reward machines can be used to represent dynamic incentives that allocate rewards in a manner that optimises the designer's goal. We also introduce the main decision problem within our framework, the payoff improvement problem. This problem essentially asks whether there exists a dynamic incentive (represented by some reward machine) that can improve the designer's payoff by more than a given threshold value. We present two variants of the problem: strong and weak. We demonstrate that both can be solved in polynomial time using a Turing machine equipped with an NP oracle. Furthermore, we also establish that these variants are either NP-hard or coNP-hard. Finally, we show how to synthesise the corresponding reward machine if it exists.

Optimal Alignment of Temporal Knowledge Bases

Answering temporal CQs over temporalized Description Logic knowledge bases (TKB) is a main technique to realize ontology-based situation recognition. In case the collected data in such a knowledge base is inaccurate, important query answers can be missed. In this paper we introduce the TKB Alignment problem, which computes a variant of the TKB that minimally changes the TKB, but entails the given temporal CQ and is in that sense (cost-)optimal. We investigate this problem for ALC TKBs and conjunctive queries with LTL operators and devise a solution technique to compute (cost-optimal) alignments of TKBs that extends techniques for the alignment problem for propositional LTL over finite traces.

On the Complexity of Rational Verification

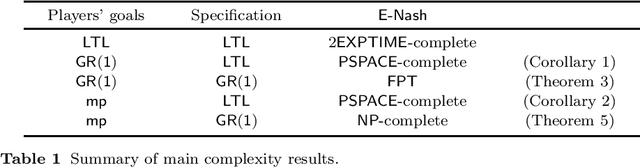
Rational verification refers to the problem of checking which temporal logic properties hold of a concurrent multiagent system, under the assumption that agents in the system choose strategies that form a game-theoretic equilibrium. Rational verification can be understood as a counterpart to model checking for multiagent systems, but while classical model checking can be done in polynomial time for some temporal logic specification languages such as CTL, and polynomial space with LTL specifications, rational verification is much harder: the key decision problems for rational verification are 2EXPTIME-complete with LTL specifications, even when using explicit-state system representations. Against this background, our contributions in this paper are threefold. First, we show that the complexity of rational verification can be greatly reduced by restricting specifications to GR(1), a fragment of LTL that can represent a broad and practically useful class of response properties of reactive systems. In particular, we show that for a number of relevant settings, rational verification can be done in polynomial space and even in polynomial time. Second, we provide improved complexity results for rational verification when considering players' goals given by mean-payoff utility functions; arguably the most widely used approach for quantitative objectives in concurrent and multiagent systems. Finally, we consider the problem of computing outcomes that satisfy social welfare constraints. To this end, we consider both utilitarian and egalitarian social welfare and show that computing such outcomes is either PSPACE-complete or NP-complete.

Equilibrium Design for Concurrent Games

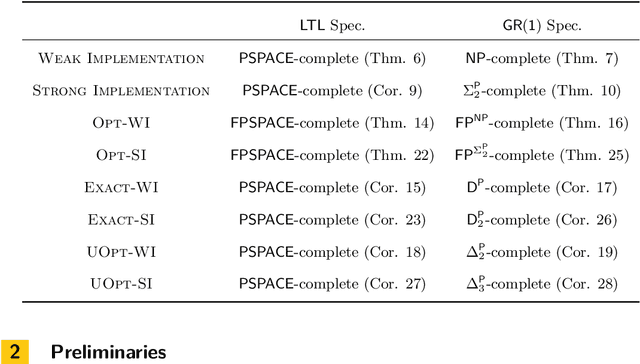
In game theory, mechanism design is concerned with the design of incentives so that a desired outcome of the game can be achieved. In this paper, we study the design of incentives so that a desirable equilibrium is obtained, for instance, an equilibrium satisfying a given temporal logic property -- a problem that we call equilibrium design. We base our study on a framework where system specifications are represented as temporal logic formulae, games as quantitative concurrent game structures, and players' goals as mean-payoff objectives. In particular, we consider system specifications given by LTL and GR(1) formulae, and show that implementing a mechanism to ensure that a given temporal logic property is satisfied on some/every Nash equilibrium of the game, whenever such a mechanism exists, can be done in PSPACE for LTL properties and in NP/$\Sigma^{P}_{2}$ for GR(1) specifications. We also study the complexity of various related decision and optimisation problems, such as optimality and uniqueness of solutions, and show that the complexities of all such problems lie within the polynomial hierarchy. As an application, equilibrium design can be used as an alternative solution to the rational synthesis and verification problems for concurrent games with mean-payoff objectives whenever no solution exists, or as a technique to repair, whenever possible, concurrent games with undesirable rational outcomes (Nash equilibria) in an optimal way.

Multi-Player Games with LDL Goals over Finite Traces

Linear Dynamic Logic on finite traces LDLf is a powerful logic for reasoning about the behaviour of concurrent and multi-agent systems. In this paper, we investigate techniques for both the characterisation and verification of equilibria in multi-player games with goals/objectives expressed using logics based on LDLf. This study builds upon a generalisation of Boolean games, a logic-based game model of multi-agent systems where players have goals succinctly represented in a logical way. Because LDLf goals are considered, in the settings we study -- Reactive Modules games and iterated Boolean games with goals over finite traces -- players' goals can be defined to be regular properties while achieved in a finite, but arbitrarily large, trace. In particular, using alternating automata, the paper investigates automata-theoretic approaches to the characterisation and verification of (pure strategy Nash) equilibria, shows that the set of Nash equilibria in multi-player games with LDLf objectives is regular, and provides complexity results for the associated automata constructions.

Equilibria for Games with Combined Qualitative and Quantitative Objectives

The overall aim of our research is to develop techniques to reason about the equilibrium properties of multi-agent systems. We model multi-agent systems as concurrent games, in which each player is a process that is assumed to act independently and strategically in pursuit of personal preferences. In this article, we study these games in the context of finite-memory strategies, and we assume players' preferences are defined by a qualitative and a quantitative objective, which are related by a lexicographic order: a player first prefers to satisfy its qualitative objective (given as a formula of Linear Temporal Logic) and then prefers to minimise costs (given by a mean-payoff function). Our main result is that deciding the existence of a strict epsilon Nash equilibrium in such games is 2ExpTime-complete (and hence decidable), even if players' deviations are implemented as infinite-memory strategies.

Automated Temporal Equilibrium Analysis: Verification and Synthesis of Multi-Player Games

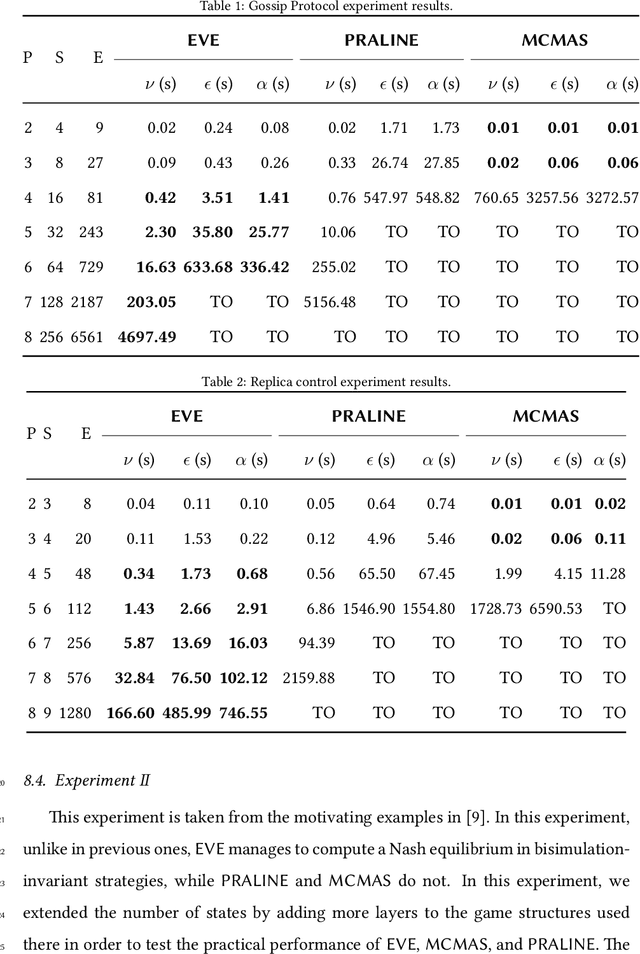
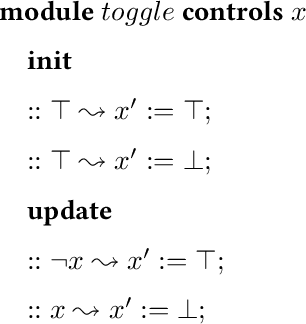
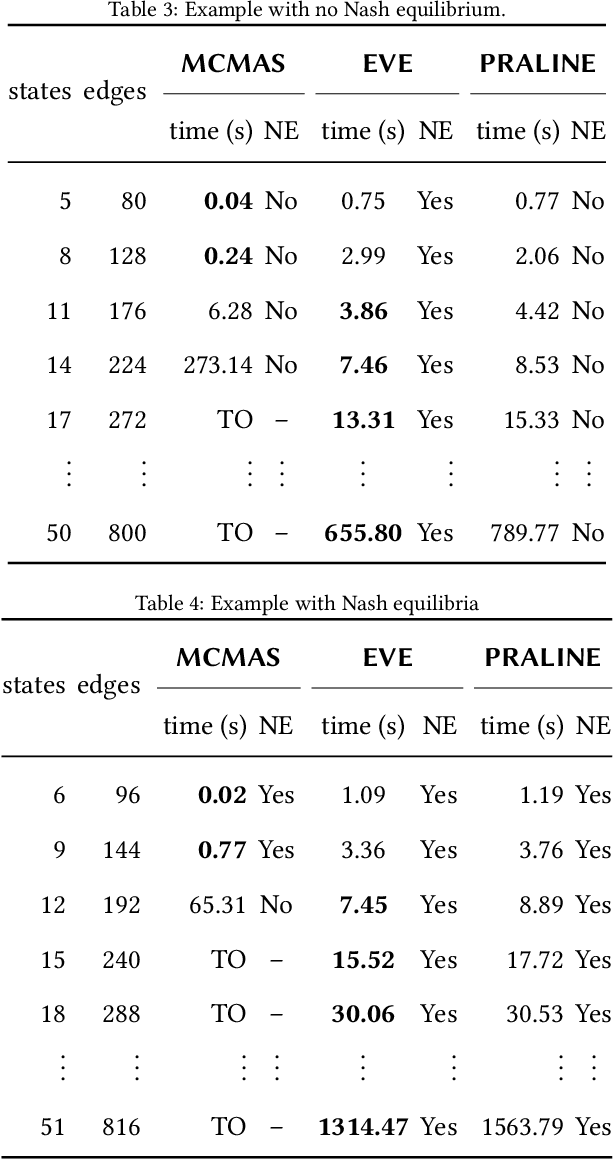
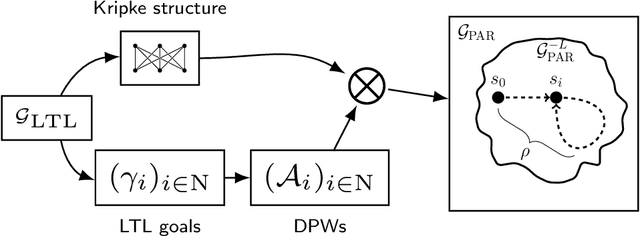
In the context of multi-agent systems, the rational verification problem is concerned with checking which temporal logic properties will hold in a system when its constituent agents are assumed to behave rationally and strategically in pursuit of individual objectives. Typically, those objectives are expressed as temporal logic formulae which the relevant agent desires to see satisfied. Unfortunately, rational verification is computationally complex, and requires specialised techniques in order to obtain practically useable implementations. In this paper, we present such a technique. This technique relies on a reduction of the rational verification problem to the solution of a collection of parity games. Our approach has been implemented in the Equilibrium Verification Environment (EVE) system. The EVE system takes as input a model of a concurrent/multi-agent system represented using the Simple Reactive Modules Language (SRML), where agent goals are represented as Linear Temporal Logic (LTL) formulae, together with a claim about the equilibrium behaviour of the system, also expressed as an LTL formula. EVE can then check whether the LTL claim holds on some (or every) computation of the system that could arise through agents choosing Nash equilibrium strategies; it can also check whether a system has a Nash equilibrium, and synthesise individual strategies for players in the multi-player game. After presenting our basic framework, we describe our new technique and prove its correctness. We then describe our implementation in the EVE system, and present experimental results which show that EVE performs favourably in comparison to other existing tools that support rational verification.

A Computational Framework for Adaptive Systems and its Verification

Modern computer systems are inherently distributed and feature autonomous and collaborative behaviour of multicomponent with global goals. These goals are expressed in terms of the combined behaviour of different components that are usually deployed in dynamic and evolving environments. It is therefore crucial to provide techniques to generate programs for collaborative and adaptive components, with guarantees of maintaining their designated global goals. To reach this endeavour, we need to extend modelling formalisms and specification languages to account for the specific features of these systems and to permit specifying both individual and system behaviour. We propose a computational framework to allow multiple components to interact in different modes, exchange information, adapt their behaviour, and reconfigure their communication interfaces. The framework permits a local interaction based on shared variables and a global one based on message passing. To be able to reason about local and global behaviour, we extend LTL to consider the exchanged messages and their constraints. Finally, we study the computational complexity of satisfiability and verification considering these extensions.