Band-ESS: Streaming Enumerative Coding with Applications to Probabilistic Shaping

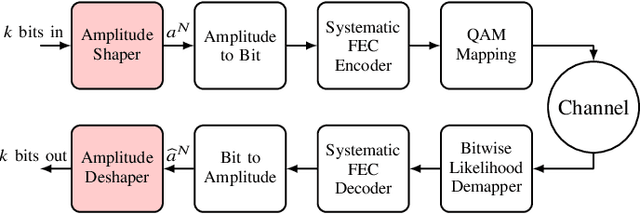
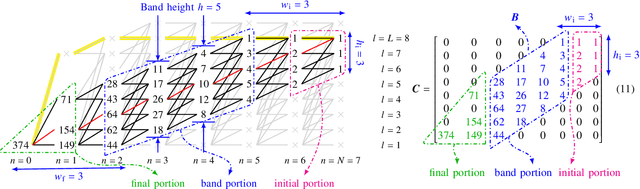
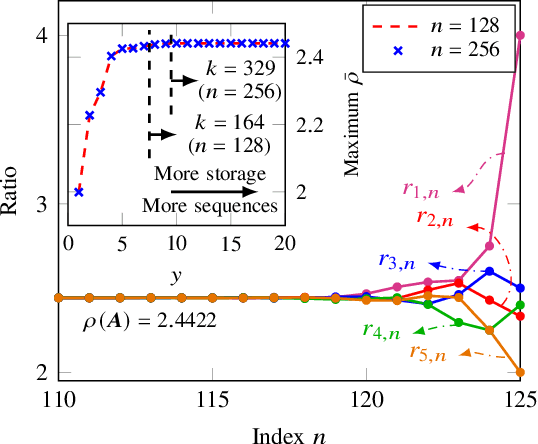
Probabilistic amplitude shaping (PAS) is on track to become the de facto coded modulation standard for communication systems aiming to operate close to channel capacity at high transmission rates. The essential component of PAS that breeds this widespread interest is the amplitude shaping block, through which the channel input distribution is controlled. This block is responsible for converting bit strings into amplitude sequences with certain properties, e.g., fixed composition, limited energy, limited energy variation, etc. Recently, band-trellis enumerative sphere shaping (B-ESS) was introduced as an amplitude shaping technique that achieves limited energy variations which is useful in optical communication scenarios. B-ESS operates based on a trellis diagram in which sequences with high energy variations are pruned. In this work, we study the implementation of B-ESS. We first show that thanks to the trellis structure obtained by this pruning, B-ESS can be implemented with very low storage complexity. The trellis computation is shown to be reduced to a set of recursive multiplications with a scalar factor. Then we show that this scalar factor can be adjusted such that the trellis computation is further simplified and realized with only binary shifts. This shift-based B-ESS (1) can be implemented for arbitrarily long blocklengths without incurring an increase in complexity, and (2) can operate in a streaming mode similar to convolutional coding.

Log-CCDM: Distribution Matching via Multiplication-free Arithmetic Coding

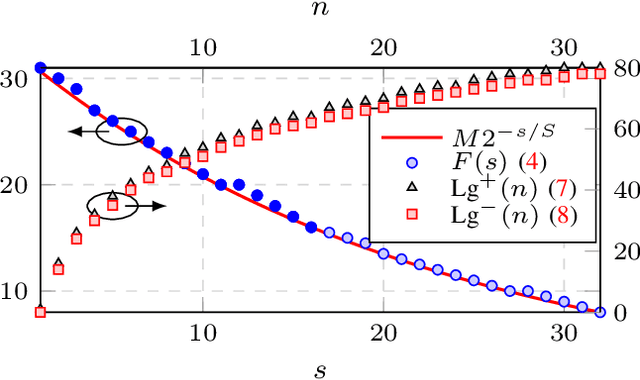
Recent years have seen renewed attention to arithmetic coding (AC). This is thanks to the use of AC for distribution matching (DM) to control the channel input distribution in probabilistic amplitude shaping. There are two main problems inherent to AC: (1) its required arithmetic precision grows linearly with the input length, and (2) high-precision multiplications and divisions are required. Here, we introduce a multiplication-free AC-based DM technique via three lookup tables (LUTs) which solves both problems above. These LUTs are used to approximate the high-precision multiplications and divisions by additions and subtractions. The required precision of our approach is shown to grow logarithmically with the input length. We prove that this approximate technique maintains the invertibility of DM. At an input length of 1024 symbols, the proposed technique achieves negligible rate loss ($<0.01$ bit/sym) against the full-precision DM, while requiring less than 4 kilobytes of storage.

Mitigating Nonlinear Interference by Limiting Energy Variations in Sphere Shaping

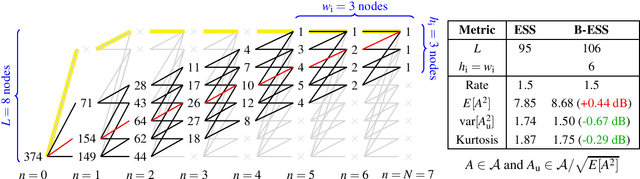
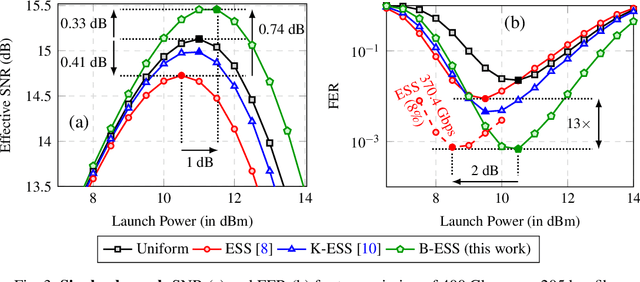
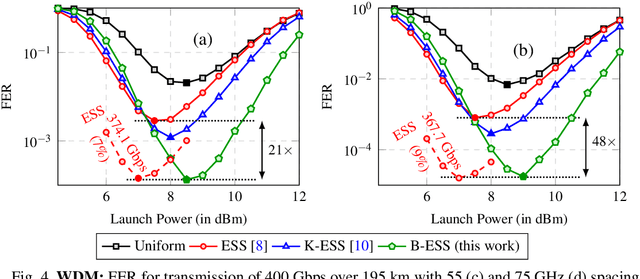
Band-trellis enumerative sphere shaping is proposed to decrease the energy variations in channel input sequences. Against sphere shaping, 0.74 dB SNR gain and up to 9% increase in data rates are demonstrated for single-span systems.

List-encoding CCDM: A Nonlinearity-tolerant Shaper Aided by Energy Dispersion Index

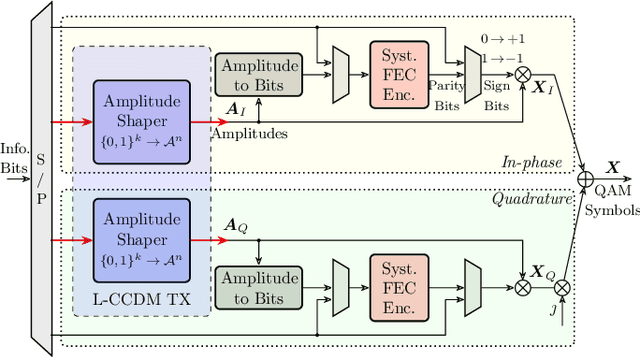
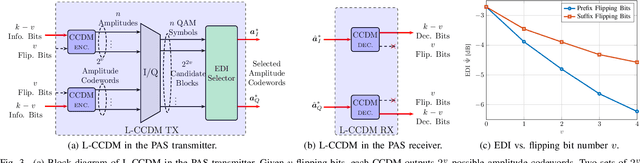
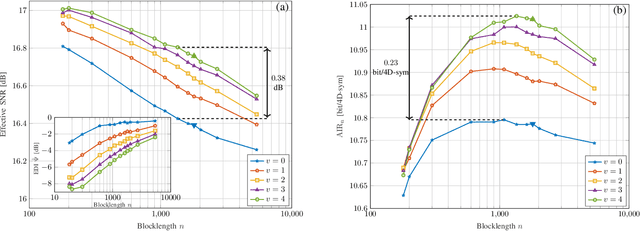
Recently, a metric called energy dispersion index (EDI) was proposed to indicate the nonlinear interference (NLI) induced by correlated symbols during optical transmission. In this paper, we propose a new shaper architecture to decrease the EDI of transmitted symbols and thus, increase the signal-to-noise ratio (SNR). We call this shaper the list-encoding constant-composition distribution matcher (L-CCDM). L-CCDM consists of an additional EDI selecting module, which is compatible with standard probabilistic amplitude shaping (PAS) architecture. Numerical results obtained from a multi-span multi-channel system show that when compared to standard CCDM with 256-ary quadrature amplitude modulation (256QAM), the proposed architecture offers an effective SNR gain of 0.35 dB, an achievable information rate gain of 0.22 bit/4D-symbol, or equivalently an 8% reach extension.

On Kurtosis-limited Enumerative Sphere Shaping for Reach Increase in Single-span Systems

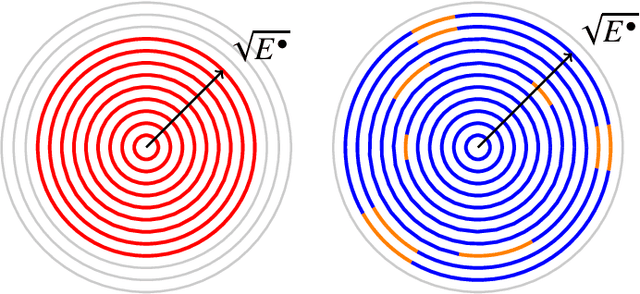
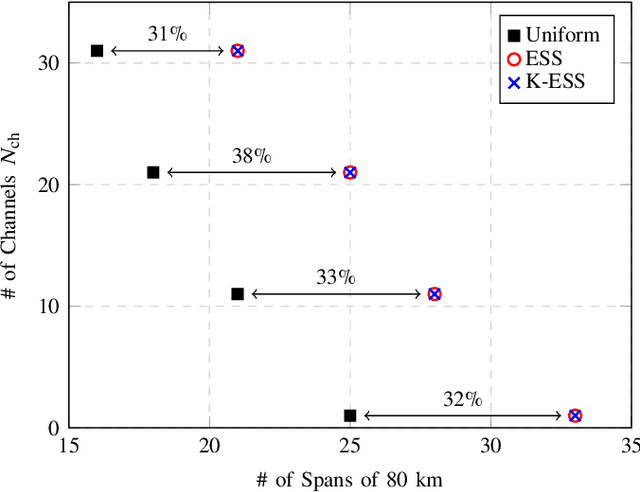
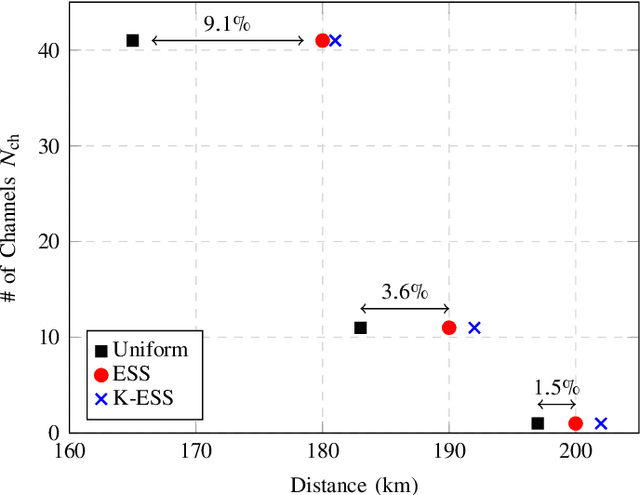
The effect of decreasing the kurtosis of channel inputs is investigated for the first time with an algorithmic shaping implementation. No significant gains in decoding performance are observed for multi-span systems, while an increase in reach is obtained for single-span transmission.

Orthogonal Time Frequency Space Modulation: A Discrete Zak Transform Approach

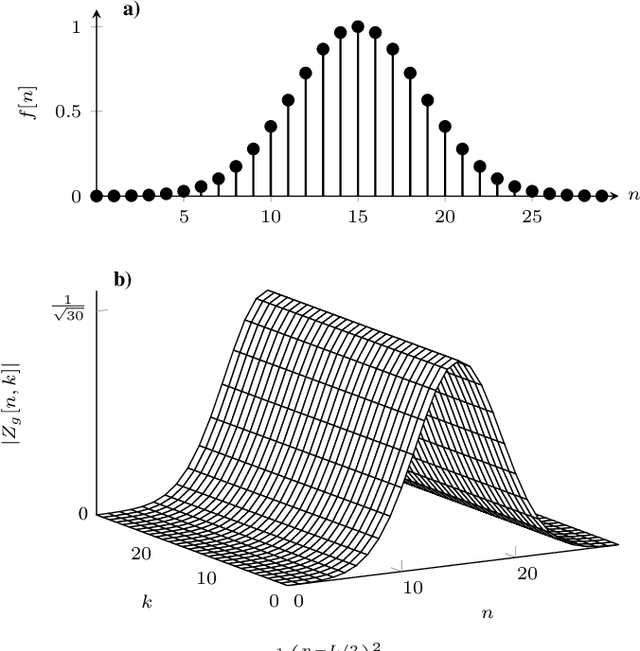
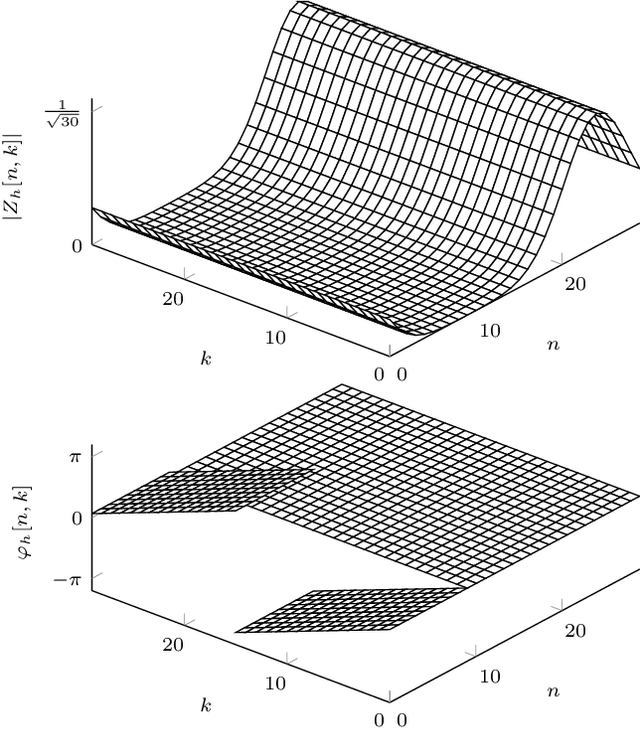
In orthogonal time frequency space (OTFS) modulation, information-carrying symbols reside in the delay-Doppler (DD) domain. By operating in the DD domain, an appealing property for communication arises: time-frequency (TF) dispersive channels encountered in high mobility environments become time-invariant. The time-invariance of the channel in the DD domain enables efficient equalizers for time-frequency dispersive channels. In this paper, we propose an OTFS system based on the discrete Zak transform. The presented formulation not only allows an efficient implementation of OTFS but also simplifies the derivation and analysis of the input-output relation of TF dispersive channel in the DD domain.

Kurtosis-limited Sphere Shaping for Nonlinear Interference Noise Reduction in Optical Channels

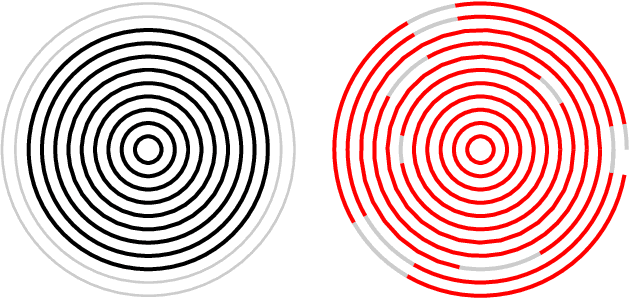
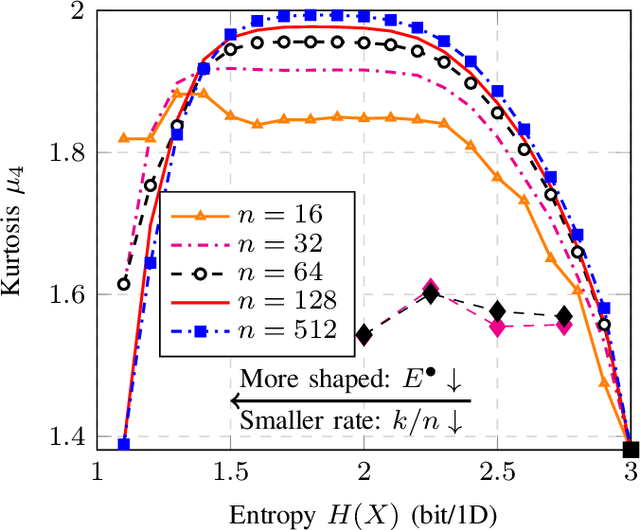
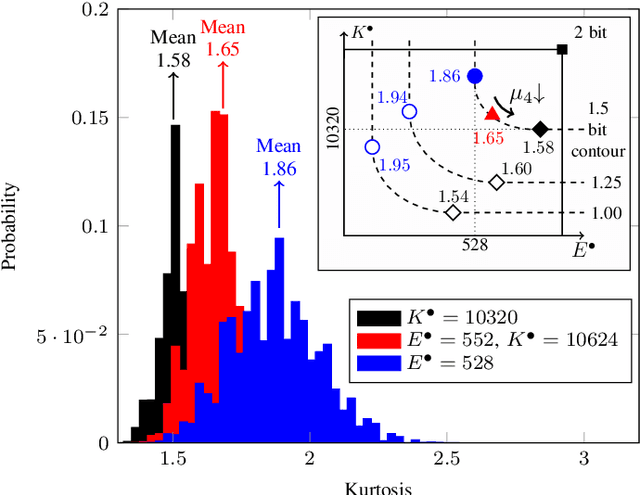
Nonlinear interference (NLI) generated during the propagation of an optical waveform through the fiber depends on the fourth order standardized moment of the channel input distribution, also known as kurtosis. Probabilistically-shaped inputs optimized for the linear Gaussian channel have a Gaussian-like distribution with high kurtosis. For optical channels, this leads to an increase in NLI power and consequently, a decrease in effective signal-to-noise ratio (SNR). In this work, we propose kurtosis-limited enumerative sphere shaping (K-ESS) as an algorithm to generate low-kurtosis shaped inputs. Numerical simulations demonstrate that with K-ESS, it is possible to increase the effective SNRs by 0.4 dB in a single-span single-channel scenario at 400 Gbit/s. K-ESS offers also a twofold decrease in frame error rate with respect to Gaussian-channel-optimal sphere shaping.

Temporal Energy Analysis of Symbol Sequences for Fiber Nonlinear Interference Modelling via Energy Dispersion Index

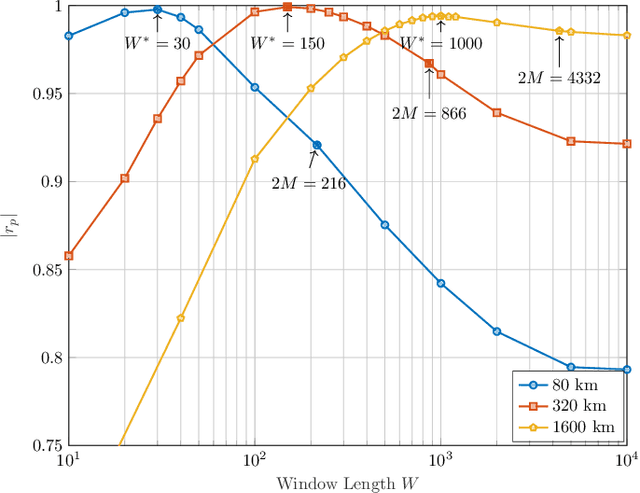
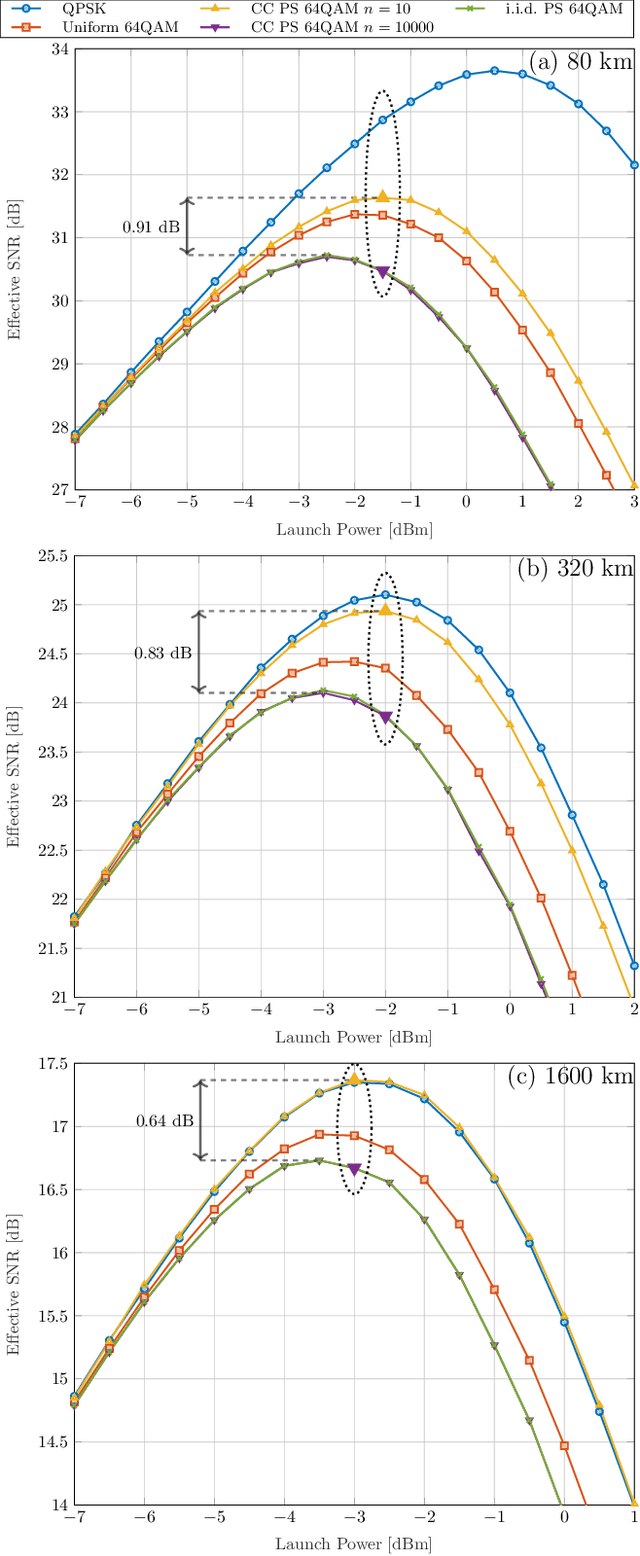
The stationary statistical properties of independent, identically distributed (i.i.d.) input symbols provide insights on the induced nonlinear interference (NLI) during fiber transmission. For example, kurtosis is known to predict the modulation format-dependent NLI. These statistical properties can be used in the design of probabilistic amplitude shaping (PAS), which is a popular scheme that relies on an amplitude shaper for increasing spectral efficiencies of fiber-optic systems. One property of certain shapers used in PAS -- including constant-composition distribution matchers -- that is often overlooked is that a time-dependency between amplitudes is introduced. This dependency results in symbols that are non-i.i.d., which have time-varying statistical properties. Somewhat surprisingly, the effective signal-to-noise ratio (SNR) in PAS has been shown to increase when the shaping blocklength decreases. This blocklength dependency of SNR has been attributed to time-varying statistical properties of the symbol sequences, in particular, to variation of the symbol energies. In this paper, we investigate the temporal energy behavior of symbol sequences, and introduce a new metric called energy dispersion index (EDI). EDI captures the time-varying statistical properties of symbol energies. Numerical results show strong correlations between EDI and effective SNR, with absolute correlation coefficients above 99% for different transmission distances.