Causal Effect Identification in LiNGAM Models with Latent Confounders

We study the generic identifiability of causal effects in linear non-Gaussian acyclic models (LiNGAM) with latent variables. We consider the problem in two main settings: When the causal graph is known a priori, and when it is unknown. In both settings, we provide a complete graphical characterization of the identifiable direct or total causal effects among observed variables. Moreover, we propose efficient algorithms to certify the graphical conditions. Finally, we propose an adaptation of the reconstruction independent component analysis (RICA) algorithm that estimates the causal effects from the observational data given the causal graph. Experimental results show the effectiveness of the proposed method in estimating the causal effects.

Parameter identification in linear non-Gaussian causal models under general confounding

Linear non-Gaussian causal models postulate that each random variable is a linear function of parent variables and non-Gaussian exogenous error terms. We study identification of the linear coefficients when such models contain latent variables. Our focus is on the commonly studied acyclic setting, where each model corresponds to a directed acyclic graph (DAG). For this case, prior literature has demonstrated that connections to overcomplete independent component analysis yield effective criteria to decide parameter identifiability in latent variable models. However, this connection is based on the assumption that the observed variables linearly depend on the latent variables. Departing from this assumption, we treat models that allow for arbitrary non-linear latent confounding. Our main result is a graphical criterion that is necessary and sufficient for deciding the generic identifiability of direct causal effects. Moreover, we provide an algorithmic implementation of the criterion with a run time that is polynomial in the number of observed variables. Finally, we report on estimation heuristics based on the identification result, explore a generalization to models with feedback loops, and provide new results on the identifiability of the causal graph.

Learning Linear Non-Gaussian Polytree Models

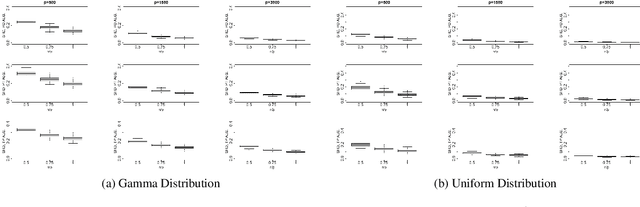
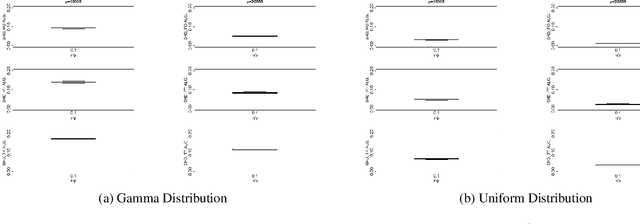
In the context of graphical causal discovery, we adapt the versatile framework of linear non-Gaussian acyclic models (LiNGAMs) to propose new algorithms to efficiently learn graphs that are polytrees. Our approach combines the Chow--Liu algorithm, which first learns the undirected tree structure, with novel schemes to orient the edges. The orientation schemes assess algebraic relations among moments of the data-generating distribution and are computationally inexpensive. We establish high-dimensional consistency results for our approach and compare different algorithmic versions in numerical experiments.