Dual Attention Driven Lumbar Magnetic Resonance Image Feature Enhancement and Automatic Diagnosis of Herniation

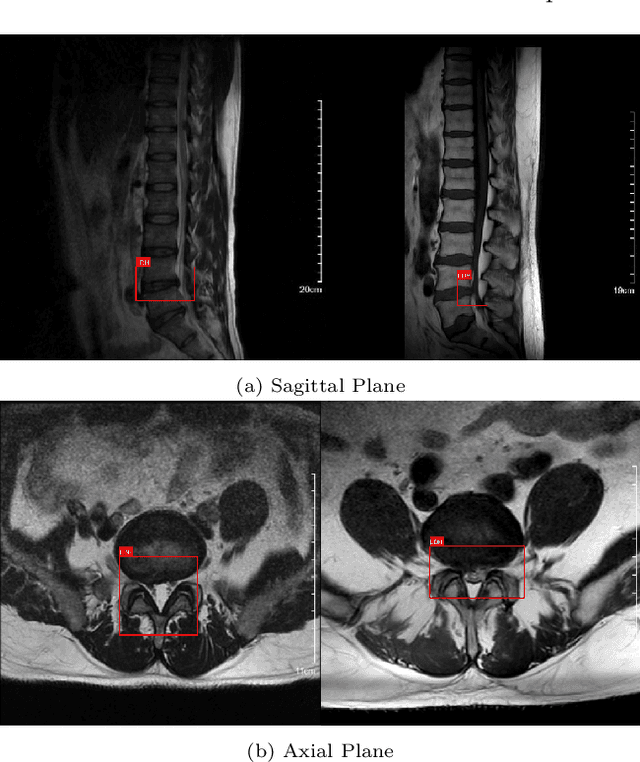
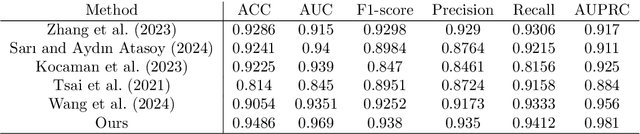
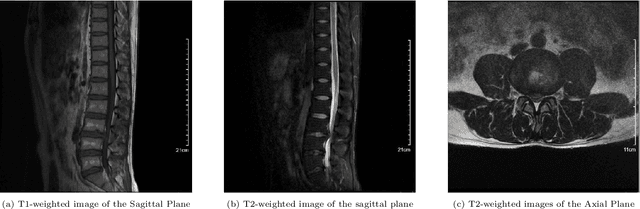
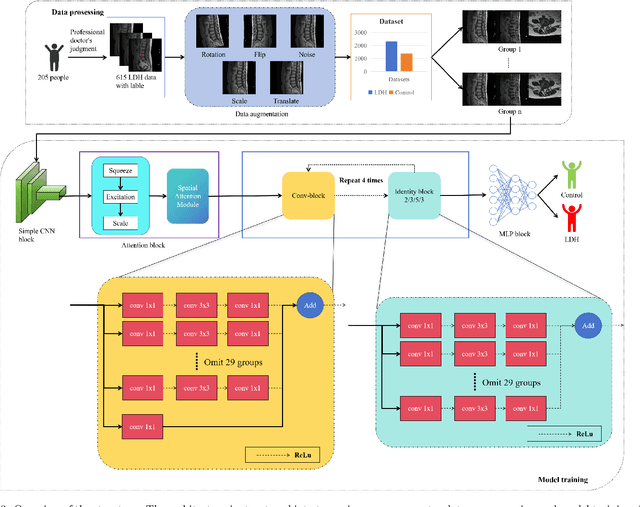
Lumbar disc herniation (LDH) is a common musculoskeletal disease that requires magnetic resonance imaging (MRI) for effective clinical management. However, the interpretation of MRI images heavily relies on the expertise of radiologists, leading to delayed diagnosis and high costs for training physicians. Therefore, this paper proposes an innovative automated LDH classification framework. To address these key issues, the framework utilizes T1-weighted and T2-weighted MRI images from 205 people. The framework extracts clinically actionable LDH features and generates standardized diagnostic outputs by leveraging data augmentation and channel and spatial attention mechanisms. These outputs can help physicians make confident and time-effective care decisions when needed. The proposed framework achieves an area under the receiver operating characteristic curve (AUC-ROC) of 0.969 and an accuracy of 0.9486 for LDH detection. The experimental results demonstrate the performance of the proposed framework. Our framework only requires a small number of datasets for training to demonstrate high diagnostic accuracy. This is expected to be a solution to enhance the LDH detection capabilities of primary hospitals.

Gotta match 'em all: Solution diversification in graph matching matched filters

We present a novel approach for finding multiple noisily embedded template graphs in a very large background graph. Our method builds upon the graph-matching-matched-filter technique proposed in Sussman et al., with the discovery of multiple diverse matchings being achieved by iteratively penalizing a suitable node-pair similarity matrix in the matched filter algorithm. In addition, we propose algorithmic speed-ups that greatly enhance the scalability of our matched-filter approach. We present theoretical justification of our methodology in the setting of correlated Erdos-Renyi graphs, showing its ability to sequentially discover multiple templates under mild model conditions. We additionally demonstrate our method's utility via extensive experiments both using simulated models and real-world dataset, include human brain connectomes and a large transactional knowledge base.

Clustered Graph Matching for Label Recovery and Graph Classification

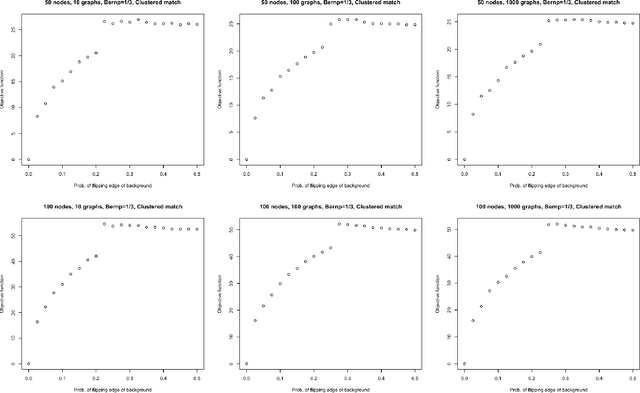
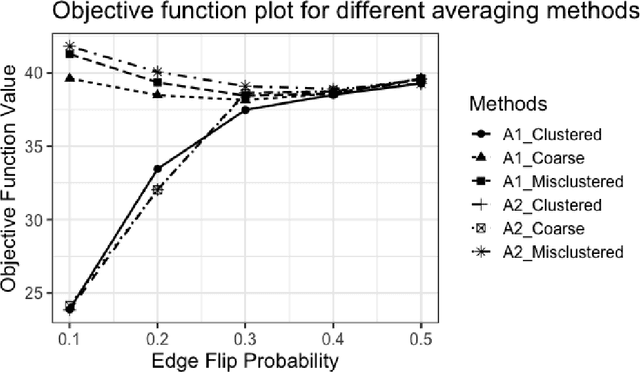
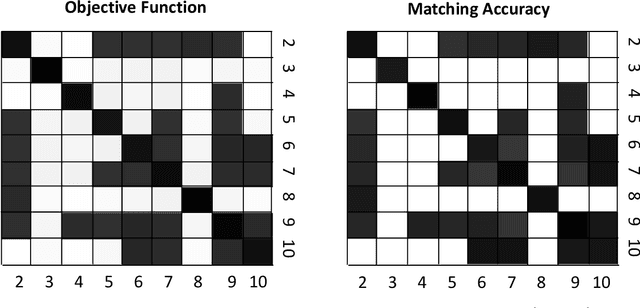
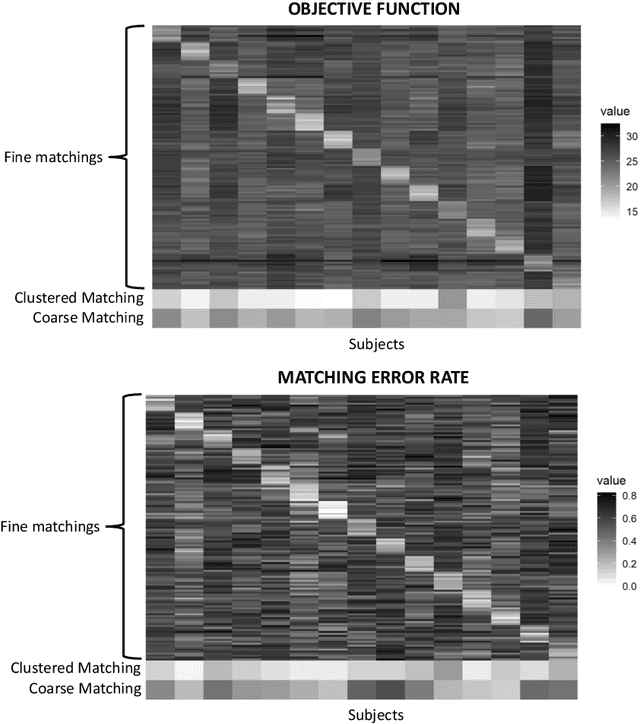
Given a collection of vertex-aligned networks and an additional label-shuffled network, we propose procedures for leveraging the signal in the vertex-aligned collection to recover the labels of the shuffled network. We consider matching the shuffled network to averages of the networks in the vertex-aligned collection at different levels of granularity. We demonstrate both in theory and practice that if the graphs come from different network classes, then clustering the networks into classes followed by matching the new graph to cluster-averages can yield higher fidelity matching performance than matching to the global average graph. Moreover, by minimizing the graph matching objective function with respect to each cluster average, this approach simultaneously classifies and recovers the vertex labels for the shuffled graph.