ExAD: An Ensemble Approach for Explanation-based Adversarial Detection

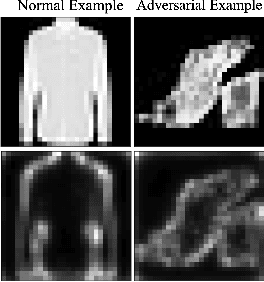
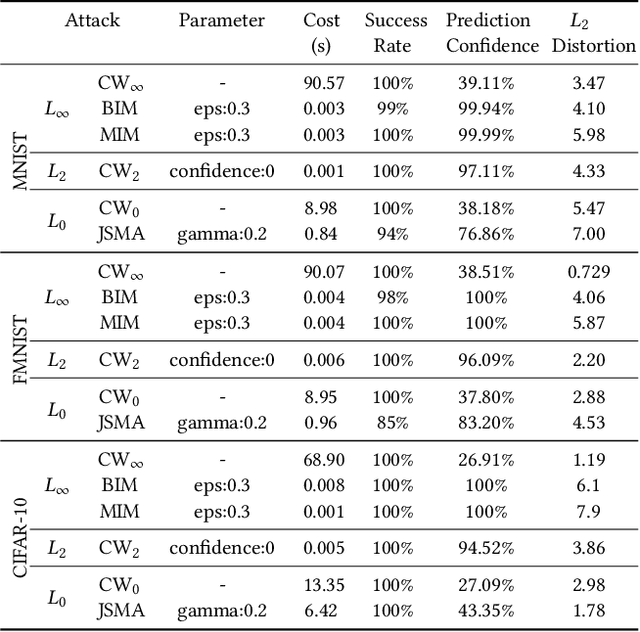
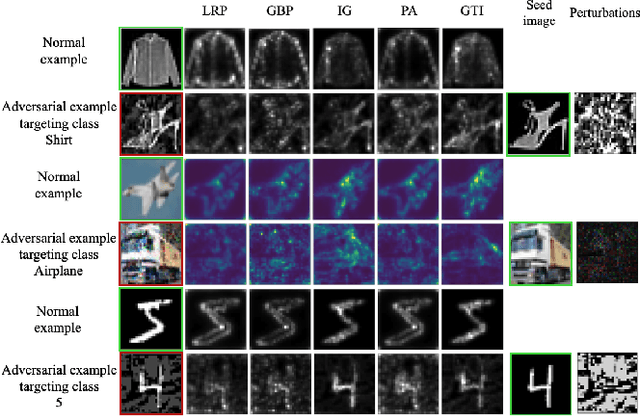
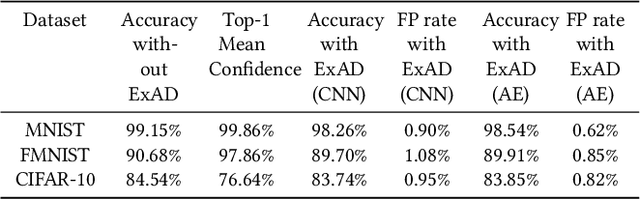
Recent research has shown Deep Neural Networks (DNNs) to be vulnerable to adversarial examples that induce desired misclassifications in the models. Such risks impede the application of machine learning in security-sensitive domains. Several defense methods have been proposed against adversarial attacks to detect adversarial examples at test time or to make machine learning models more robust. However, while existing methods are quite effective under blackbox threat model, where the attacker is not aware of the defense, they are relatively ineffective under whitebox threat model, where the attacker has full knowledge of the defense. In this paper, we propose ExAD, a framework to detect adversarial examples using an ensemble of explanation techniques. Each explanation technique in ExAD produces an explanation map identifying the relevance of input variables for the model's classification. For every class in a dataset, the system includes a detector network, corresponding to each explanation technique, which is trained to distinguish between normal and abnormal explanation maps. At test time, if the explanation map of an input is detected as abnormal by any detector model of the classified class, then we consider the input to be an adversarial example. We evaluate our approach using six state-of-the-art adversarial attacks on three image datasets. Our extensive evaluation shows that our mechanism can effectively detect these attacks under blackbox threat model with limited false-positives. Furthermore, we find that our approach achieves promising results in limiting the success rate of whitebox attacks.

Neighborhood Structure Assisted Non-negative Matrix Factorization and its Application in Unsupervised Point Anomaly Detection

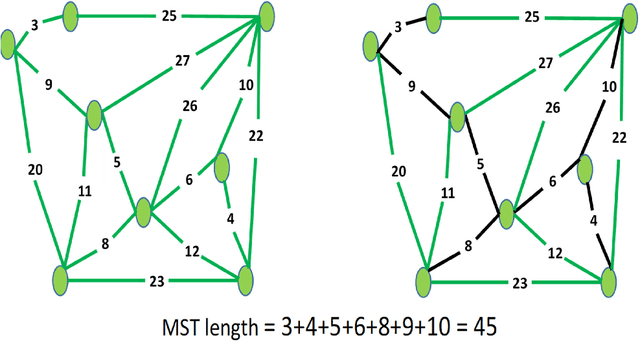
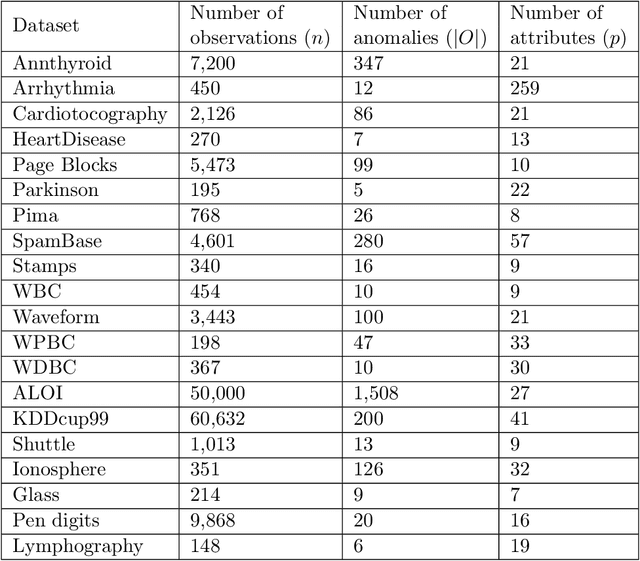
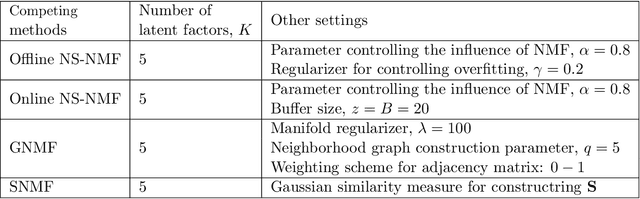
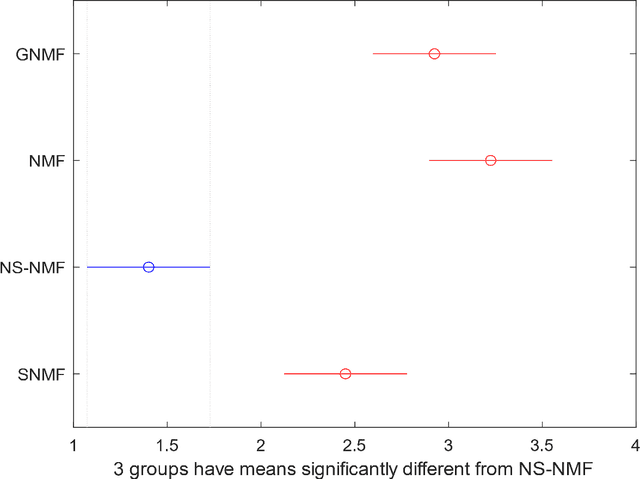
Dimensionality reduction is considered as an important step for ensuring competitive performance in unsupervised learning such as anomaly detection. Non-negative matrix factorization (NMF) is a popular and widely used method to accomplish this goal. But NMF, together with its recent, enhanced version, like graph regularized NMF or symmetric NMF, do not have the provision to include the neighborhood structure information and, as a result, may fail to provide satisfactory performance in presence of nonlinear manifold structure. To address that shortcoming, we propose to consider and incorporate the neighborhood structural similarity information within the NMF framework by modeling the data through a minimum spanning tree. What motivates our choice is the understanding that in the presence of complicated data structure, a minimum spanning tree can approximate the intrinsic distance between two data points better than a simple Euclidean distance does, and consequently, it constitutes a more reasonable basis for differentiating anomalies from the normal class data. We label the resulting method as the neighborhood structure assisted NMF. By comparing the formulation and properties of the neighborhood structure assisted NMF with other versions of NMF including graph regularized NMF and symmetric NMF, it is apparent that the inclusion of the neighborhood structure information using minimum spanning tree makes a key difference. We further devise both offline and online algorithmic versions of the proposed method. Empirical comparisons using twenty benchmark datasets as well as an industrial dataset extracted from a hydropower plant demonstrate the superiority of the neighborhood structure assisted NMF and support our claim of merit.