Wenzheng Li
Wasserstein Identity Testing
Oct 28, 2017
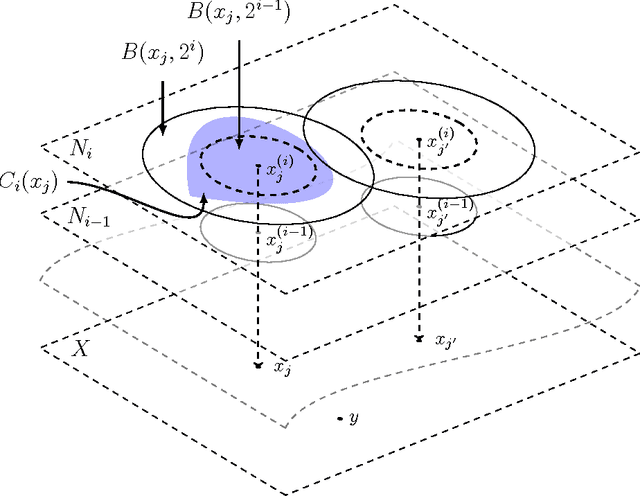
Abstract:Uniformity testing and the more general identity testing are well studied problems in distributional property testing. Most previous work focuses on testing under $L_1$-distance. However, when the support is very large or even continuous, testing under $L_1$-distance may require a huge (even infinite) number of samples. Motivated by such issues, we consider the identity testing in Wasserstein distance (a.k.a. transportation distance and earthmover distance) on a metric space (discrete or continuous). In this paper, we propose the Wasserstein identity testing problem (Identity Testing in Wasserstein distance). We obtain nearly optimal worst-case sample complexity for the problem. Moreover, for a large class of probability distributions satisfying the so-called "Doubling Condition", we provide nearly instance-optimal sample complexity.
Adapting Low-Cost Platforms for Robotics Research
May 20, 2017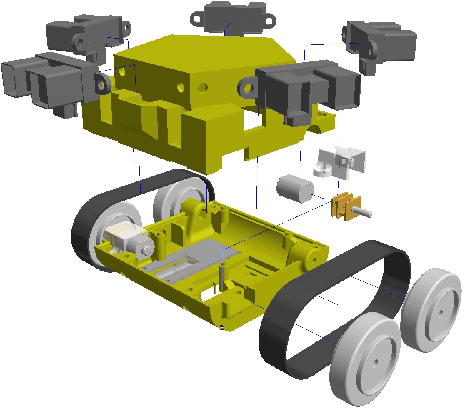
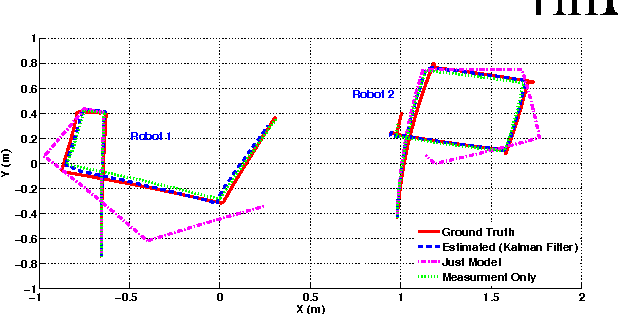
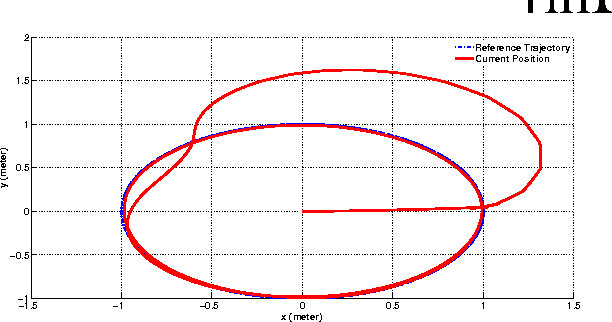
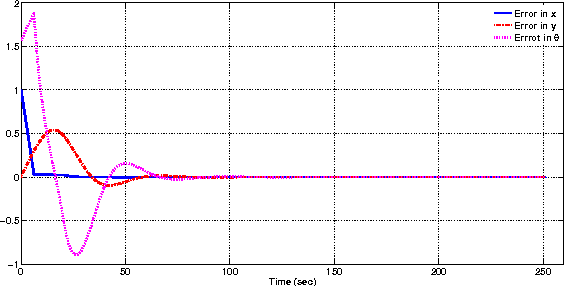
Abstract:Validation of robotics theory on real-world hardware platforms is important to prove the practical feasibility of algorithms. This paper discusses some of the lessons learned while adapting the EvoBot, a low-cost robotics platform that we designed and prototyped, for research in diverse areas in robotics. The EvoBot platform was designed to be a low cost, open source, general purpose robotics platform intended to enable testing and validation of algorithms from a wide variety of sub-fields of robotics. Throughout the paper, we outline and discuss some common failures, practical limitations and inconsistencies between theory and practice that one may encounter while adapting such low-cost platforms for robotics research. We demonstrate these aspects through four representative common robotics tasks- localization, real-time control, swarm consensus and path planning applications, performed using the EvoBots. We also propose some potential solutions to the encountered problems and try to generalize them.
* FinE-r@IROS, 2015, 7 pages, 5 figures
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge