Private Counterfactual Retrieval With Immutable Features

In a classification task, counterfactual explanations provide the minimum change needed for an input to be classified into a favorable class. We consider the problem of privately retrieving the exact closest counterfactual from a database of accepted samples while enforcing that certain features of the input sample cannot be changed, i.e., they are \emph{immutable}. An applicant (user) whose feature vector is rejected by a machine learning model wants to retrieve the sample closest to them in the database without altering a private subset of their features, which constitutes the immutable set. While doing this, the user should keep their feature vector, immutable set and the resulting counterfactual index information-theoretically private from the institution. We refer to this as immutable private counterfactual retrieval (I-PCR) problem which generalizes PCR to a more practical setting. In this paper, we propose two I-PCR schemes by leveraging techniques from private information retrieval (PIR) and characterize their communication costs. Further, we quantify the information that the user learns about the database and compare it for the proposed schemes.

Private Counterfactual Retrieval

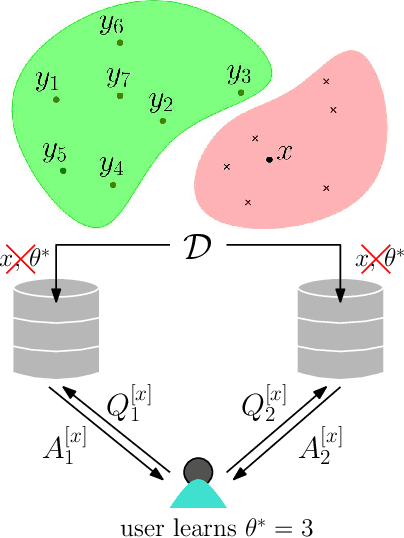
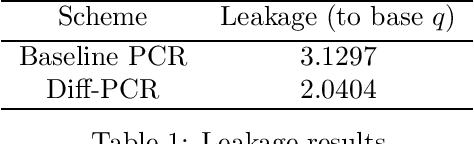
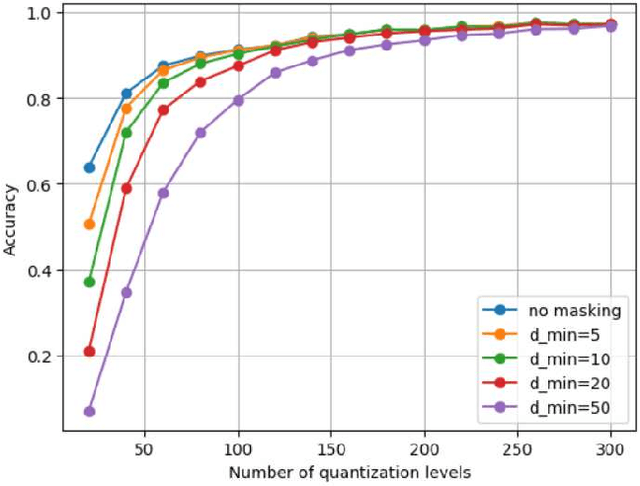
Transparency and explainability are two extremely important aspects to be considered when employing black-box machine learning models in high-stake applications. Providing counterfactual explanations is one way of catering this requirement. However, this also poses a threat to the privacy of both the institution that is providing the explanation as well as the user who is requesting it. In this work, we propose multiple schemes inspired by private information retrieval (PIR) techniques which ensure the \emph{user's privacy} when retrieving counterfactual explanations. We present a scheme which retrieves the \emph{exact} nearest neighbor counterfactual explanation from a database of accepted points while achieving perfect (information-theoretic) privacy for the user. While the scheme achieves perfect privacy for the user, some leakage on the database is inevitable which we quantify using a mutual information based metric. Furthermore, we propose strategies to reduce this leakage to achieve an advanced degree of database privacy. We extend these schemes to incorporate user's preference on transforming their attributes, so that a more actionable explanation can be received. Since our schemes rely on finite field arithmetic, we empirically validate our schemes on real datasets to understand the trade-off between the accuracy and the finite field sizes.

HetDAPAC: Distributed Attribute-Based Private Access Control with Heterogeneous Attributes

Verifying user attributes to provide fine-grained access control to databases is fundamental to an attribute-based authentication system. In such systems, either a single (central) authority verifies all attributes, or multiple independent authorities verify individual attributes distributedly to allow a user to access records stored on the servers. While a \emph{central} setup is more communication cost efficient, it causes privacy breach of \emph{all} user attributes to a central authority. Recently, Jafarpisheh et al. studied an information theoretic formulation of the \emph{distributed} multi-authority setup with $N$ non-colluding authorities, $N$ attributes and $K$ possible values for each attribute, called an $(N,K)$ distributed attribute-based private access control (DAPAC) system, where each server learns only one attribute value that it verifies, and remains oblivious to the remaining $N-1$ attributes. We show that off-loading a subset of attributes to a central server for verification improves the achievable rate from $\frac{1}{2K}$ in Jafarpisheh et al. to $\frac{1}{K+1}$ in this paper, thus \emph{almost doubling the rate} for relatively large $K$, while sacrificing the privacy of a few possibly non-sensitive attributes.

Distributed Optimization with Feasible Set Privacy

We consider the setup of a constrained optimization problem with two agents $E_1$ and $E_2$ who jointly wish to learn the optimal solution set while keeping their feasible sets $\mathcal{P}_1$ and $\mathcal{P}_2$ private from each other. The objective function $f$ is globally known and each feasible set is a collection of points from a global alphabet. We adopt a sequential symmetric private information retrieval (SPIR) framework where one of the agents (say $E_1$) privately checks in $\mathcal{P}_2$, the presence of candidate solutions of the problem constrained to $\mathcal{P}_1$ only, while learning no further information on $\mathcal{P}_2$ than the solution alone. Further, we extract an information theoretically private threshold PSI (ThPSI) protocol from our scheme and characterize its download cost. We show that, compared to privately acquiring the feasible set $\mathcal{P}_1\cap \mathcal{P}_2$ using an SPIR-based private set intersection (PSI) protocol, and finding the optimum, our scheme is better as it incurs less information leakage and less download cost than the former. Over all possible uniform mappings of $f$ to a fixed range of values, our scheme outperforms the former with a high probability.