Lazy and Fast Greedy MAP Inference for Determinantal Point Process

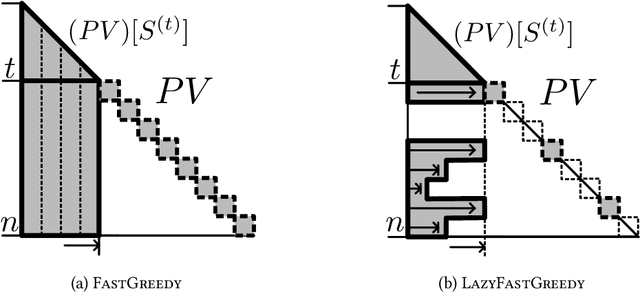
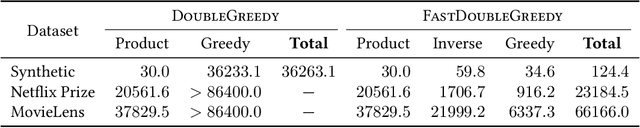
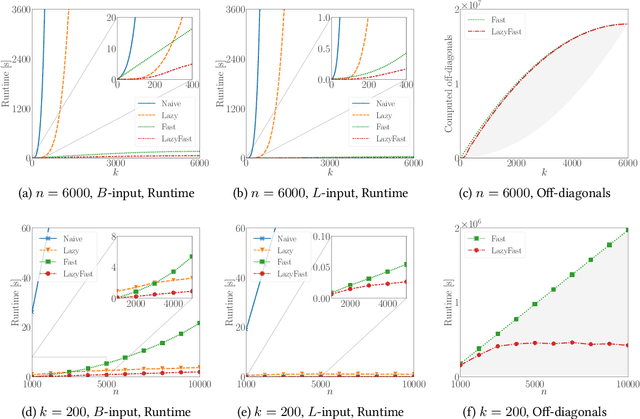
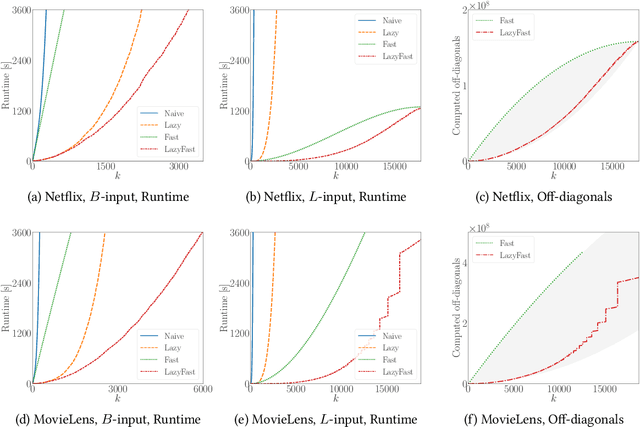
The maximum a posteriori (MAP) inference for determinantal point processes (DPPs) is crucial for selecting diverse items in many machine learning applications. Although DPP MAP inference is NP-hard, the greedy algorithm often finds high-quality solutions, and many researchers have studied its efficient implementation. One classical and practical method is the lazy greedy algorithm, which is applicable to general submodular function maximization, while a recent fast greedy algorithm based on the Cholesky factorization is more efficient for DPP MAP inference. This paper presents how to combine the ideas of "lazy" and "fast", which have been considered incompatible in the literature. Our lazy and fast greedy algorithm achieves almost the same time complexity as the current best one and runs faster in practice. The idea of "lazy + fast" is extendable to other greedy-type algorithms. We also give a fast version of the double greedy algorithm for unconstrained DPP MAP inference. Experiments validate the effectiveness of our acceleration ideas.

Learning Valuation Functions

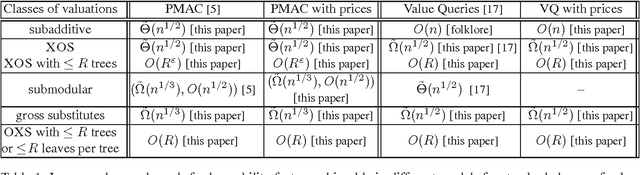
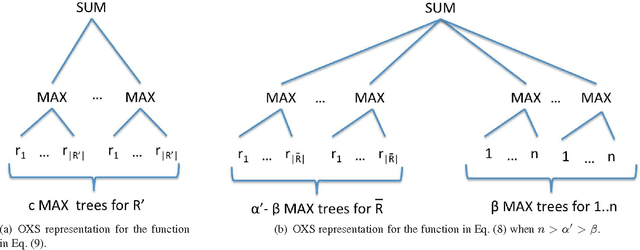
In this paper we study the approximate learnability of valuations commonly used throughout economics and game theory for the quantitative encoding of agent preferences. We provide upper and lower bounds regarding the learnability of important subclasses of valuation functions that express no-complementarities. Our main results concern their approximate learnability in the distributional learning (PAC-style) setting. We provide nearly tight lower and upper bounds of $\tilde{\Theta}(n^{1/2})$ on the approximation factor for learning XOS and subadditive valuations, both widely studied superclasses of submodular valuations. Interestingly, we show that the $\tilde{\Omega}(n^{1/2})$ lower bound can be circumvented for XOS functions of polynomial complexity; we provide an algorithm for learning the class of XOS valuations with a representation of polynomial size achieving an $O(n^{\eps})$ approximation factor in time $O(n^{1/\eps})$ for any $\eps > 0$. This highlights the importance of considering the complexity of the target function for polynomial time learning. We also provide new learning results for interesting subclasses of submodular functions. Our upper bounds for distributional learning leverage novel structural results for all these valuation classes. We show that many of these results provide new learnability results in the Goemans et al. model (SODA 2009) of approximate learning everywhere via value queries. We also introduce a new model that is more realistic in economic settings, in which the learner can set prices and observe purchase decisions at these prices rather than observing the valuation function directly. In this model, most of our upper bounds continue to hold despite the fact that the learner receives less information (both for learning in the distributional setting and with value queries), while our lower bounds naturally extend.