Integrating Vision Systems and STPA for Robust Landing and Take-Off in VTOL Aircraft

Vertical take-off and landing (VTOL) unmanned aerial vehicles (UAVs) are versatile platforms widely used in applications such as surveillance, search and rescue, and urban air mobility. Despite their potential, the critical phases of take-off and landing in uncertain and dynamic environments pose significant safety challenges due to environmental uncertainties, sensor noise, and system-level interactions. This paper presents an integrated approach combining vision-based sensor fusion with System-Theoretic Process Analysis (STPA) to enhance the safety and robustness of VTOL UAV operations during take-off and landing. By incorporating fiducial markers, such as AprilTags, into the control architecture, and performing comprehensive hazard analysis, we identify unsafe control actions and propose mitigation strategies. Key contributions include developing the control structure with vision system capable of identifying a fiducial marker, multirotor controller and corresponding unsafe control actions and mitigation strategies. The proposed solution is expected to improve the reliability and safety of VTOL UAV operations, paving the way for resilient autonomous systems.

Automated Adversary Emulation for Cyber-Physical Systems via Reinforcement Learning

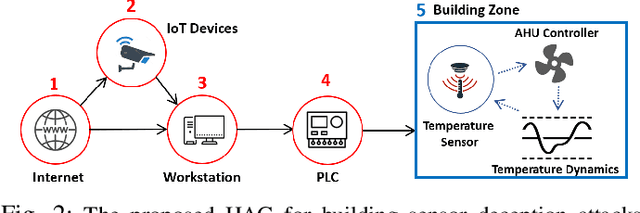
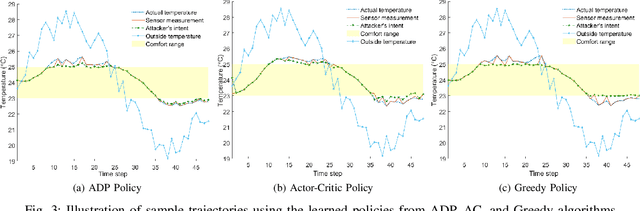
Adversary emulation is an offensive exercise that provides a comprehensive assessment of a system's resilience against cyber attacks. However, adversary emulation is typically a manual process, making it costly and hard to deploy in cyber-physical systems (CPS) with complex dynamics, vulnerabilities, and operational uncertainties. In this paper, we develop an automated, domain-aware approach to adversary emulation for CPS. We formulate a Markov Decision Process (MDP) model to determine an optimal attack sequence over a hybrid attack graph with cyber (discrete) and physical (continuous) components and related physical dynamics. We apply model-based and model-free reinforcement learning (RL) methods to solve the discrete-continuous MDP in a tractable fashion. As a baseline, we also develop a greedy attack algorithm and compare it with the RL procedures. We summarize our findings through a numerical study on sensor deception attacks in buildings to compare the performance and solution quality of the proposed algorithms.

Secure Route Planning Using Dynamic Games with Stopping States

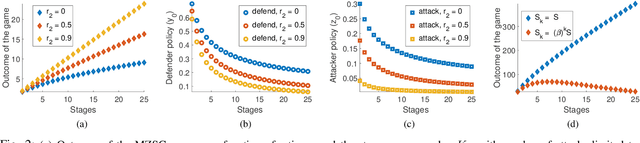
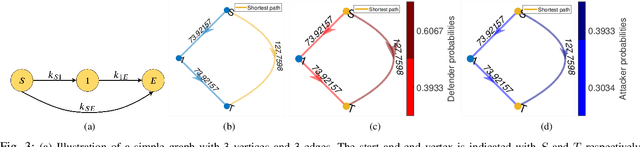
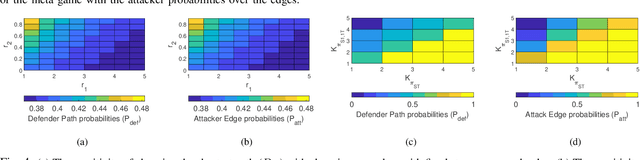
We consider the classic motion planning problem defined over a roadmap in which a vehicle seeks to find an optimal path to a given destination from a given starting location in presence of an attacker who can launch attacks on the vehicle over any edge of the roadmap. The vehicle (defender) has the capability to switch on/off a countermeasure that can detect and permanently disable the attack if it occurs concurrently. We model this problem using the framework of a zero-sum dynamic game with a stopping state being played simultaneously by the two players. We characterize the Nash equilibria of this game and provide closed form expressions for the case of two actions per player. We further provide an analytic lower bound on the value of the game and characterize conditions under which it grows sub-linearly with the number of stages. We then study the sensitivity of the Nash equilibrium to (i) the cost of using the countermeasure, (ii) the cost of motion and (iii) the benefit of disabling the attack. We then apply these results to solve the motion planning problem and compare the benefit of our approach over a competing approach based on converting the problem to a shortest path problem using the expected cost of playing the game over each edge.