Classification with neural networks with quadratic decision functions

Neural network with quadratic decision functions have been introduced as alternatives to standard neural networks with affine linear one. They are advantageous when the objects to be identified are of compact basic geometries like circles, ellipsis etc. In this paper we investigate the use of such ansatz functions for classification. In particular we test and compare the algorithm on the MNIST dataset for classification of handwritten digits and for classification of subspecies. We also show, that the implementation can be based on the neural network structure in the software Tensorflow and Keras, respectively.

Motion Detection in Diffraction Tomography by Common Circle Methods

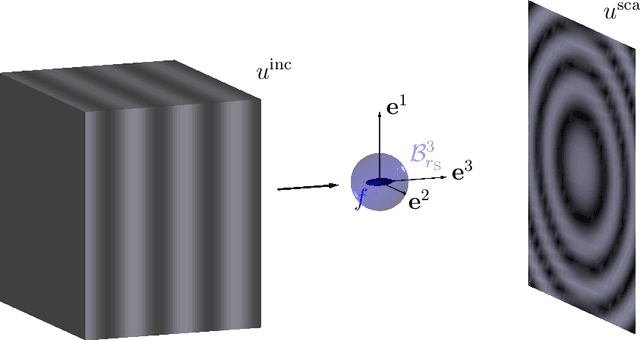
The method of common lines is a well-established reconstruction technique in cryogenic electron microscopy (cryo-EM), which can be used to extract the relative orientations of an object in tomographic projection images from different directions. In this paper, we deal with an analogous problem in optical diffraction tomography. Based on the Fourier diffraction theorem, we show that rigid motions, i.e., a map composed of rotations and translations, can be determined by detecting common circles in the Fourier-transformed data. We introduce two methods based on the idea of identifying common circles to reconstruct the object motion: While the first one is motivated by the common line approach for projection images and detects the relative orientation by the shape of the common circles in the two images, the second one assumes a smooth motion over time and calculates the angular velocity of the rotational motion from an infinitesimal version of the common circle method. Interestingly, using the stereographic projection, both methods can be reformulated as common line methods, but these lines are, in contrast to those used in cryo-EM, not confined to pass through the origin and allow for a full reconstruction of the relative orientation. Numerical proof-of-the-concept examples demonstrate the performance of our reconstruction methods.

Uncertainty-Aware Blob Detection with an Application to Integrated-Light Stellar Population Recoveries

Context. Blob detection is a common problem in astronomy. One example is in stellar population modelling, where the distribution of stellar ages and metallicities in a galaxy is inferred from observations. In this context, blobs may correspond to stars born in-situ versus those accreted from satellites, and the task of blob detection is to disentangle these components. A difficulty arises when the distributions come with significant uncertainties, as is the case for stellar population recoveries inferred from modelling spectra of unresolved stellar systems. There is currently no satisfactory method for blob detection with uncertainties. Aims. We introduce a method for uncertainty-aware blob detection developed in the context of stellar population modelling of integrated-light spectra of stellar systems. Methods. We develop theory and computational tools for an uncertainty-aware version of the classic Laplacian-of-Gaussians method for blob detection, which we call ULoG. This identifies significant blobs considering a variety of scales. As a prerequisite to apply ULoG to stellar population modelling, we introduce a method for efficient computation of uncertainties for spectral modelling. This method is based on the truncated Singular Value Decomposition and Markov Chain Monte Carlo sampling (SVD-MCMC). Results. We apply the methods to data of the star cluster M54. We show that the SVD-MCMC inferences match those from standard MCMC, but are a factor 5-10 faster to compute. We apply ULoG to the inferred M54 age/metallicity distributions, identifying between 2 or 3 significant, distinct populations amongst its stars.

On a spatial-temporal decomposition of the optical flow

In this paper we present a decomposition algorithm for computation of the spatial-temporal optical flow of a dynamic image sequence. We consider several applications, such as the extraction of temporal motion features and motion detection in dynamic sequences under varying illumination conditions, such as they appear for instance in psychological flickering experiments. For the numerical implementation we are solving an integro-differential equation by a fixed point iteration. For comparison purposes we use a standard time dependent optical flow algorithm, which in contrast to our method, constitutes in solving a spatial-temporal differential equation.

Dynamical optical flow of saliency maps for predicting visual attention

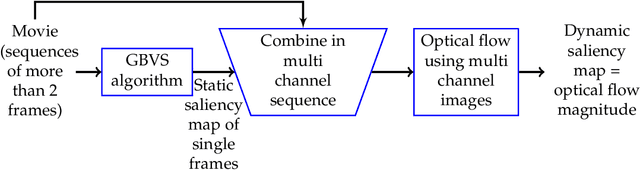
Saliency maps are used to understand human attention and visual fixation. However, while very well established for static images, there is no general agreement on how to compute a saliency map of dynamic scenes. In this paper we propose a mathematically rigorous approach to this prob- lem, including static saliency maps of each video frame for the calculation of the optical flow. Taking into account static saliency maps for calculating the optical flow allows for overcoming the aperture problem. Our ap- proach is able to explain human fixation behavior in situations which pose challenges to standard approaches, such as when a fixated object disappears behind an occlusion and reappears after several frames. In addition, we quantitatively compare our model against alternative solutions using a large eye tracking data set. Together, our results suggest that assessing optical flow information across a series of saliency maps gives a highly accurate and useful account of human overt attention in dynamic scenes.

Optical Flow on Evolving Sphere-Like Surfaces

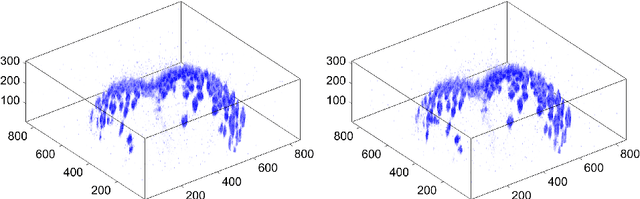
In this work we consider optical flow on evolving Riemannian 2-manifolds which can be parametrised from the 2-sphere. Our main motivation is to estimate cell motion in time-lapse volumetric microscopy images depicting fluorescently labelled cells of a live zebrafish embryo. We exploit the fact that the recorded cells float on the surface of the embryo and allow for the extraction of an image sequence together with a sphere-like surface. We solve the resulting variational problem by means of a Galerkin method based on vector spherical harmonics and present numerical results computed from the aforementioned microscopy data.

Optical Flow on Evolving Surfaces with Space and Time Regularisation

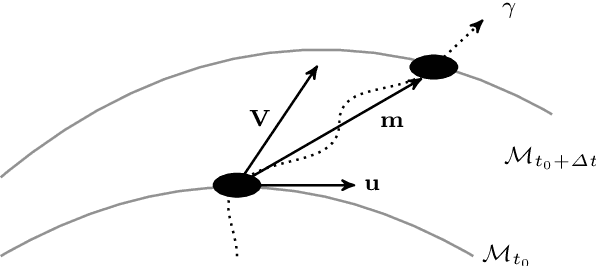
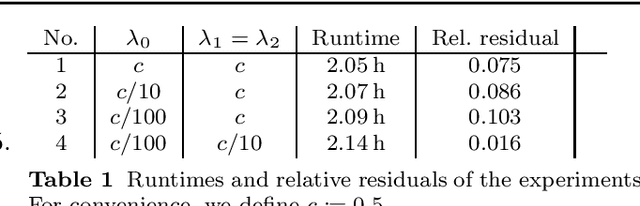
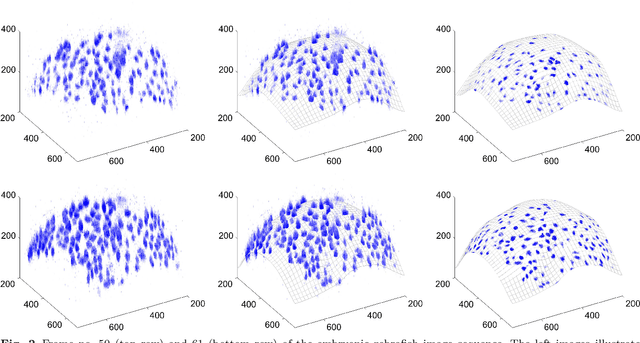
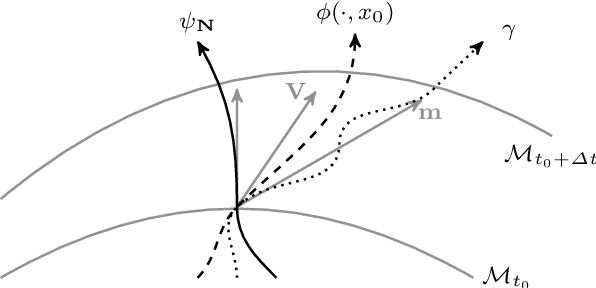
We extend the concept of optical flow with spatiotemporal regularisation to a dynamic non-Euclidean setting. Optical flow is traditionally computed from a sequence of flat images. The purpose of this paper is to introduce variational motion estimation for images that are defined on an evolving surface. Volumetric microscopy images depicting a live zebrafish embryo serve as both biological motivation and test data.

Decomposition of Optical Flow on the Sphere

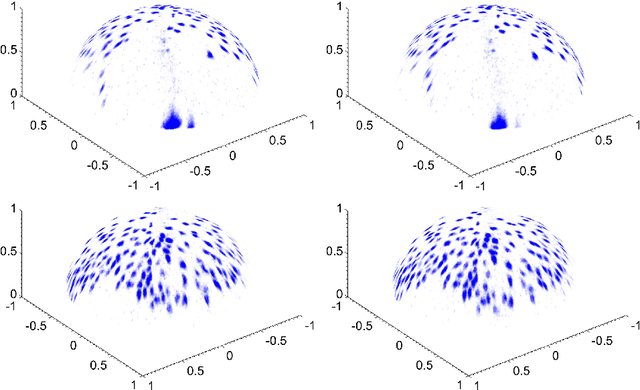
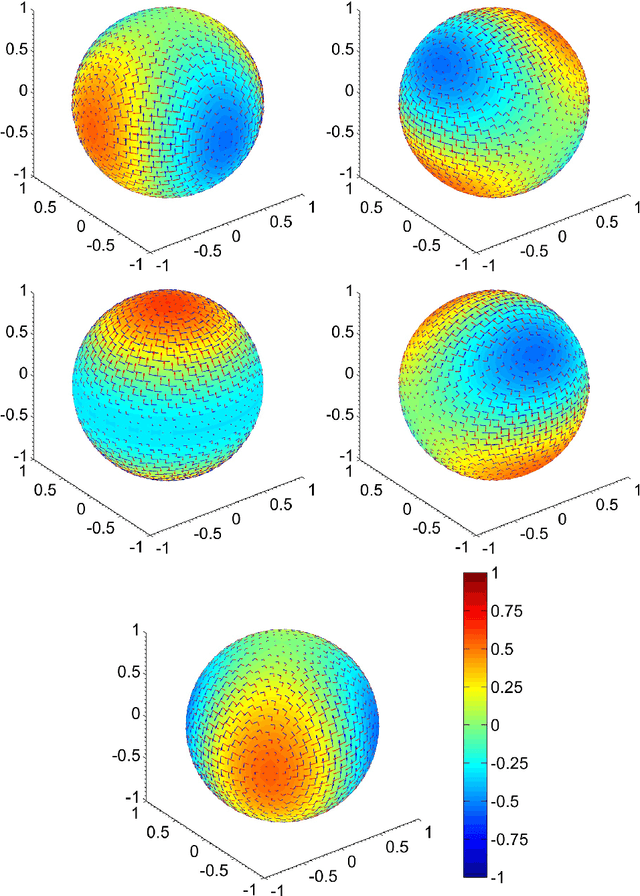
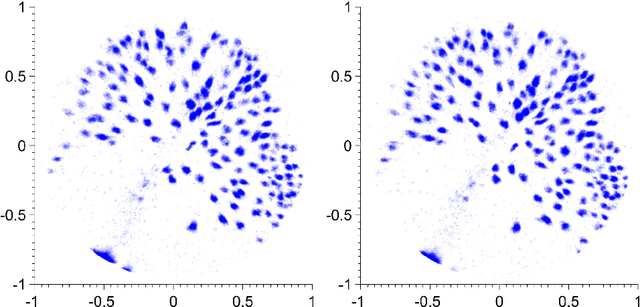
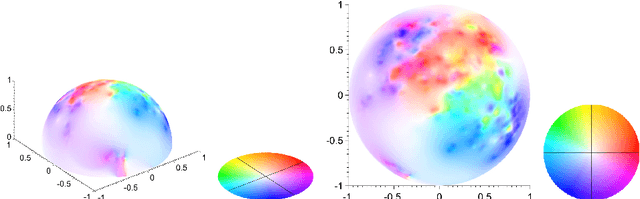
We propose a number of variational regularisation methods for the estimation and decomposition of motion fields on the $2$-sphere. While motion estimation is based on the optical flow equation, the presented decomposition models are motivated by recent trends in image analysis. In particular we treat $u+v$ decomposition as well as hierarchical decomposition. Helmholtz decomposition of motion fields is obtained as a natural by-product of the chosen numerical method based on vector spherical harmonics. All models are tested on time-lapse microscopy data depicting fluorescently labelled endodermal cells of a zebrafish embryo.

Optical Flow on Evolving Surfaces with an Application to the Analysis of 4D Microscopy Data

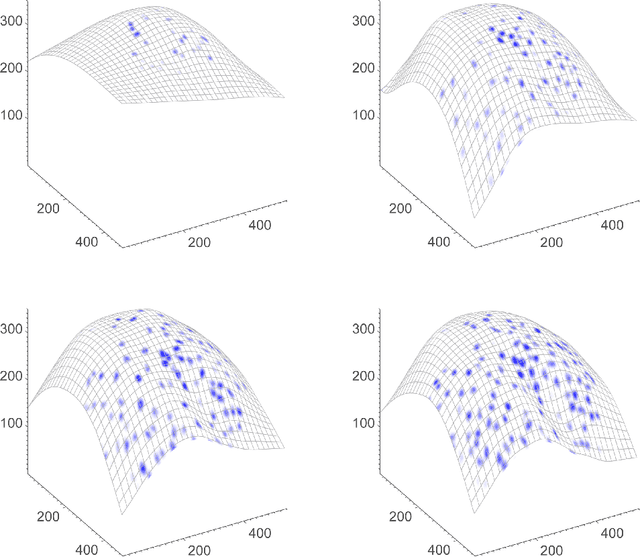
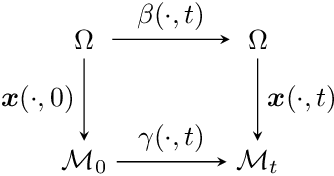
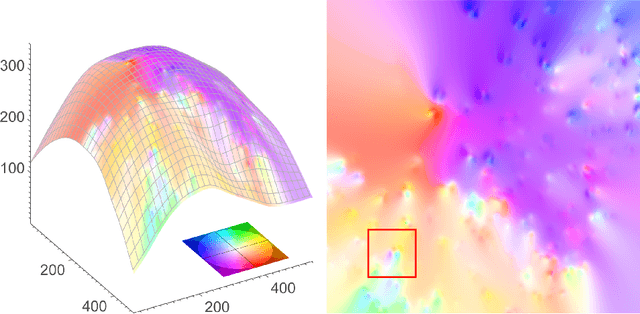
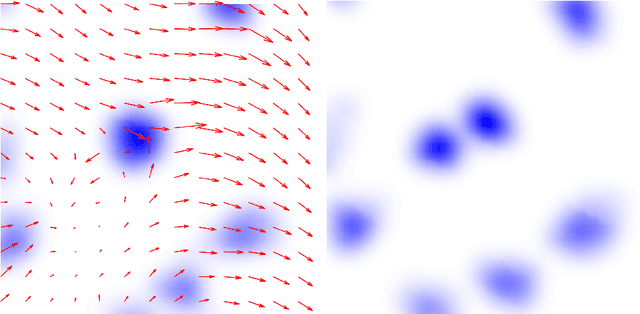
We extend the concept of optical flow to a dynamic non-Euclidean setting. Optical flow is traditionally computed from a sequence of flat images. It is the purpose of this paper to introduce variational motion estimation for images that are defined on an evolving surface. Volumetric microscopy images depicting a live zebrafish embryo serve as both biological motivation and test data.