Lukas F. Lang
A Numerical Framework for Efficient Motion Estimation on Evolving Sphere-Like Surfaces based on Brightness and Mass Conservation Laws
May 02, 2018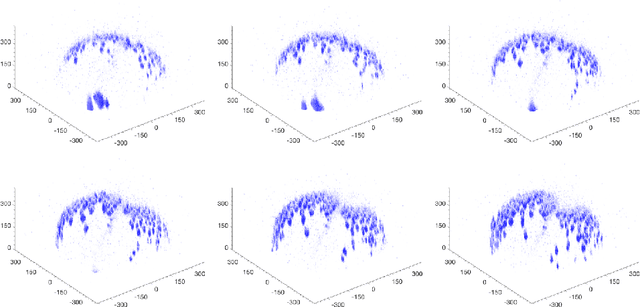
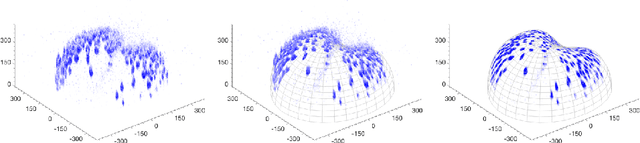
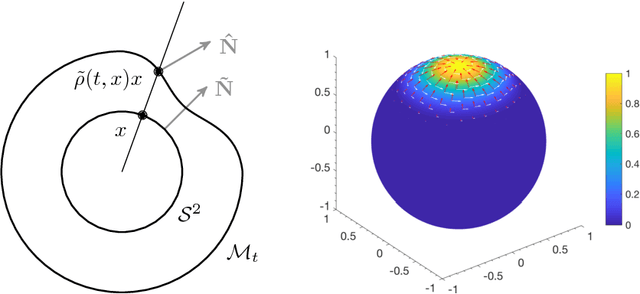
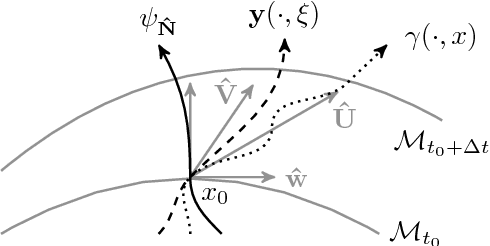
Abstract:In this work we consider brightness and mass conservation laws for motion estimation on evolving Riemannian 2-manifolds that allow for a radial parametrisation from the 2-sphere. While conservation of brightness constitutes the foundation for optical flow methods and has been generalised to said scenario, we formulate in this article the principle of mass conservation for time-varying surfaces which are embedded in Euclidean 3-space and derive a generalised continuity equation. The main motivation for this work is efficient cell motion estimation in time-lapse (4D) volumetric fluorescence microscopy images of a living zebrafish embryo. Increasing spatial and temporal resolution of modern microscopes require efficient analysis of such data. With this application in mind we address this need and follow an emerging paradigm in this field: dimensional reduction. In light of the ill-posedness of considered conservation laws we employ Tikhonov regularisation and propose the use of spatially varying regularisation functionals that recover motion only in regions with cells. For the efficient numerical solution we devise a Galerkin method based on compactly supported (tangent) vectorial basis functions. Furthermore, for the fast and accurate estimation of the evolving sphere-like surface from scattered data we utilise surface interpolation with spatio-temporal regularisation. We present numerical results based on aforementioned zebrafish microscopy data featuring fluorescently labelled cells.
A Dynamic Programming Solution to Bounded Dejittering Problems
Mar 27, 2017
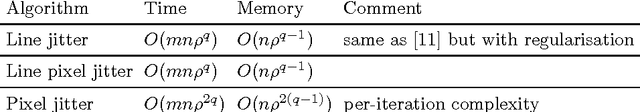


Abstract:We propose a dynamic programming solution to image dejittering problems with bounded displacements and obtain efficient algorithms for the removal of line jitter, line pixel jitter, and pixel jitter.
Optical Flow on Evolving Sphere-Like Surfaces
Jun 10, 2015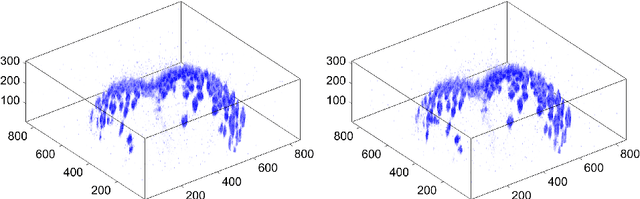



Abstract:In this work we consider optical flow on evolving Riemannian 2-manifolds which can be parametrised from the 2-sphere. Our main motivation is to estimate cell motion in time-lapse volumetric microscopy images depicting fluorescently labelled cells of a live zebrafish embryo. We exploit the fact that the recorded cells float on the surface of the embryo and allow for the extraction of an image sequence together with a sphere-like surface. We solve the resulting variational problem by means of a Galerkin method based on vector spherical harmonics and present numerical results computed from the aforementioned microscopy data.
Optical Flow on Evolving Surfaces with Space and Time Regularisation
Jun 25, 2014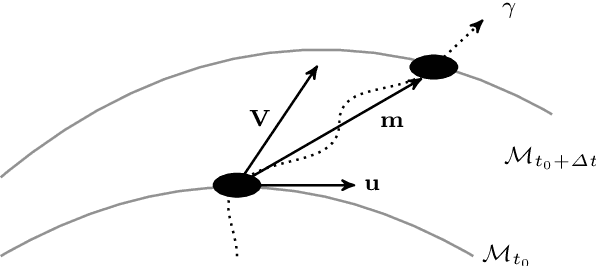
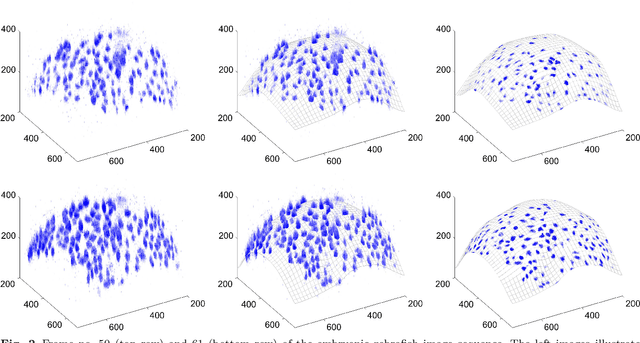
Abstract:We extend the concept of optical flow with spatiotemporal regularisation to a dynamic non-Euclidean setting. Optical flow is traditionally computed from a sequence of flat images. The purpose of this paper is to introduce variational motion estimation for images that are defined on an evolving surface. Volumetric microscopy images depicting a live zebrafish embryo serve as both biological motivation and test data.
Decomposition of Optical Flow on the Sphere
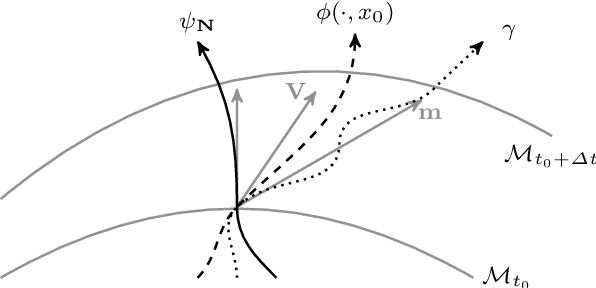
Mar 04, 2014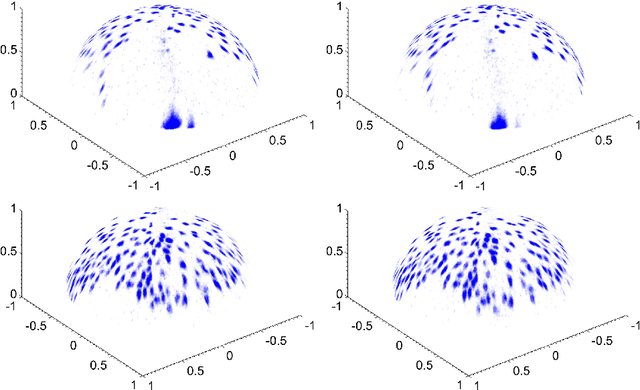
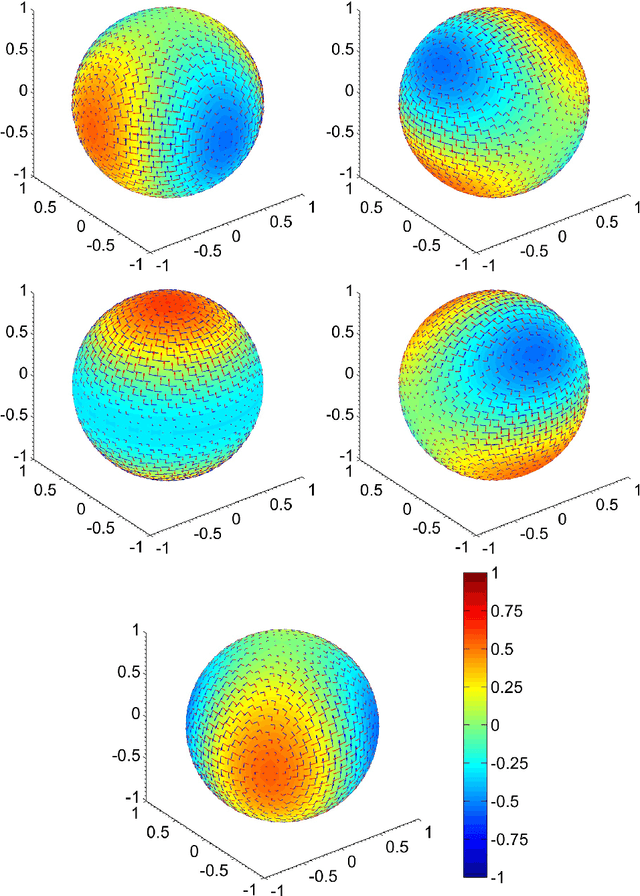
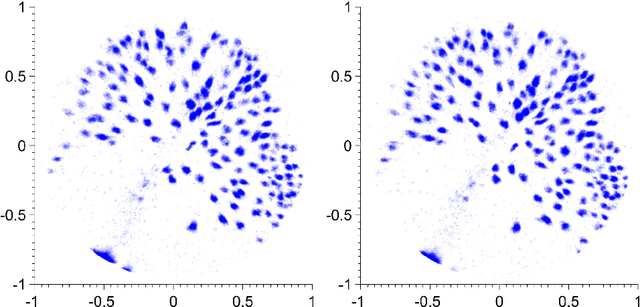
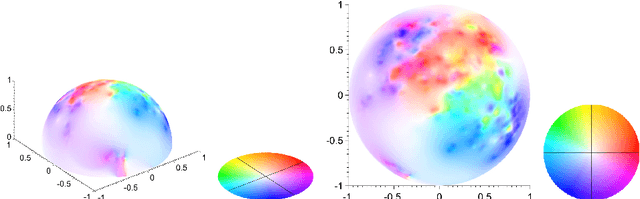
Abstract:We propose a number of variational regularisation methods for the estimation and decomposition of motion fields on the $2$-sphere. While motion estimation is based on the optical flow equation, the presented decomposition models are motivated by recent trends in image analysis. In particular we treat $u+v$ decomposition as well as hierarchical decomposition. Helmholtz decomposition of motion fields is obtained as a natural by-product of the chosen numerical method based on vector spherical harmonics. All models are tested on time-lapse microscopy data depicting fluorescently labelled endodermal cells of a zebrafish embryo.
Optical Flow on Evolving Surfaces with an Application to the Analysis of 4D Microscopy Data
May 21, 2013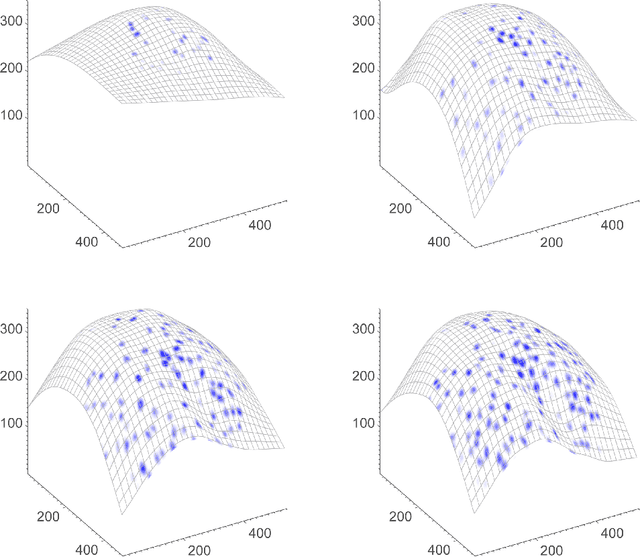
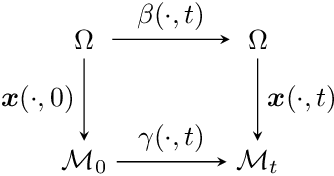
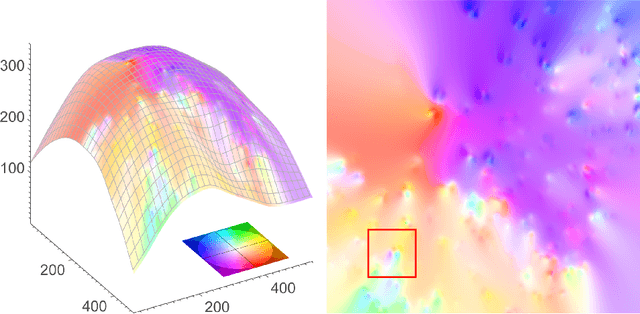
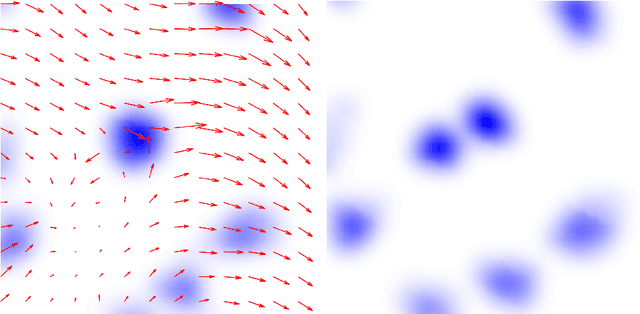
Abstract:We extend the concept of optical flow to a dynamic non-Euclidean setting. Optical flow is traditionally computed from a sequence of flat images. It is the purpose of this paper to introduce variational motion estimation for images that are defined on an evolving surface. Volumetric microscopy images depicting a live zebrafish embryo serve as both biological motivation and test data.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge