Mauricio Cerda
INRIA Lorraine - LORIA
A Class of Topological Pseudodistances for Fast Comparison of Persistence Diagrams
Feb 22, 2024Abstract:Persistence diagrams (PD)s play a central role in topological data analysis, and are used in an ever increasing variety of applications. The comparison of PD data requires computing comparison metrics among large sets of PDs, with metrics which are accurate, theoretically sound, and fast to compute. Especially for denser multi-dimensional PDs, such comparison metrics are lacking. While on the one hand, Wasserstein-type distances have high accuracy and theoretical guarantees, they incur high computational cost. On the other hand, distances between vectorizations such as Persistence Statistics (PS)s have lower computational cost, but lack the accuracy guarantees and in general they are not guaranteed to distinguish PDs (i.e. the two PS vectors of different PDs may be equal). In this work we introduce a class of pseudodistances called Extended Topological Pseudodistances (ETD)s, which have tunable complexity, and can approximate Sliced and classical Wasserstein distances at the high-complexity extreme, while being computationally lighter and close to Persistence Statistics at the lower complexity extreme, and thus allow users to interpolate between the two metrics. We build theoretical comparisons to show how to fit our new distances at an intermediate level between persistence vectorizations and Wasserstein distances. We also experimentally verify that ETDs outperform PSs in terms of accuracy and outperform Wasserstein and Sliced Wasserstein distances in terms of computational complexity.
RaViTT: Random Vision Transformer Tokens
Jun 19, 2023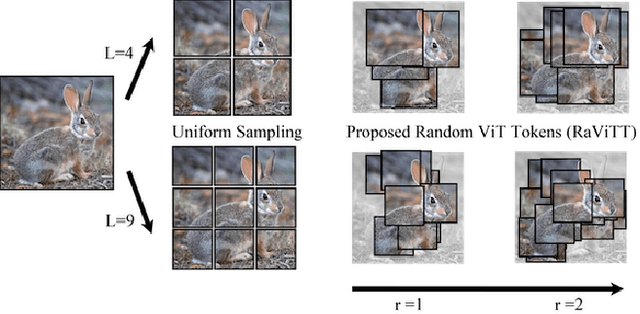
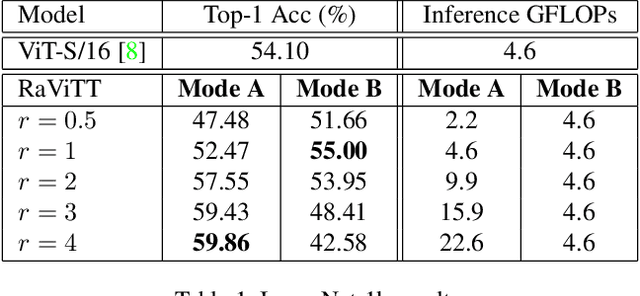
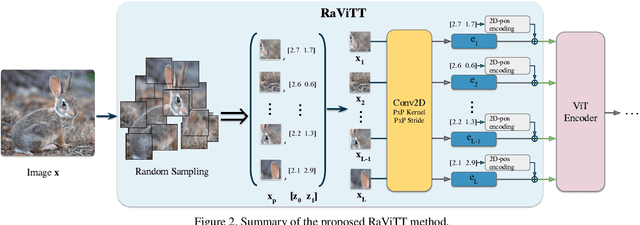
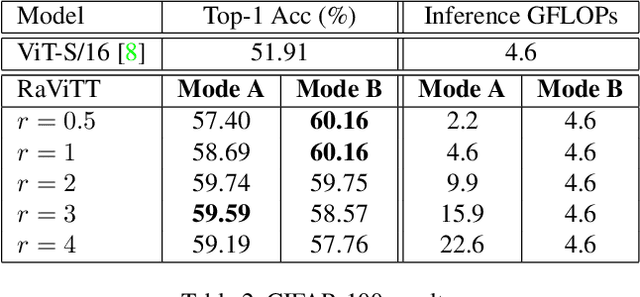
Abstract:Vision Transformers (ViTs) have successfully been applied to image classification problems where large annotated datasets are available. On the other hand, when fewer annotations are available, such as in biomedical applications, image augmentation techniques like introducing image variations or combinations have been proposed. However, regarding ViT patch sampling, less has been explored outside grid-based strategies. In this work, we propose Random Vision Transformer Tokens (RaViTT), a random patch sampling strategy that can be incorporated into existing ViTs. We experimentally evaluated RaViTT for image classification, comparing it with a baseline ViT and state-of-the-art (SOTA) augmentation techniques in 4 datasets, including ImageNet-1k and CIFAR-100. Results show that RaViTT increases the accuracy of the baseline in all datasets and outperforms the SOTA augmentation techniques in 3 out of 4 datasets by a significant margin +1.23% to +4.32%. Interestingly, RaViTT accuracy improvements can be achieved even with fewer tokens, thus reducing the computational load of any ViT model for a given accuracy value.
A Topological Data Analysis Based Classifier
Nov 10, 2021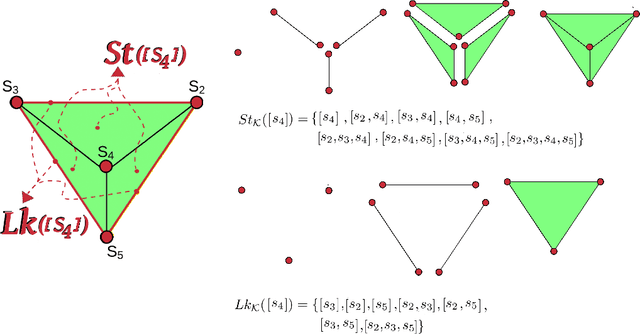
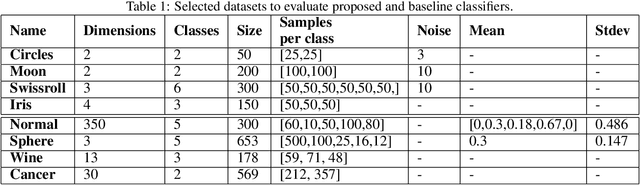
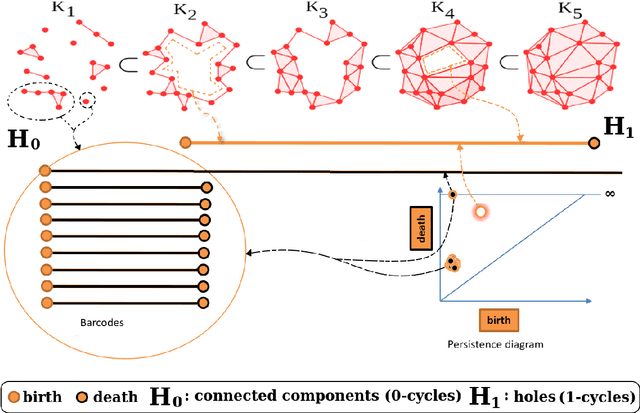

Abstract:Topological Data Analysis (TDA) is an emergent field that aims to discover topological information hidden in a dataset. TDA tools have been commonly used to create filters and topological descriptors to improve Machine Learning (ML) methods. This paper proposes an algorithm that applies TDA directly to multi-class classification problems, without any further ML stage, showing advantages for imbalanced datasets. The proposed algorithm builds a filtered simplicial complex on the dataset. Persistent Homology (PH) is applied to guide the selection of a sub-complex where unlabeled points obtain the label with the majority of votes from labeled neighboring points. We select 8 datasets with different dimensions, degrees of class overlap and imbalanced samples per class. On average, the proposed TDABC method was better than KNN and weighted-KNN. It behaves competitively with Local SVM and Random Forest baseline classifiers in balanced datasets, and it outperforms all baseline methods classifying entangled and minority classes.
Classification based on Topological Data Analysis
Feb 07, 2021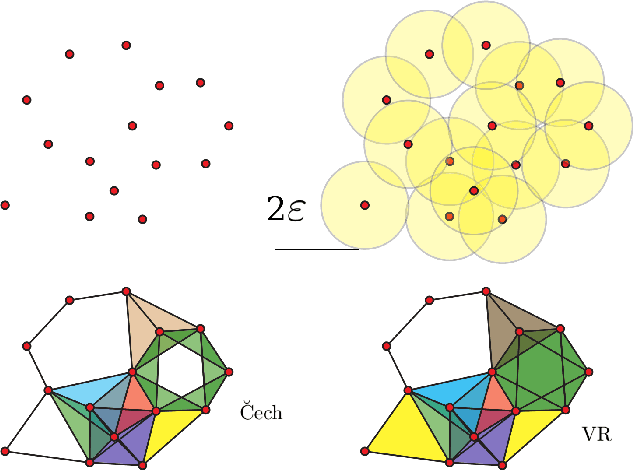
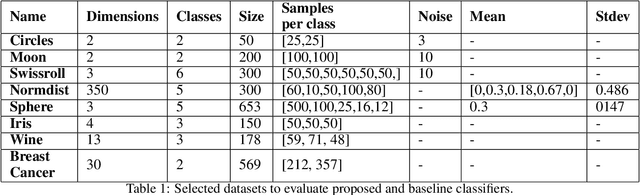
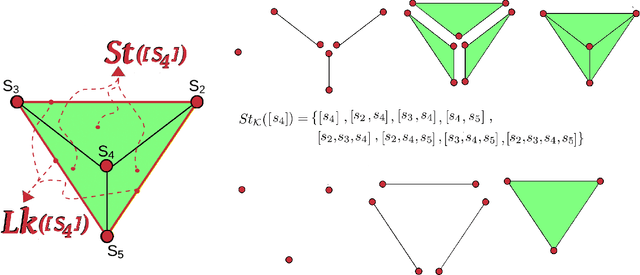

Abstract:Topological Data Analysis (TDA) is an emergent field that aims to discover topological information hidden in a dataset. TDA tools have been commonly used to create filters and topological descriptors to improve Machine Learning (ML) methods. This paper proposes an algorithm that applies TDA directly to multi-class classification problems, even imbalanced datasets, without any further ML stage. The proposed algorithm built a filtered simplicial complex on the dataset. Persistent homology is then applied to guide choosing a sub-complex where unlabeled points obtain the label with most votes from labeled neighboring points. To assess the proposed method, 8 datasets were selected with several degrees of class entanglement, variability on the samples per class, and dimensionality. On average, the proposed TDABC method was capable of overcoming baseline classifiers (wk-NN and k-NN) in each of the computed metrics, especially on classifying entangled and minority classes.
Bio-inspired speed detection and discrimination
Nov 23, 2009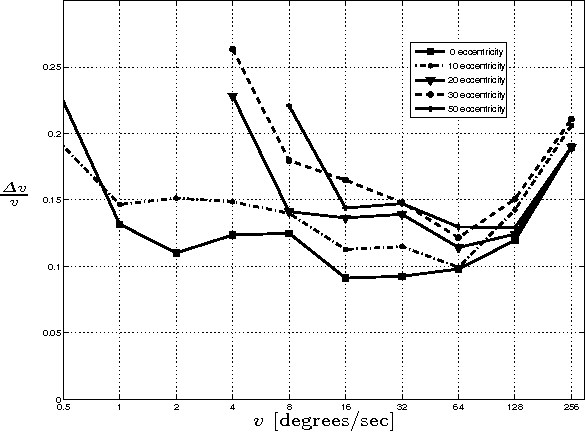
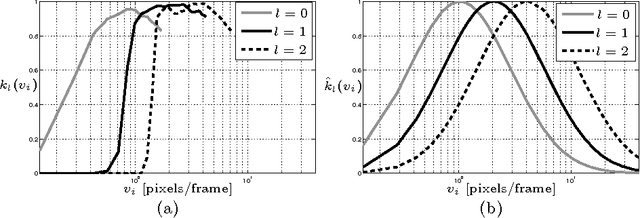
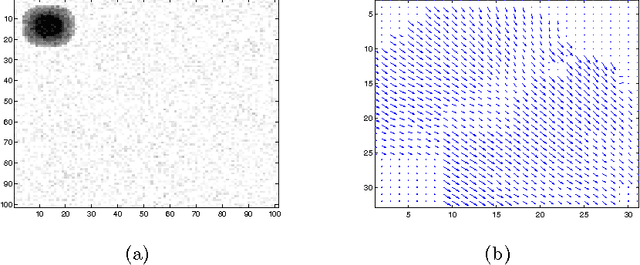
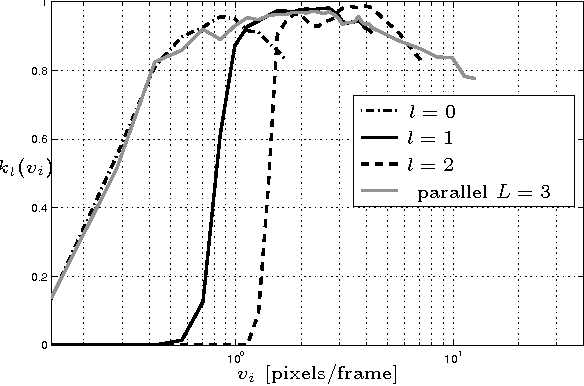
Abstract:In the field of computer vision, a crucial task is the detection of motion (also called optical flow extraction). This operation allows analysis such as 3D reconstruction, feature tracking, time-to-collision and novelty detection among others. Most of the optical flow extraction techniques work within a finite range of speeds. Usually, the range of detection is extended towards higher speeds by combining some multiscale information in a serial architecture. This serial multi-scale approach suffers from the problem of error propagation related to the number of scales used in the algorithm. On the other hand, biological experiments show that human motion perception seems to follow a parallel multiscale scheme. In this work we present a bio-inspired parallel architecture to perform detection of motion, providing a wide range of operation and avoiding error propagation associated with the serial architecture. To test our algorithm, we perform relative error comparisons between both classical and proposed techniques, showing that the parallel architecture is able to achieve motion detection with results similar to the serial approach.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge