Detecting Causality in the Frequency Domain with Cross-Mapping Coherence

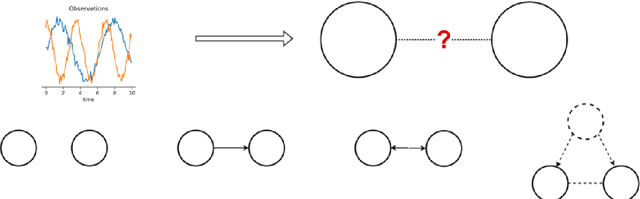
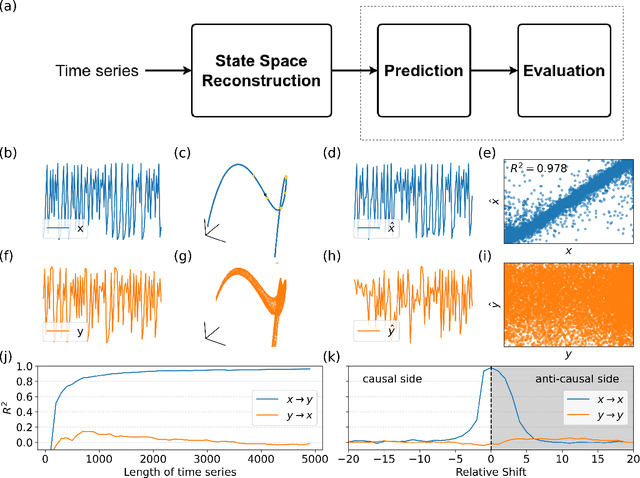
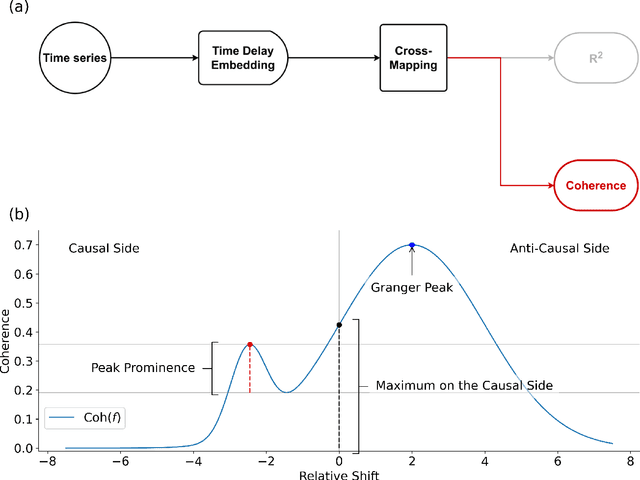
Understanding causal relationships within a system is crucial for uncovering its underlying mechanisms. Causal discovery methods, which facilitate the construction of such models from time-series data, hold the potential to significantly advance scientific and engineering fields. This study introduces the Cross-Mapping Coherence (CMC) method, designed to reveal causal connections in the frequency domain between time series. CMC builds upon nonlinear state-space reconstruction and extends the Convergent Cross-Mapping algorithm to the frequency domain by utilizing coherence metrics for evaluation. We tested the Cross-Mapping Coherence method using simulations of logistic maps, Lorenz systems, Kuramoto oscillators, and the Wilson-Cowan model of the visual cortex. CMC accurately identified the direction of causal connections in all simulated scenarios. When applied to the Wilson-Cowan model, CMC yielded consistent results similar to spectral Granger causality. Furthermore, CMC exhibits high sensitivity in detecting weak connections, demonstrates sample efficiency, and maintains robustness in the presence of noise. In conclusion, the capability to determine directed causal influences across different frequency bands allows CMC to provide valuable insights into the dynamics of complex, nonlinear systems.

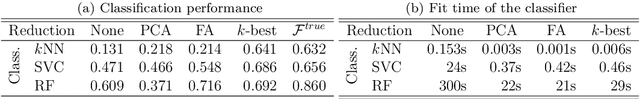
Feature space reduction method for ultrahigh-dimensional, multiclass data: Random forest-based multiround screening (RFMS)

In recent years, numerous screening methods have been published for ultrahigh-dimensional data that contain hundreds of thousands of features; however, most of these features cannot handle data with thousands of classes. Prediction models built to authenticate users based on multichannel biometric data result in this type of problem. In this study, we present a novel method known as random forest-based multiround screening (RFMS) that can be effectively applied under such circumstances. The proposed algorithm divides the feature space into small subsets and executes a series of partial model builds. These partial models are used to implement tournament-based sorting and the selection of features based on their importance. To benchmark RFMS, a synthetic biometric feature space generator known as BiometricBlender is employed. Based on the results, the RFMS is on par with industry-standard feature screening methods while simultaneously possessing many advantages over these methods.

BiometricBlender: Ultra-high dimensional, multi-class synthetic data generator to imitate biometric feature space

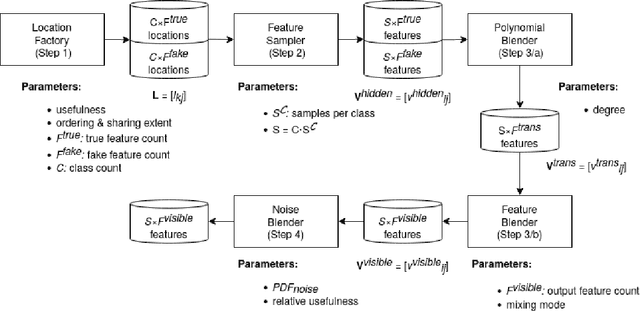
The lack of freely available (real-life or synthetic) high or ultra-high dimensional, multi-class datasets may hamper the rapidly growing research on feature screening, especially in the field of biometrics, where the usage of such datasets is common. This paper reports a Python package called BiometricBlender, which is an ultra-high dimensional, multi-class synthetic data generator to benchmark a wide range of feature screening methods. During the data generation process, the overall usefulness and the intercorrelations of blended features can be controlled by the user, thus the synthetic feature space is able to imitate the key properties of a real biometric dataset.

Manifold-adaptive dimension estimation revisited

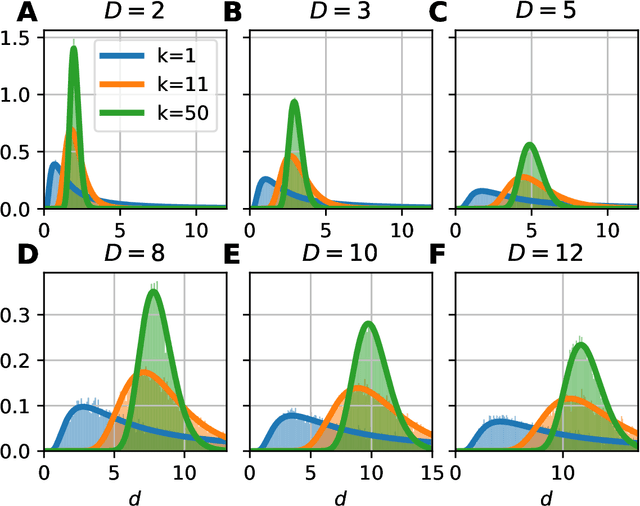
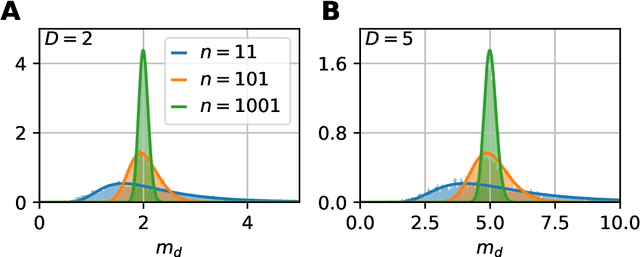
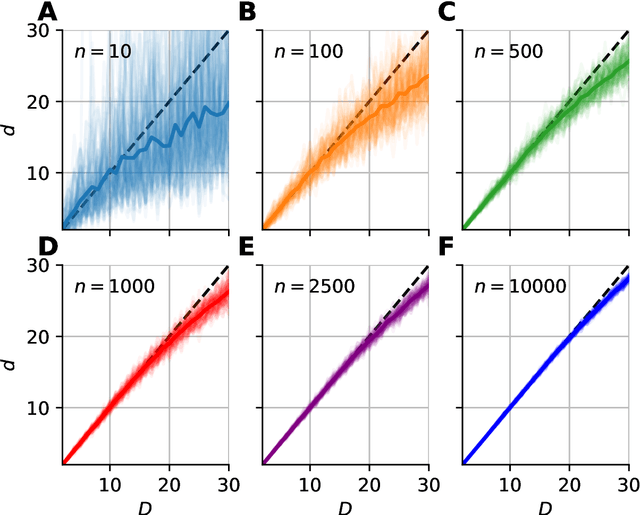
Data dimensionality informs us about data complexity and sets limit on the structure of successful signal processing pipelines. In this work we revisit and improve the manifold-adaptive Farahmand-Szepesv\'ari-Audibert (FSA) dimension estimator, making it one of the best nearest neighbor-based dimension estimators available. We compute the probability density function of local FSA estimates, if the local manifold density is uniform. Based on the probability density function, we propose to use the median of local estimates as a basic global measure of intrinsic dimensionality, and we demonstrate the advantages of this asymptotically unbiased estimator over the previously proposed statistics: the mode and the mean. Additionally, from the probability density function, we derive the maximum likelihood formula for global intrinsic dimensionality, if i.i.d. holds. We tackle edge and finite-sample effects with an exponential correction formula, calibrated on hypercube datasets. We compare the performance of the corrected-median-FSA estimator with kNN estimators: maximum likelihood (ML, Levina-Bickel) and two implementations of DANCo (R and matlab). We show that corrected-median-FSA estimator beats the ML estimator and it is on equal footing with DANCo for standard synthetic benchmarks according to mean percentage error and error rate metrics. With the median-FSA algorithm, we reveal diverse changes in the neural dynamics while resting state and during epileptic seizures. We identify brain areas with lower-dimensional dynamics that are possible causal sources and candidates for being seizure onset zones.