Markdowns in E-Commerce Fresh Retail: A Counterfactual Prediction and Multi-Period Optimization Approach

In this paper, by leveraging abundant observational transaction data, we propose a novel data-driven and interpretable pricing approach for markdowns, consisting of counterfactual prediction and multi-period price optimization. Firstly, we build a semi-parametric structural model to learn individual price elasticity and predict counterfactual demand. This semi-parametric model takes advantage of both the predictability of nonparametric machine learning model and the interpretability of economic model. Secondly, we propose a multi-period dynamic pricing algorithm to maximize the overall profit of a perishable product over its finite selling horizon. Different with the traditional approaches that use the deterministic demand, we model the uncertainty of counterfactual demand since it inevitably has randomness in the prediction process. Based on the stochastic model, we derive a sequential pricing strategy by Markov decision process, and design a two-stage algorithm to solve it. The proposed algorithm is very efficient. It reduces the time complexity from exponential to polynomial. Experimental results show the advantages of our pricing algorithm, and the proposed framework has been successfully deployed to the well-known e-commerce fresh retail scenario - Freshippo.

Distributed Variational Bayesian Algorithms Over Sensor Networks

Distributed inference/estimation in Bayesian framework in the context of sensor networks has recently received much attention due to its broad applicability. The variational Bayesian (VB) algorithm is a technique for approximating intractable integrals arising in Bayesian inference. In this paper, we propose two novel distributed VB algorithms for general Bayesian inference problem, which can be applied to a very general class of conjugate-exponential models. In the first approach, the global natural parameters at each node are optimized using a stochastic natural gradient that utilizes the Riemannian geometry of the approximation space, followed by an information diffusion step for cooperation with the neighbors. In the second method, a constrained optimization formulation for distributed estimation is established in natural parameter space and solved by alternating direction method of multipliers (ADMM). An application of the distributed inference/estimation of a Bayesian Gaussian mixture model is then presented, to evaluate the effectiveness of the proposed algorithms. Simulations on both synthetic and real datasets demonstrate that the proposed algorithms have excellent performance, which are almost as good as the corresponding centralized VB algorithm relying on all data available in a fusion center.

Distributed Variational Bayesian Algorithms for Extended Object Tracking

This paper is concerned with the problem of distributed extended object tracking, which aims to collaboratively estimate the state and extension of an object by a network of nodes. In traditional tracking applications, most approaches consider an object as a point source of measurements due to limited sensor resolution capabilities. Recently, some studies consider the extended objects, which are spatially structured, i.e., multiple resolution cells are occupied by an object. In this setting, multiple measurements are generated by each object per time step. In this paper, we present a Bayesian model for extended object tracking problem in a sensor network. In this model, the object extension is represented by a symmetric positive definite random matrix, and we assume that the measurement noise exists but is unknown. Using this Bayesian model, we first propose a novel centralized algorithm for extended object tracking based on variational Bayesian methods. Then, we extend it to the distributed scenario based on the alternating direction method of multipliers (ADMM) technique. The proposed algorithms can simultaneously estimate the extended object state (the kinematic state and extension) and the measurement noise covariance. Simulations on both extended object tracking and group target tracking are given to verify the effectiveness of the proposed model and algorithms.

A Unified Framework for Marketing Budget Allocation

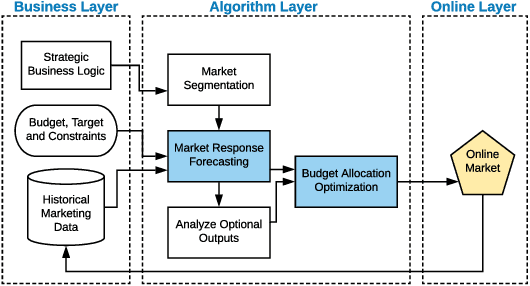
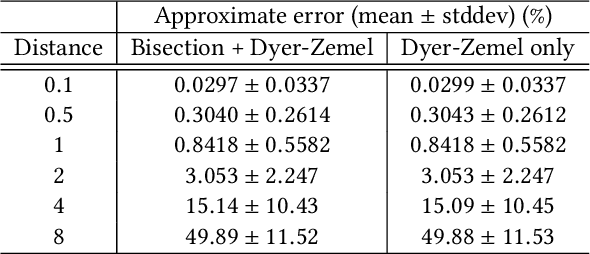
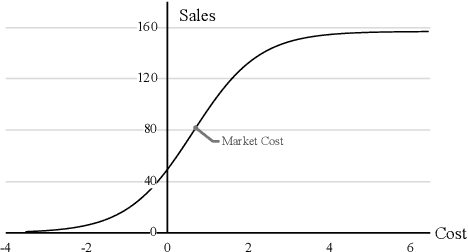
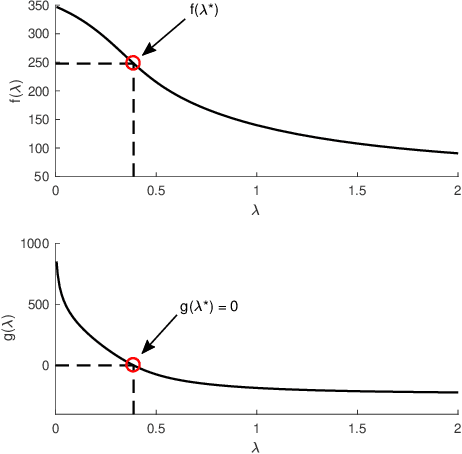
While marketing budget allocation has been studied for decades in traditional business, nowadays online business brings much more challenges due to the dynamic environment and complex decision-making process. In this paper, we present a novel unified framework for marketing budget allocation. By leveraging abundant data, the proposed data-driven approach can help us to overcome the challenges and make more informed decisions. In our approach, a semi-black-box model is built to forecast the dynamic market response and an efficient optimization method is proposed to solve the complex allocation task. First, the response in each market-segment is forecasted by exploring historical data through a semi-black-box model, where the capability of logit demand curve is enhanced by neural networks. The response model reveals relationship between sales and marketing cost. Based on the learned model, budget allocation is then formulated as an optimization problem, and we design efficient algorithms to solve it in both continuous and discrete settings. Several kinds of business constraints are supported in one unified optimization paradigm, including cost upper bound, profit lower bound, or ROI lower bound. The proposed framework is easy to implement and readily to handle large-scale problems. It has been successfully applied to many scenarios in Alibaba Group. The results of both offline experiments and online A/B testing demonstrate its effectiveness.