Corrector Operator to Enhance Accuracy and Reliability of Neural Operator Surrogates of Nonlinear Variational Boundary-Value Problems

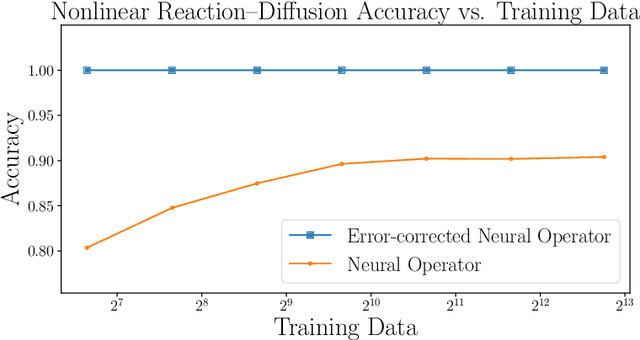
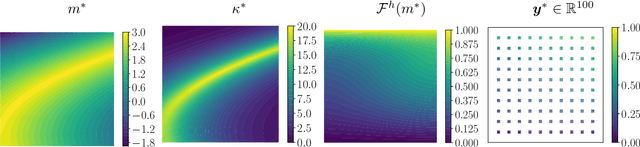
This work focuses on developing methods for approximating the solution operators of a class of parametric partial differential equations via neural operators. Neural operators have several challenges, including the issue of generating appropriate training data, cost-accuracy trade-offs, and nontrivial hyperparameter tuning. The unpredictability of the accuracy of neural operators impacts their applications in downstream problems of inference, optimization, and control. A framework is proposed based on the linear variational problem that gives the correction to the prediction furnished by neural operators. The operator associated with the corrector problem is referred to as the corrector operator. Numerical results involving a nonlinear diffusion model in two dimensions with PCANet-type neural operators show almost two orders of increase in the accuracy of approximations when neural operators are corrected using the proposed scheme. Further, topology optimization involving a nonlinear diffusion model is considered to highlight the limitations of neural operators and the efficacy of the correction scheme. Optimizers with neural operator surrogates are seen to make significant errors (as high as 80 percent). However, the errors are much lower (below 7 percent) when neural operators are corrected following the proposed method.

Residual-based error correction for neural operator accelerated infinite-dimensional Bayesian inverse problems

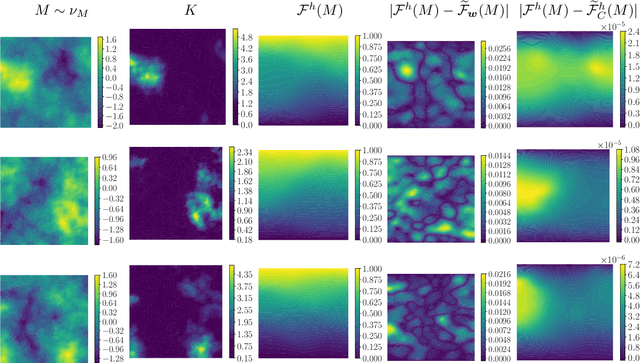
We explore using neural operators, or neural network representations of nonlinear maps between function spaces, to accelerate infinite-dimensional Bayesian inverse problems (BIPs) with models governed by nonlinear parametric partial differential equations (PDEs). Neural operators have gained significant attention in recent years for their ability to approximate the parameter-to-solution maps defined by PDEs using as training data solutions of PDEs at a limited number of parameter samples. The computational cost of BIPs can be drastically reduced if the large number of PDE solves required for posterior characterization are replaced with evaluations of trained neural operators. However, reducing error in the resulting BIP solutions via reducing the approximation error of the neural operators in training can be challenging and unreliable. We provide an a priori error bound result that implies certain BIPs can be ill-conditioned to the approximation error of neural operators, thus leading to inaccessible accuracy requirements in training. To reliably deploy neural operators in BIPs, we consider a strategy for enhancing the performance of neural operators, which is to correct the prediction of a trained neural operator by solving a linear variational problem based on the PDE residual. We show that a trained neural operator with error correction can achieve a quadratic reduction of its approximation error, all while retaining substantial computational speedups of posterior sampling when models are governed by highly nonlinear PDEs. The strategy is applied to two numerical examples of BIPs based on a nonlinear reaction--diffusion problem and deformation of hyperelastic materials. We demonstrate that posterior representations of the two BIPs produced using trained neural operators are greatly and consistently enhanced by error correction.

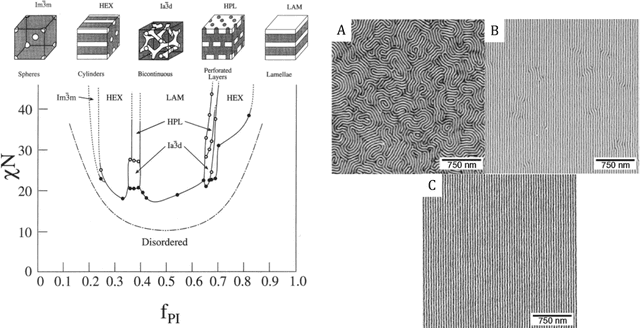
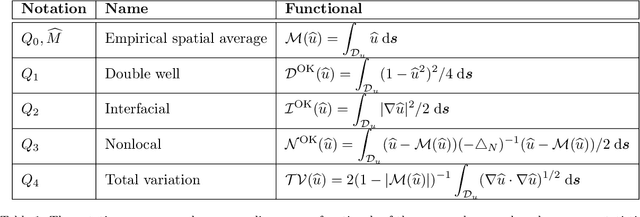
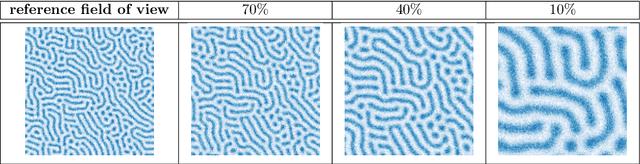
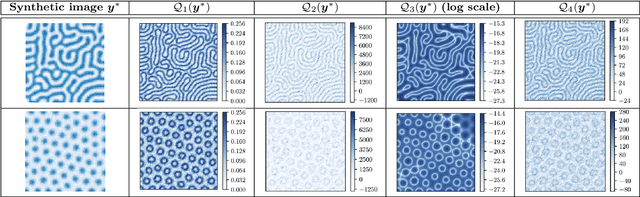
Bayesian model calibration for block copolymer self-assembly: Likelihood-free inference and expected information gain computation via measure transport

We consider the Bayesian calibration of models describing the phenomenon of block copolymer (BCP) self-assembly using image data produced by microscopy or X-ray scattering techniques. To account for the random long-range disorder in BCP equilibrium structures, we introduce auxiliary variables to represent this aleatory uncertainty. These variables, however, result in an integrated likelihood for high-dimensional image data that is generally intractable to evaluate. We tackle this challenging Bayesian inference problem using a likelihood-free approach based on measure transport together with the construction of summary statistics for the image data. We also show that expected information gains (EIGs) from the observed data about the model parameters can be computed with no significant additional cost. Lastly, we present a numerical case study based on the Ohta--Kawasaki model for diblock copolymer thin film self-assembly and top-down microscopy characterization. For calibration, we introduce several domain-specific energy- and Fourier-based summary statistics, and quantify their informativeness using EIG. We demonstrate the power of the proposed approach to study the effect of data corruptions and experimental designs on the calibration results.