A Fast Multitaper Power Spectrum Estimation in Nonuniformly Sampled Time Series

Nonuniformly sampled signals are prevalent in real-world applications but pose a significant challenge when estimating their power spectra from a finite number of samples of a single realization. The optimal solution using Bronez Generalized Prolate Spheroidal Sequence (GPSS) is computationally intensive and thus impractical for large datasets. This paper presents a fast nonparametric method, MultiTaper NonUniform Fast Fourier Transform (MTNUFFT), capable of estimating power spectra with lower computational burden. The method first derives a set of optimal tapers via cubic spline interpolation on a nominal analysis band, and subsequently shifts these tapers to other analysis bands using NonUniform FFT (NUFFT). The estimated spectral power within the band is the average power at the outputs of the taper set. This algorithm eliminates the time-consuming computation for solving the Generalized Eigenvalue Problem (GEP), thus reducing the computational load from $O(N^4)$ to $O(N \log N + N \log(1/\epsilon))$, comparable with the NUFFT. The statistical properties of the estimator are assessed using Bronez GPSS theory, revealing that the bias and variance bound of the MTNUFFT estimator are identical to those of the optimal estimator. Furthermore, the degradation of bias bound can serve as a measure of the deviation from optimality. The performance of the estimator is evaluated using both simulation and real-world data, demonstrating its practical applicability. The code of the proposed fast algorithm is available on GitHub (https://github.com/jiecui/mtnufft).

Reinforcement Learning based Disease Progression Model for Alzheimer's Disease

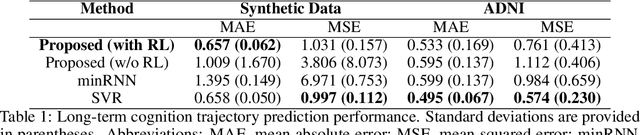
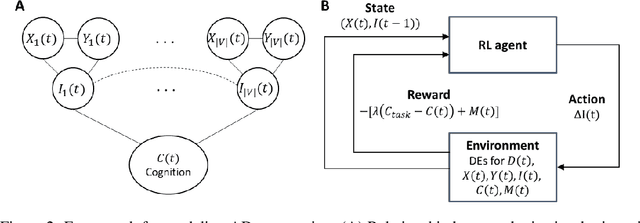
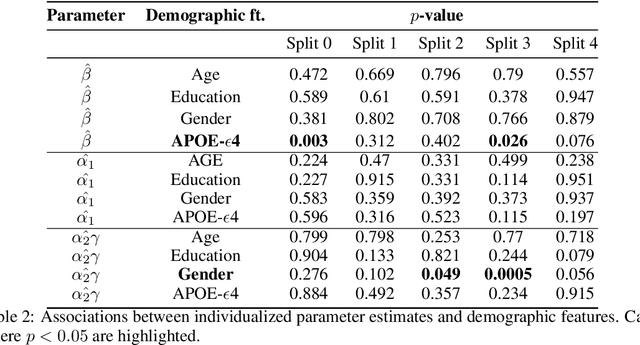
We model Alzheimer's disease (AD) progression by combining differential equations (DEs) and reinforcement learning (RL) with domain knowledge. DEs provide relationships between some, but not all, factors relevant to AD. We assume that the missing relationships must satisfy general criteria about the working of the brain, for e.g., maximizing cognition while minimizing the cost of supporting cognition. This allows us to extract the missing relationships by using RL to optimize an objective (reward) function that captures the above criteria. We use our model consisting of DEs (as a simulator) and the trained RL agent to predict individualized 10-year AD progression using baseline (year 0) features on synthetic and real data. The model was comparable or better at predicting 10-year cognition trajectories than state-of-the-art learning-based models. Our interpretable model demonstrated, and provided insights into, "recovery/compensatory" processes that mitigate the effect of AD, even though those processes were not explicitly encoded in the model. Our framework combines DEs with RL for modelling AD progression and has broad applicability for understanding other neurological disorders.