Scattering Spectra Models for Physics

Physicists routinely need probabilistic models for a number of tasks such as parameter inference or the generation of new realizations of a field. Establishing such models for highly non-Gaussian fields is a challenge, especially when the number of samples is limited. In this paper, we introduce scattering spectra models for stationary fields and we show that they provide accurate and robust statistical descriptions of a wide range of fields encountered in physics. These models are based on covariances of scattering coefficients, i.e. wavelet decomposition of a field coupled with a point-wise modulus. After introducing useful dimension reductions taking advantage of the regularity of a field under rotation and scaling, we validate these models on various multi-scale physical fields and demonstrate that they reproduce standard statistics, including spatial moments up to 4th order. These scattering spectra provide us with a low-dimensional structured representation that captures key properties encountered in a wide range of physical fields. These generic models can be used for data exploration, classification, parameter inference, symmetry detection, and component separation.

Martian time-series unraveled: A multi-scale nested approach with factorial variational autoencoders

Unsupervised source separation involves unraveling an unknown set of source signals recorded through a mixing operator, with limited prior knowledge about the sources, and only access to a dataset of signal mixtures. This problem is inherently ill-posed and is further challenged by the variety of time-scales exhibited by sources in time series data. Existing methods typically rely on a preselected window size that limits their capacity to handle multi-scale sources. To address this issue, instead of operating in the time domain, we propose an unsupervised multi-scale clustering and source separation framework by leveraging wavelet scattering covariances that provide a low-dimensional representation of stochastic processes, capable of distinguishing between different non-Gaussian stochastic processes. Nested within this representation space, we develop a factorial Gaussian-mixture variational autoencoder that is trained to (1) probabilistically cluster sources at different time-scales and (2) independently sample scattering covariance representations associated with each cluster. Using samples from each cluster as prior information, we formulate source separation as an optimization problem in the wavelet scattering covariance representation space, resulting in separated sources in the time domain. When applied to seismic data recorded during the NASA InSight mission on Mars, our multi-scale nested approach proves to be a powerful tool for discriminating between sources varying greatly in time-scale, e.g., minute-long transient one-sided pulses (known as ``glitches'') and structured ambient noises resulting from atmospheric activities that typically last for tens of minutes. These results provide an opportunity to conduct further investigations into the isolated sources related to atmospheric-surface interactions, thermal relaxations, and other complex phenomena.

Unearthing InSights into Mars: unsupervised source separation with limited data

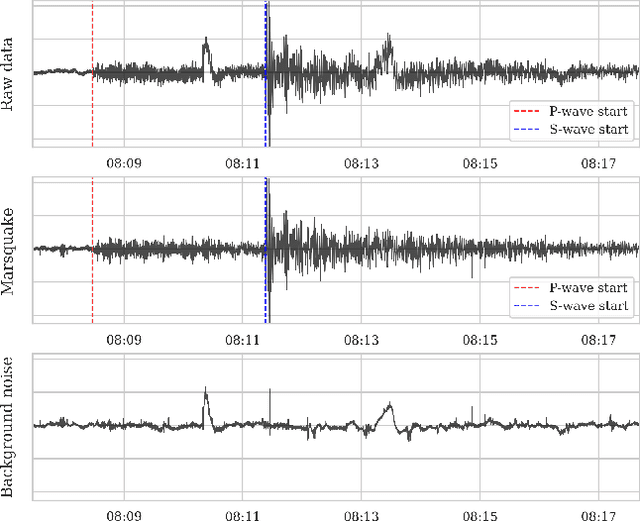
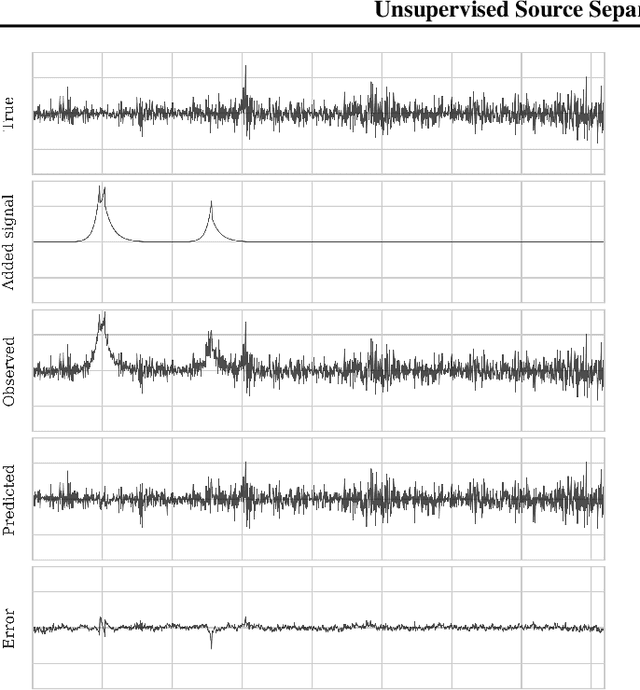
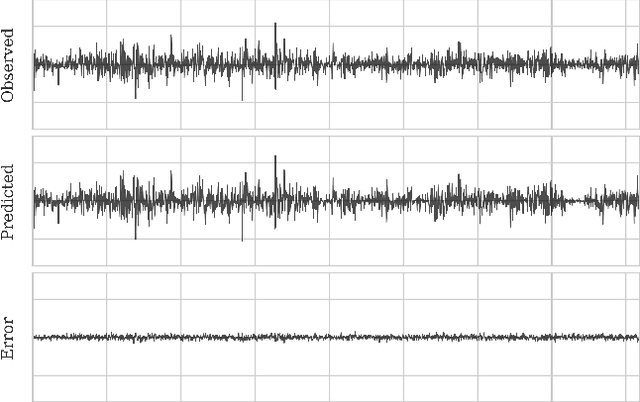
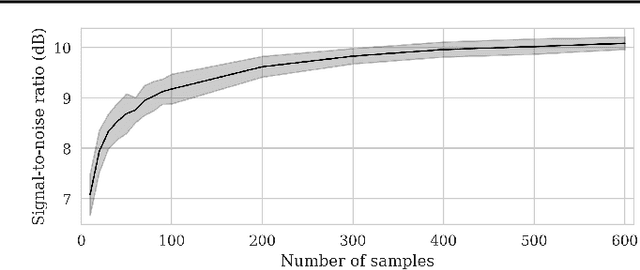
Source separation entails the ill-posed problem of retrieving a set of source signals observed through a mixing operator. Solving this problem requires prior knowledge, which is commonly incorporated by imposing regularity conditions on the source signals or implicitly learned in supervised or unsupervised methods from existing data. While data-driven methods have shown great promise in source separation, they are often dependent on large amounts of data, which rarely exists in planetary space missions. Considering this challenge, we propose an unsupervised source separation scheme for domains with limited data access that involves solving an optimization problem in the wavelet scattering representation space$\unicode{x2014}$an interpretable low-dimensional representation of stationary processes. We present a real-data example in which we remove transient thermally induced microtilts, known as glitches, from data recorded by a seismometer during NASA's InSight mission on Mars. Owing to the wavelet scattering covariances' ability to capture non-Gaussian properties of stochastic processes, we are able to separate glitches using only a few glitch-free data snippets.