Multi-Agent Q-Learning Dynamics in Random Networks: Convergence due to Exploration and Sparsity

Beyond specific settings, many multi-agent learning algorithms fail to converge to an equilibrium solution, and instead display complex, non-stationary behaviours such as recurrent or chaotic orbits. In fact, recent literature suggests that such complex behaviours are likely to occur when the number of agents increases. In this paper, we study Q-learning dynamics in network polymatrix games where the network structure is drawn from classical random graph models. In particular, we focus on the Erdos-Renyi model, a well-studied model for social networks, and the Stochastic Block model, which generalizes the above by accounting for community structures within the network. In each setting, we establish sufficient conditions under which the agents' joint strategies converge to a unique equilibrium. We investigate how this condition depends on the exploration rates, payoff matrices and, crucially, the sparsity of the network. Finally, we validate our theoretical findings through numerical simulations and demonstrate that convergence can be reliably achieved in many-agent systems, provided network sparsity is controlled.

WardropNet: Traffic Flow Predictions via Equilibrium-Augmented Learning

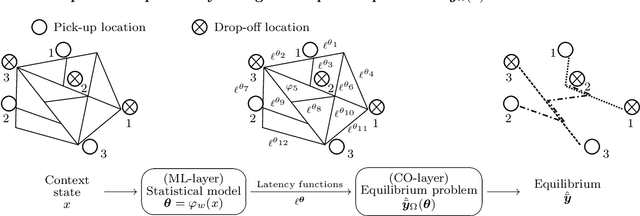
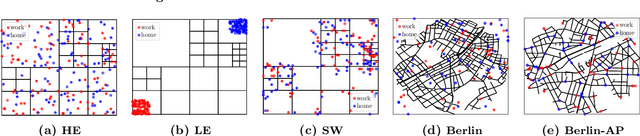
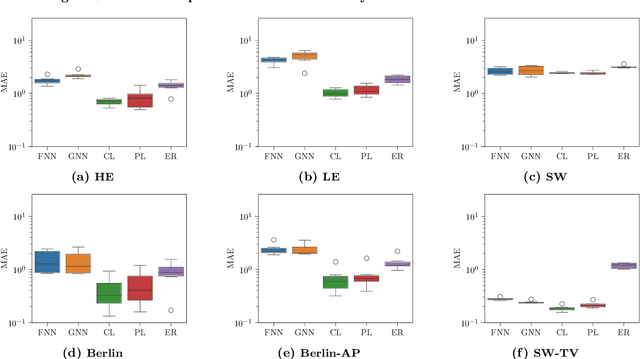
When optimizing transportation systems, anticipating traffic flows is a central element. Yet, computing such traffic equilibria remains computationally expensive. Against this background, we introduce a novel combinatorial optimization augmented neural network architecture that allows for fast and accurate traffic flow predictions. We propose WardropNet, a neural network that combines classical layers with a subsequent equilibrium layer: the first ones inform the latter by predicting the parameterization of the equilibrium problem's latency functions. Using supervised learning we minimize the difference between the actual traffic flow and the predicted output. We show how to leverage a Bregman divergence fitting the geometry of the equilibria, which allows for end-to-end learning. WardropNet outperforms pure learning-based approaches in predicting traffic equilibria for realistic and stylized traffic scenarios. On realistic scenarios, WardropNet improves on average for time-invariant predictions by up to 72% and for time-variant predictions by up to 23% over pure learning-based approaches.