DiffSim2Real: Deploying Quadrupedal Locomotion Policies Purely Trained in Differentiable Simulation

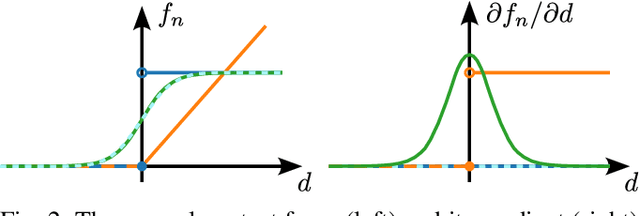
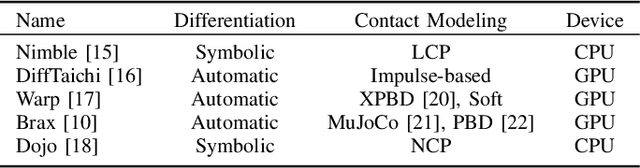
Differentiable simulators provide analytic gradients, enabling more sample-efficient learning algorithms and paving the way for data intensive learning tasks such as learning from images. In this work, we demonstrate that locomotion policies trained with analytic gradients from a differentiable simulator can be successfully transferred to the real world. Typically, simulators that offer informative gradients lack the physical accuracy needed for sim-to-real transfer, and vice-versa. A key factor in our success is a smooth contact model that combines informative gradients with physical accuracy, ensuring effective transfer of learned behaviors. To the best of our knowledge, this is the first time a real quadrupedal robot is able to locomote after training exclusively in a differentiable simulation.

Learning Quadrupedal Locomotion via Differentiable Simulation

The emergence of differentiable simulators enabling analytic gradient computation has motivated a new wave of learning algorithms that hold the potential to significantly increase sample efficiency over traditional Reinforcement Learning (RL) methods. While recent research has demonstrated performance gains in scenarios with comparatively smooth dynamics and, thus, smooth optimization landscapes, research on leveraging differentiable simulators for contact-rich scenarios, such as legged locomotion, is scarce. This may be attributed to the discontinuous nature of contact, which introduces several challenges to optimizing with analytic gradients. The purpose of this paper is to determine if analytic gradients can be beneficial even in the face of contact. Our investigation focuses on the effects of different soft and hard contact models on the learning process, examining optimization challenges through the lens of contact simulation. We demonstrate the viability of employing analytic gradients to learn physically plausible locomotion skills with a quadrupedal robot using Short-Horizon Actor-Critic (SHAC), a learning algorithm leveraging analytic gradients, and draw a comparison to a state-of-the-art RL algorithm, Proximal Policy Optimization (PPO), to understand the benefits of analytic gradients.