Brandon Kates
Privileged Zero-Shot AutoML
Jun 25, 2021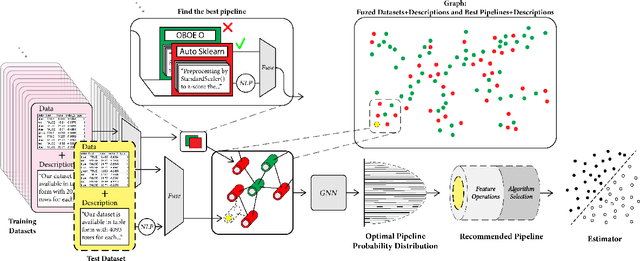
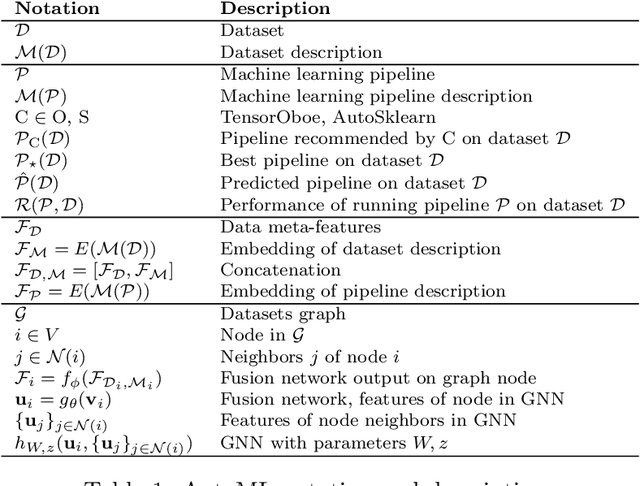
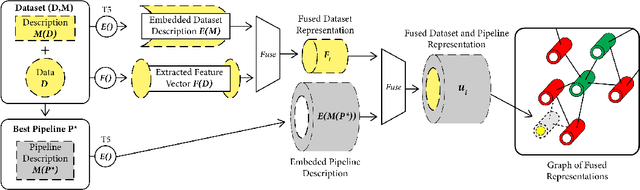
Abstract:This work improves the quality of automated machine learning (AutoML) systems by using dataset and function descriptions while significantly decreasing computation time from minutes to milliseconds by using a zero-shot approach. Given a new dataset and a well-defined machine learning task, humans begin by reading a description of the dataset and documentation for the algorithms to be used. This work is the first to use these textual descriptions, which we call privileged information, for AutoML. We use a pre-trained Transformer model to process the privileged text and demonstrate that using this information improves AutoML performance. Thus, our approach leverages the progress of unsupervised representation learning in natural language processing to provide a significant boost to AutoML. We demonstrate that using only textual descriptions of the data and functions achieves reasonable classification performance, and adding textual descriptions to data meta-features improves classification across tabular datasets. To achieve zero-shot AutoML we train a graph neural network with these description embeddings and the data meta-features. Each node represents a training dataset, which we use to predict the best machine learning pipeline for a new test dataset in a zero-shot fashion. Our zero-shot approach rapidly predicts a high-quality pipeline for a supervised learning task and dataset. In contrast, most AutoML systems require tens or hundreds of pipeline evaluations. We show that zero-shot AutoML reduces running and prediction times from minutes to milliseconds, consistently across datasets. By speeding up AutoML by orders of magnitude this work demonstrates real-time AutoML.
Learning to Solve Combinatorial Optimization Problems on Real-World Graphs in Linear Time
Jun 12, 2020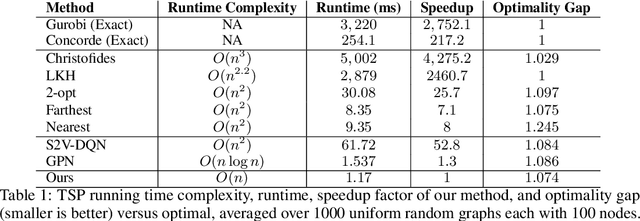
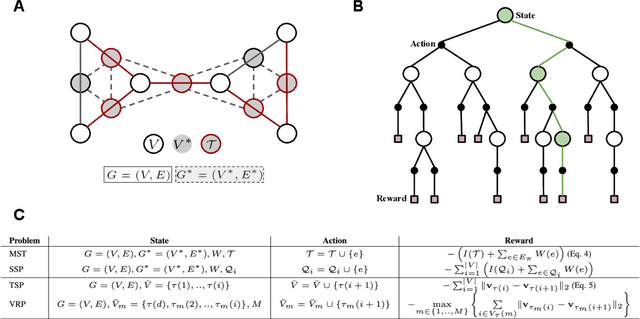
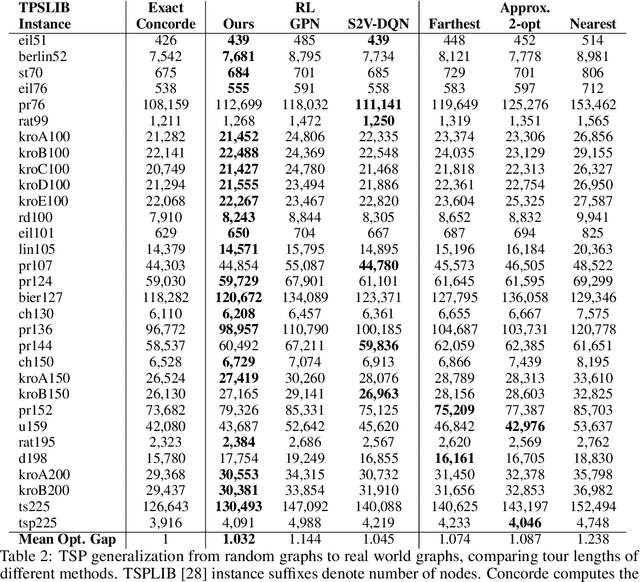
Abstract:Combinatorial optimization algorithms for graph problems are usually designed afresh for each new problem with careful attention by an expert to the problem structure. In this work, we develop a new framework to solve any combinatorial optimization problem over graphs that can be formulated as a single player game defined by states, actions, and rewards, including minimum spanning tree, shortest paths, traveling salesman problem, and vehicle routing problem, without expert knowledge. Our method trains a graph neural network using reinforcement learning on an unlabeled training set of graphs. The trained network then outputs approximate solutions to new graph instances in linear running time. In contrast, previous approximation algorithms or heuristics tailored to NP-hard problems on graphs generally have at least quadratic running time. We demonstrate the applicability of our approach on both polynomial and NP-hard problems with optimality gaps close to 1, and show that our method is able to generalize well: (i) from training on small graphs to testing on large graphs; (ii) from training on random graphs of one type to testing on random graphs of another type; and (iii) from training on random graphs to running on real world graphs.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge