Addressing Pilot Contamination in Channel Estimation with Variational Autoencoders

Pilot contamination (PC) is a well-known problem that affects massive multiple-input multiple-output (MIMO) systems. When frequency and pilots are reused between different cells, PC constitutes one of the main bottlenecks of the system's performance. In this paper, we propose a method based on the variational autoencoder (VAE), capable of reducing the impact of PC-related interference during channel estimation (CE). We obtain the first and second-order statistics of the conditionally Gaussian (CG) channels for both the user equipments (UEs) in a cell of interest and those in interfering cells, and we then use these moments to compute conditional linear minimum mean square error estimates. We show that the proposed estimator is capable of exploiting the interferers' additional statistical knowledge, outperforming other classical approaches. Moreover, we highlight how the achievable performance is tied to the chosen setup, making the setup selection crucial in the study of multi-cell CE.

A Versatile Pilot Design Scheme for FDD Systems Utilizing Gaussian Mixture Models

In this work, we propose a Gaussian mixture model (GMM)-based pilot design scheme for downlink (DL) channel estimation in single- and multi-user multiple-input multiple-output (MIMO) frequency division duplex (FDD) systems. In an initial offline phase, the GMM captures prior information during training, which is then utilized for pilot design. In the single-user case, the GMM is utilized to construct a codebook of pilot matrices and, once shared with the mobile terminal (MT), can be employed to determine a feedback index at the MT. This index selects a pilot matrix from the constructed codebook, eliminating the need for online pilot optimization. We further establish a sum conditional mutual information (CMI)-based pilot optimization framework for multi-user MIMO (MU-MIMO) systems. Based on the established framework, we utilize the GMM for pilot matrix design in MU-MIMO systems. The analytic representation of the GMM enables the adaptation to any signal-to-noise ratio (SNR) level and pilot configuration without re-training. Additionally, an adaption to any number of MTs is facilitated. Extensive simulations demonstrate the superior performance of the proposed pilot design scheme compared to state-of-the-art approaches. The performance gains can be exploited, e.g., to deploy systems with fewer pilots.

Linear and Nonlinear MMSE Estimation in One-Bit Quantized Systems under a Gaussian Mixture Prior

We present new fundamental results for the mean square error (MSE)-optimal conditional mean estimator (CME) in one-bit quantized systems for a Gaussian mixture model (GMM) distributed signal of interest, possibly corrupted by additive white Gaussian noise (AWGN). We first derive novel closed-form analytic expressions for the Bussgang estimator, the well-known linear minimum mean square error (MMSE) estimator in quantized systems. Afterward, closed-form analytic expressions for the CME in special cases are presented, revealing that the optimal estimator is linear in the one-bit quantized observation, opposite to higher resolution cases. Through a comparison to the recently studied Gaussian case, we establish a novel MSE inequality and show that that the signal of interest is correlated with the auxiliary quantization noise. We extend our analysis to multiple observation scenarios, examining the MSE-optimal transmit sequence and conducting an asymptotic analysis, yielding analytic expressions for the MSE and its limit. These contributions have broad impact for the analysis and design of various signal processing applications.

High-SNR Comparison of Linear Precoding and DPC in RIS-Aided MIMO Broadcast Channels

We compare dirty paper coding (DPC) and linear precoding methods in a reconfigurable intelligent surface (RIS)- aided high-signal-to-noise ratio (SNR) scenario, where the channel between the base station (BS) and the RIS is dominated by a line-of-sight (LOS) component. Furthermore, we consider two groups of users where one group can be efficiently served by the BS, whereas the other one has a negligible direct channel and has to be served via the RIS. These considerations allow us to analytically show fundamental differences between DPC and linear methods. In particular, our analysis addresses two essential aspects, i.e., the orthogonality of the BS-RIS channel with the direct channel and an interference term that is present only for linear precoding techniques. The interference term leads to strong limitations for the linear method, especially for random or statistical phase shifts. Moreover, we discuss under which circumstances this interference term is negligible and in which scenarios DPC and linear precoding lead to the same performance.

Enhancing Channel Estimation in Quantized Systems with a Generative Prior

Channel estimation in quantized systems is challenging, particularly in low-resolution systems. In this work, we propose to leverage a Gaussian mixture model (GMM) as generative prior, capturing the channel distribution of the propagation environment, to enhance a classical estimation technique based on the expectation-maximization (EM) algorithm for one-bit quantization. Thereby, a maximum a posteriori (MAP) estimate of the most responsible mixture component is inferred for a quantized received signal, which is subsequently utilized in the EM algorithm as side information. Numerical results demonstrate the significant performance improvement of our proposed approach over both a simplistic Gaussian prior and current state-of-the-art channel estimators. Furthermore, the proposed estimation framework exhibits adaptability to higher resolution systems and alternative generative priors.

Channel-Adaptive Pilot Design for FDD-MIMO Systems Utilizing Gaussian Mixture Models

In this work, we propose to utilize Gaussian mixture models (GMMs) to design pilots for downlink (DL) channel estimation in frequency division duplex (FDD) systems. The GMM captures prior information during training that is leveraged to design a codebook of pilot matrices in an initial offline phase. Once shared with the mobile terminal (MT), the GMM is utilized to determine a feedback index at the MT in the online phase. This index selects a pilot matrix from a codebook, eliminating the need for online pilot optimization. The GMM is further used for DL channel estimation at the MT via observation-dependent linear minimum mean square error (LMMSE) filters, parametrized by the GMM. The analytic representation of the GMM allows adaptation to any signal-to-noise ratio (SNR) level and pilot configuration without re-training. With extensive simulations, we demonstrate the superior performance of the proposed GMM-based pilot scheme compared to state-of-the-art approaches.

Diffusion-based Generative Prior for Low-Complexity MIMO Channel Estimation

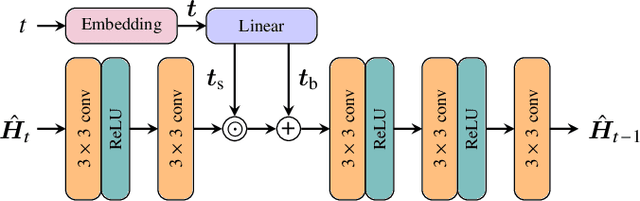
This work proposes a novel channel estimator based on diffusion models (DMs), one of the currently top-rated generative models. Contrary to related works utilizing generative priors, a lightweight convolutional neural network (CNN) with positional embedding of the signal-to-noise ratio (SNR) information is designed by learning the channel distribution in the sparse angular domain. Combined with an estimation strategy that avoids stochastic resampling and truncates reverse diffusion steps that account for lower SNR than the given pilot observation, the resulting DM estimator has both low complexity and memory overhead. Numerical results exhibit better performance than state-of-the-art channel estimators utilizing generative priors.

On the Asymptotic Mean Square Error Optimality of Diffusion Probabilistic Models

Diffusion probabilistic models (DPMs) have recently shown great potential for denoising tasks. Despite their practical utility, there is a notable gap in their theoretical understanding. This paper contributes novel theoretical insights by rigorously proving the asymptotic convergence of a specific DPM denoising strategy to the mean square error (MSE)-optimal conditional mean estimator (CME) over a large number of diffusion steps. The studied DPM-based denoiser shares the training procedure of DPMs but distinguishes itself by forwarding only the conditional mean during the reverse inference process after training. We highlight the unique perspective that DPMs are composed of an asymptotically optimal denoiser while simultaneously inheriting a powerful generator by switching re-sampling in the reverse process on and off. The theoretical findings are validated by numerical results.

Wireless Channel Prediction via Gaussian Mixture Models

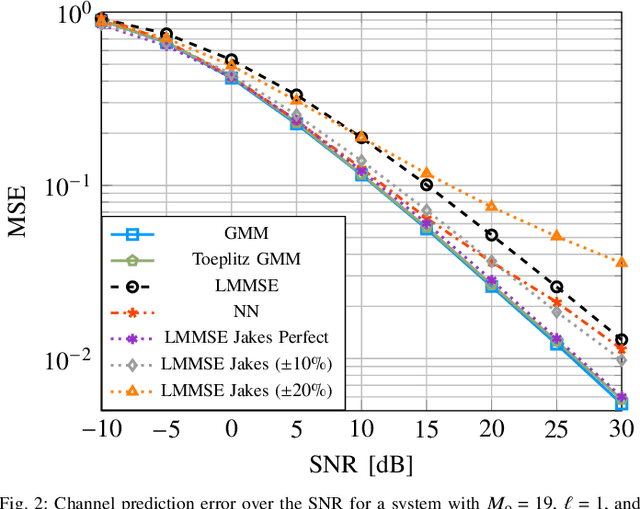
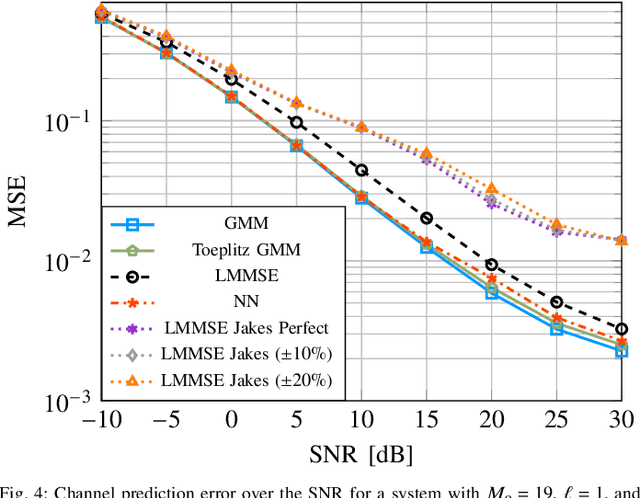
In this work, we utilize a Gaussian mixture model (GMM) to capture the underlying probability density function (PDF) of the channel trajectories of moving mobile terminals (MTs) within the coverage area of a base station (BS) in an offline phase. We propose to leverage the same GMM for channel prediction in the online phase. Our proposed approach does not require signal-to-noise ratio (SNR)-specific training and allows for parallelization. Numerical simulations for both synthetic and measured channel data demonstrate the effectiveness of our proposed GMM-based channel predictor compared to state-ofthe-art channel prediction methods.

Limited Feedback on Measurements: Sharing a Codebook or a Generative Model?

Discrete Fourier transform (DFT) codebook-based solutions are well-established for limited feedback schemes in frequency division duplex (FDD) systems. In recent years, data-aided solutions have been shown to achieve higher performance, enabled by the adaptivity of the feedback scheme to the propagation environment of the base station (BS) cell. In particular, a versatile limited feedback scheme utilizing Gaussian mixture models (GMMs) was recently introduced. The scheme supports multi-user communications, exhibits low complexity, supports parallelization, and offers significant flexibility concerning various system parameters. Conceptually, a GMM captures environment knowledge and is subsequently transferred to the mobile terminals (MTs) for online inference of feedback information. Afterward, the BS designs precoders using either directional information or a generative modeling-based approach. A major shortcoming of recent works is that the assessed system performance is only evaluated through synthetic simulation data that is generally unable to fully characterize the features of real-world environments. It raises the question of how the GMM-based feedback scheme performs on real-world measurement data, especially compared to the well-established DFT-based solution. Our experiments reveal that the GMM-based feedback scheme tremendously improves the system performance measured in terms of sum-rate, allowing to deploy systems with fewer pilots or feedback bits.