Stationary Kernels and Gaussian Processes on Lie Groups and their Homogeneous Spaces II: non-compact symmetric spaces

Gaussian processes are arguably the most important class of spatiotemporal models within machine learning. They encode prior information about the modeled function and can be used for exact or approximate Bayesian learning. In many applications, particularly in physical sciences and engineering, but also in areas such as geostatistics and neuroscience, invariance to symmetries is one of the most fundamental forms of prior information one can consider. The invariance of a Gaussian process' covariance to such symmetries gives rise to the most natural generalization of the concept of stationarity to such spaces. In this work, we develop constructive and practical techniques for building stationary Gaussian processes on a very large class of non-Euclidean spaces arising in the context of symmetries. Our techniques make it possible to (i) calculate covariance kernels and (ii) sample from prior and posterior Gaussian processes defined on such spaces, both in a practical manner. This work is split into two parts, each involving different technical considerations: part I studies compact spaces, while part II studies non-compact spaces possessing certain structure. Our contributions make the non-Euclidean Gaussian process models we study compatible with well-understood computational techniques available in standard Gaussian process software packages, thereby making them accessible to practitioners.

On power sum kernels on symmetric groups

In this note, we introduce a family of "power sum" kernels and the corresponding Gaussian processes on symmetric groups $\mathrm{S}_n$. Such processes are bi-invariant: the action of $\mathrm{S}_n$ on itself from both sides does not change their finite-dimensional distributions. We show that the values of power sum kernels can be efficiently calculated, and we also propose a method enabling approximate sampling of the corresponding Gaussian processes with polynomial computational complexity. By doing this we provide the tools that are required to use the introduced family of kernels and the respective processes for statistical modeling and machine learning.

Stationary Kernels and Gaussian Processes on Lie Groups and their Homogeneous Spaces I: the Compact Case

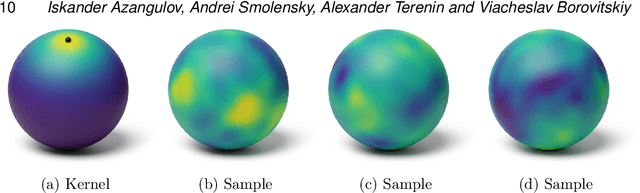
Gaussian processes are arguably the most important model class in spatial statistics. They encode prior information about the modeled function and can be used for exact or approximate Bayesian inference. In many applications, particularly in physical sciences and engineering, but also in areas such as geostatistics and neuroscience, invariance to symmetries is one of the most fundamental forms of prior information one can consider. The invariance of a Gaussian process' covariance to such symmetries gives rise to the most natural generalization of the concept of stationarity to such spaces. In this work, we develop constructive and practical techniques for building stationary Gaussian processes on a very large class of non-Euclidean spaces arising in the context of symmetries. Our techniques make it possible to (i) calculate covariance kernels and (ii) sample from prior and posterior Gaussian processes defined on such spaces, both in a practical manner. This work is split into two parts, each involving different technical considerations: part I studies compact spaces, while part II studies non-compact spaces possessing certain structure. Our contributions make the non-Euclidean Gaussian process models we study compatible with well-understood computational techniques available in standard Gaussian process software packages, thereby making them accessible to practitioners.

Geometry-aware Bayesian Optimization in Robotics using Riemannian Matérn Kernels

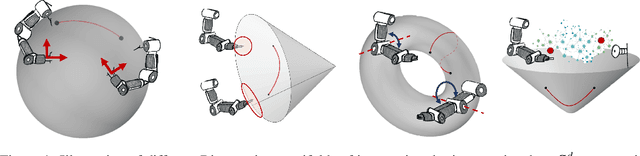
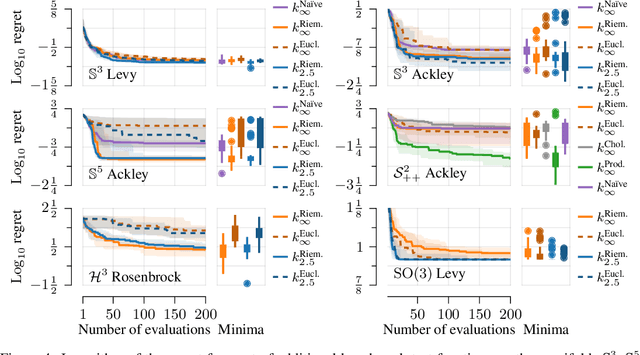
Bayesian optimization is a data-efficient technique which can be used for control parameter tuning, parametric policy adaptation, and structure design in robotics. Many of these problems require optimization of functions defined on non-Euclidean domains like spheres, rotation groups, or spaces of positive-definite matrices. To do so, one must place a Gaussian process prior, or equivalently define a kernel, on the space of interest. Effective kernels typically reflect the geometry of the spaces they are defined on, but designing them is generally non-trivial. Recent work on the Riemannian Mat\'ern kernels, based on stochastic partial differential equations and spectral theory of the Laplace-Beltrami operator, offers promising avenues towards constructing such geometry-aware kernels. In this paper, we study techniques for implementing these kernels on manifolds of interest in robotics, demonstrate their performance on a set of artificial benchmark functions, and illustrate geometry-aware Bayesian optimization for a variety of robotic applications, covering orientation control, manipulability optimization, and motion planning, while showing its improved performance.