High-dimensional additive Gaussian processes under monotonicity constraints

We introduce an additive Gaussian process framework accounting for monotonicity constraints and scalable to high dimensions. Our contributions are threefold. First, we show that our framework enables to satisfy the constraints everywhere in the input space. We also show that more general componentwise linear inequality constraints can be handled similarly, such as componentwise convexity. Second, we propose the additive MaxMod algorithm for sequential dimension reduction. By sequentially maximizing a squared-norm criterion, MaxMod identifies the active input dimensions and refines the most important ones. This criterion can be computed explicitly at a linear cost. Finally, we provide open-source codes for our full framework. We demonstrate the performance and scalability of the methodology in several synthetic examples with hundreds of dimensions under monotonicity constraints as well as on a real-world flood application.

Gaussian Process Modulated Cox Processes under Linear Inequality Constraints

Gaussian process (GP) modulated Cox processes are widely used to model point patterns. Existing approaches require a mapping (link function) between the unconstrained GP and the positive intensity function. This commonly yields solutions that do not have a closed form or that are restricted to specific covariance functions. We introduce a novel finite approximation of GP-modulated Cox processes where positiveness conditions can be imposed directly on the GP, with no restrictions on the covariance function. Our approach can also ensure other types of inequality constraints (e.g. monotonicity, convexity), resulting in more versatile models that can be used for other classes of point processes (e.g. renewal processes). We demonstrate on both synthetic and real-world data that our framework accurately infers the intensity functions. Where monotonicity is a feature of the process, our ability to include this in the inference improves results.

Approximating Gaussian Process Emulators with Linear Inequality Constraints and Noisy Observations via MC and MCMC

Adding inequality constraints (e.g. boundedness, monotonicity, convexity) into Gaussian processes (GPs) can lead to more realistic stochastic emulators. Due to the truncated Gaussianity of the posterior, its distribution has to be approximated. In this work, we consider Monte Carlo (MC) and Markov chain Monte Carlo (MCMC). However, strictly interpolating the observations may entail expensive computations due to highly restrictive sample spaces. Having (constrained) GP emulators when data are actually noisy is also of interest. We introduce a noise term for the relaxation of the interpolation conditions, and we develop the corresponding approximation of GP emulators under linear inequality constraints. We show with various toy examples that the performance of MC and MCMC samplers improves when considering noisy observations. Finally, on a 5D monotonic example, we show that our framework still provides high effective sample rates with reasonable running times.

Physically-inspired Gaussian processes for transcriptional regulation in Drosophila melanogaster

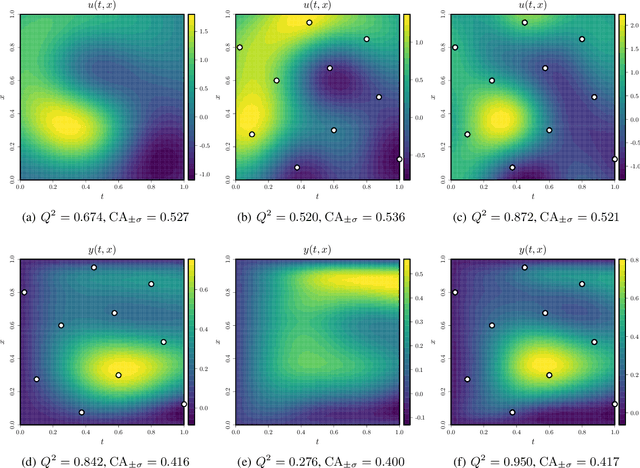
The regulatory process in Drosophila melanogaster is thoroughly studied for understanding several principles in systems biology. Since transcriptional regulation of the Drosophila depends on spatiotemporal interactions between mRNA expressions and gap-gene proteins, proper physically-inspired stochastic models are required to describe the existing link between both biological quantities. Many studies have shown that the use of Gaussian processes (GPs) and differential equations yields promising inference results when modelling regulatory processes. In order to exploit the benefits of GPs, two types of physically-inspired GPs based on the reaction-diffusion equation are further investigated in this paper. The main difference between both approaches lies on whether the GP prior is placed: either over mRNA expressions or protein concentrations. Contrarily to other stochastic frameworks, discretising the spatial space is not required here. Both GP models are tested under different conditions depending on the availability of biological data. Finally, their performances are assessed using a high-resolution dataset describing the blastoderm stage of the early embryo of Drosophila.

Switched latent force models for reverse-engineering transcriptional regulation in gene expression data

To survive environmental conditions, cells transcribe their response activities into encoded mRNA sequences in order to produce certain amounts of protein concentrations. The external conditions are mapped into the cell through the activation of special proteins called transcription factors (TFs). Due to the difficult task to measure experimentally TF behaviours, and the challenges to capture their quick-time dynamics, different types of models based on differential equations have been proposed. However, those approaches usually incur in costly procedures, and they present problems to describe sudden changes in TF regulators. In this paper, we present a switched dynamical latent force model for reverse-engineering transcriptional regulation in gene expression data which allows the exact inference over latent TF activities driving some observed gene expressions through a linear differential equation. To deal with discontinuities in the dynamics, we introduce an approach that switches between different TF activities and different dynamical systems. This creates a versatile representation of transcription networks that can capture discrete changes and non-linearities We evaluate our model on both simulated data and real-data (e.g. microaerobic shift in E. coli, yeast respiration), concluding that our framework allows for the fitting of the expression data while being able to infer continuous-time TF profiles.

Finite-dimensional Gaussian approximation with linear inequality constraints

Introducing inequality constraints in Gaussian process (GP) models can lead to more realistic uncertainties in learning a great variety of real-world problems. We consider the finite-dimensional Gaussian approach from Maatouk and Bay (2017) which can satisfy inequality conditions everywhere (either boundedness, monotonicity or convexity). Our contributions are threefold. First, we extend their approach in order to deal with general sets of linear inequalities. Second, we explore several Markov Chain Monte Carlo (MCMC) techniques to approximate the posterior distribution. Third, we investigate theoretical and numerical properties of the constrained likelihood for covariance parameter estimation. According to experiments on both artificial and real data, our full framework together with a Hamiltonian Monte Carlo-based sampler provides efficient results on both data fitting and uncertainty quantification.

Sparse Linear Models applied to Power Quality Disturbance Classification

Power quality (PQ) analysis describes the non-pure electric signals that are usually present in electric power systems. The automatic recognition of PQ disturbances can be seen as a pattern recognition problem, in which different types of waveform distortion are differentiated based on their features. Similar to other quasi-stationary signals, PQ disturbances can be decomposed into time-frequency dependent components by using time-frequency or time-scale transforms, also known as dictionaries. These dictionaries are used in the feature extraction step in pattern recognition systems. Short-time Fourier, Wavelets and Stockwell transforms are some of the most common dictionaries used in the PQ community, aiming to achieve a better signal representation. To the best of our knowledge, previous works about PQ disturbance classification have been restricted to the use of one among several available dictionaries. Taking advantage of the theory behind sparse linear models (SLM), we introduce a sparse method for PQ representation, starting from overcomplete dictionaries. In particular, we apply Group Lasso. We employ different types of time-frequency (or time-scale) dictionaries to characterize the PQ disturbances, and evaluate their performance under different pattern recognition algorithms. We show that the SLM reduce the PQ classification complexity promoting sparse basis selection, and improving the classification accuracy.