Semi-Supervised Few-Shot Intent Classification and Slot Filling

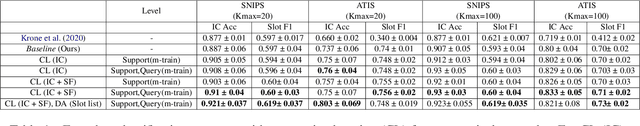
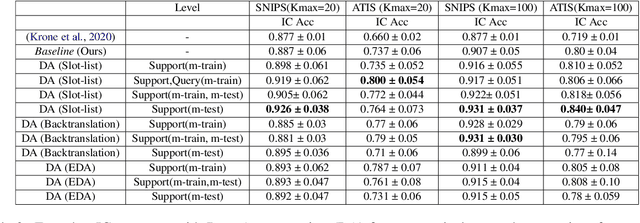
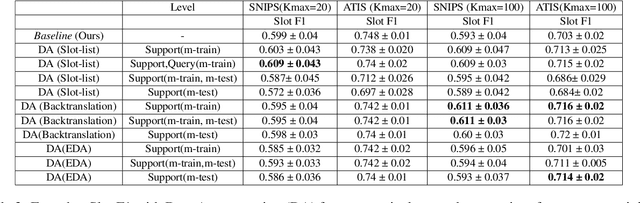
Intent classification (IC) and slot filling (SF) are two fundamental tasks in modern Natural Language Understanding (NLU) systems. Collecting and annotating large amounts of data to train deep learning models for such systems is not scalable. This problem can be addressed by learning from few examples using fast supervised meta-learning techniques such as prototypical networks. In this work, we systematically investigate how contrastive learning and unsupervised data augmentation methods can benefit these existing supervised meta-learning pipelines for jointly modelled IC/SF tasks. Through extensive experiments across standard IC/SF benchmarks (SNIPS and ATIS), we show that our proposed semi-supervised approaches outperform standard supervised meta-learning methods: contrastive losses in conjunction with prototypical networks consistently outperform the existing state-of-the-art for both IC and SF tasks, while data augmentation strategies primarily improve few-shot IC by a significant margin.

A Real-Time Solver For Time-Optimal Control Of Omnidirectional Robots with Bounded Acceleration

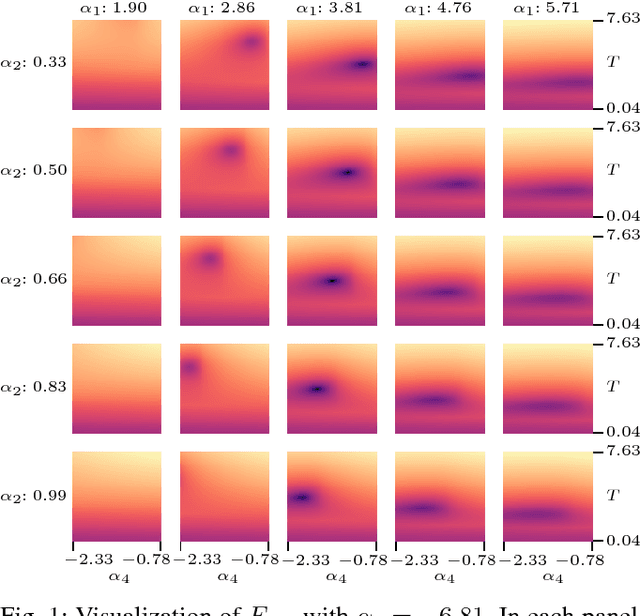
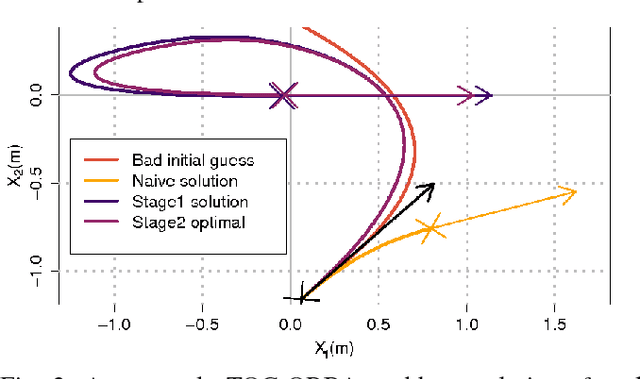
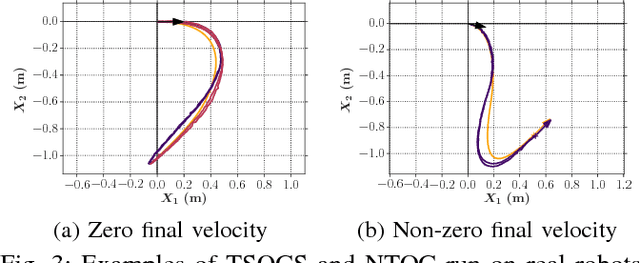
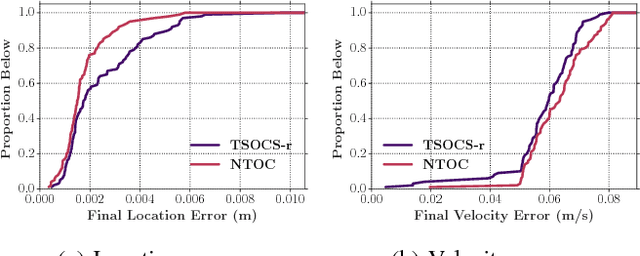
We are interested in the problem of time-optimal control of omnidirectional robots with bounded acceleration (TOC-ORBA). While there exist approximate solutions for such robots, and exact solutions with unbounded acceleration, exact solvers to the TOC-ORBA problem have remained elusive until now. In this paper, we present a real-time solver for true time-optimal control of omnidirectional robots with bounded acceleration. We first derive the general parameterized form of the solution to the TOC-ORBA problem by application of Pontryagin's maximum principle. We then frame the boundary value problem of TOC-ORBA as an optimization problem over the parametrized control space. To overcome local minima and poor initial guesses to the optimization problem, we introduce a two-stage optimal control solver (TSOCS): The first stage computes an upper bound to the total time for the TOC-ORBA problem and holds the time constant while optimizing the parameters of the trajectory to approach the boundary value conditions. The second stage uses the parameters found by the first stage, and relaxes the constraint on the total time to solve for the parameters of the complete TOC-ORBA problem. We further implement TSOCS as a closed loop controller to overcome actuation errors on real robots in real-time. We empirically demonstrate the effectiveness of TSOCS in simulation and on real robots, showing that 1) it runs in real time, generating solutions in less than 0.5ms on average; 2) it generates faster trajectories compared to an approximate solver; and 3) it is able to solve TOC-ORBA problems with non-zero final velocities that were previously unsolvable in real-time.