3D Correspondence Grouping with Compatibility Features

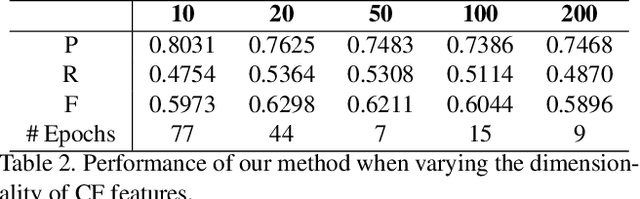
We present a simple yet effective method for 3D correspondence grouping. The objective is to accurately classify initial correspondences obtained by matching local geometric descriptors into inliers and outliers. Although the spatial distribution of correspondences is irregular, inliers are expected to be geometrically compatible with each other. Based on such observation, we propose a novel representation for 3D correspondences, dubbed compatibility feature (CF), to describe the consistencies within inliers and inconsistencies within outliers. CF consists of top-ranked compatibility scores of a candidate to other correspondences, which purely relies on robust and rotation-invariant geometric constraints. We then formulate the grouping problem as a classification problem for CF features, which is accomplished via a simple multilayer perceptron (MLP) network. Comparisons with nine state-of-the-art methods on four benchmarks demonstrate that: 1) CF is distinctive, robust, and rotation-invariant; 2) our CF-based method achieves the best overall performance and holds good generalization ability.