Variants of Tagged Sentential Decision Diagrams

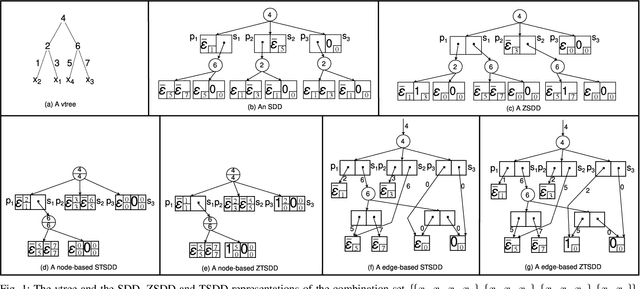
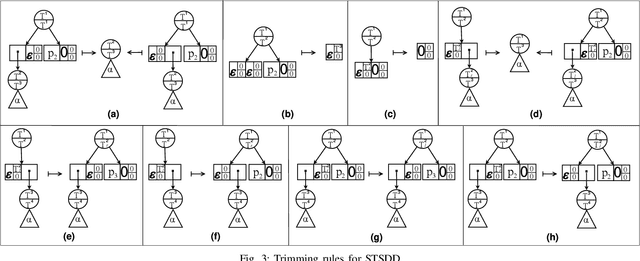
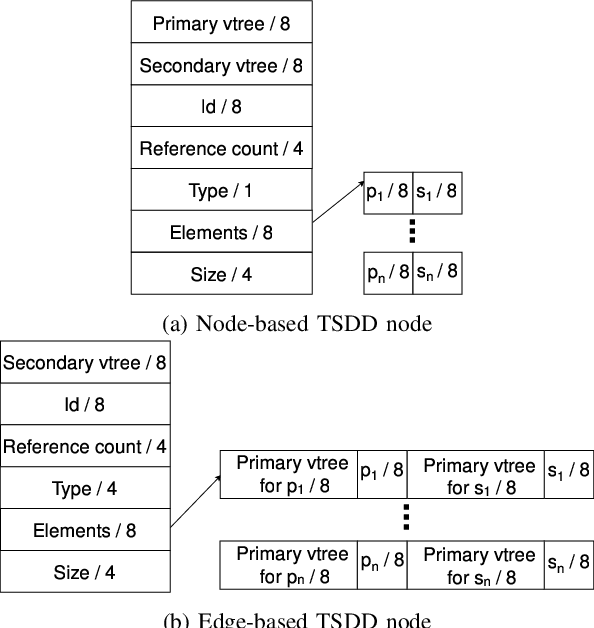
A recently proposed canonical form of Boolean functions, namely tagged sentential decision diagrams (TSDDs), exploits both the standard and zero-suppressed trimming rules. The standard ones minimize the size of sentential decision diagrams (SDDs) while the zero-suppressed trimming rules have the same objective as the standard ones but for zero-suppressed sentential decision diagrams (ZSDDs). The original TSDDs, which we call zero-suppressed TSDDs (ZTSDDs), firstly fully utilize the zero-suppressed trimming rules, and then the standard ones. In this paper, we present a variant of TSDDs which we call standard TSDDs (STSDDs) by reversing the order of trimming rules. We then prove the canonicity of STSDDs and present the algorithms for binary operations on TSDDs. In addition, we offer two kinds of implementations of STSDDs and ZTSDDs and acquire three variations of the original TSDDs. Experimental evaluations demonstrate that the four versions of TSDDs have the size advantage over SDDs and ZSDDs.

Dependence in Propositional Logic: Formula-Formula Dependence and Formula Forgetting -- Application to Belief Update and Conservative Extension

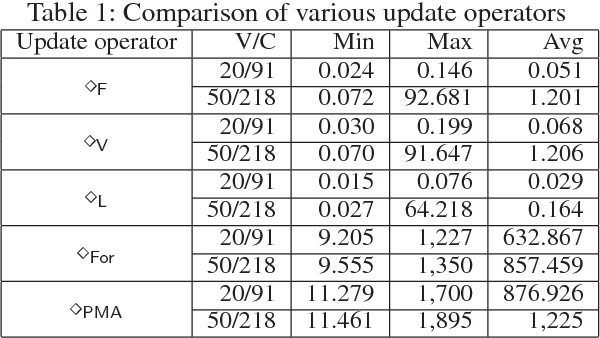
Dependence is an important concept for many tasks in artificial intelligence. A task can be executed more efficiently by discarding something independent from the task. In this paper, we propose two novel notions of dependence in propositional logic: formula-formula dependence and formula forgetting. The first is a relation between formulas capturing whether a formula depends on another one, while the second is an operation that returns the strongest consequence independent of a formula. We also apply these two notions in two well-known issues: belief update and conservative extension. Firstly, we define a new update operator based on formula-formula dependence. Furthermore, we reduce conservative extension to formula forgetting.