Physics-Constrained Generative Artificial Intelligence for Rapid Takeoff Trajectory Design

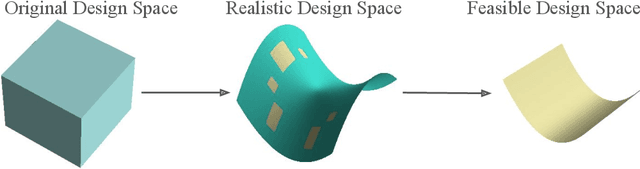
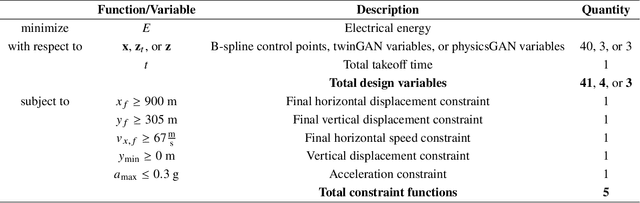
To aid urban air mobility (UAM), electric vertical takeoff and landing (eVTOL) aircraft are being targeted. Conventional multidisciplinary analysis and optimization (MDAO) can be expensive, while surrogate-based optimization can struggle with challenging physical constraints. This work proposes physics-constrained generative adversarial networks (physicsGAN), to intelligently parameterize the takeoff control profiles of an eVTOL aircraft and to transform the original design space to a feasible space. Specifically, the transformed feasible space refers to a space where all designs directly satisfy all design constraints. The physicsGAN-enabled surrogate-based takeoff trajectory design framework was demonstrated on the Airbus A3 Vahana. The physicsGAN generated only feasible control profiles of power and wing angle in the feasible space with around 98.9% of designs satisfying all constraints. The proposed design framework obtained 99.6% accuracy compared with simulation-based optimal design and took only 2.2 seconds, which reduced the computational time by around 200 times. Meanwhile, data-driven GAN-enabled surrogate-based optimization took 21.9 seconds using a derivative-free optimizer, which was around an order of magnitude slower than the proposed framework. Moreover, the data-driven GAN-based optimization using gradient-based optimizers could not consistently find the optimal design during random trials and got stuck in an infeasible region, which is problematic in real practice. Therefore, the proposed physicsGAN-based design framework outperformed data-driven GAN-based design to the extent of efficiency (2.2 seconds), optimality (99.6% accurate), and feasibility (100% feasible). According to the literature review, this is the first physics-constrained generative artificial intelligence enabled by surrogate models.

Machine Learning in Aerodynamic Shape Optimization

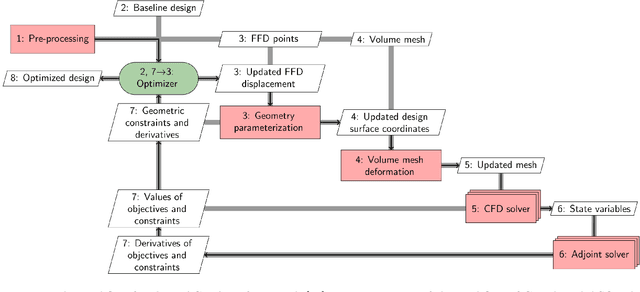
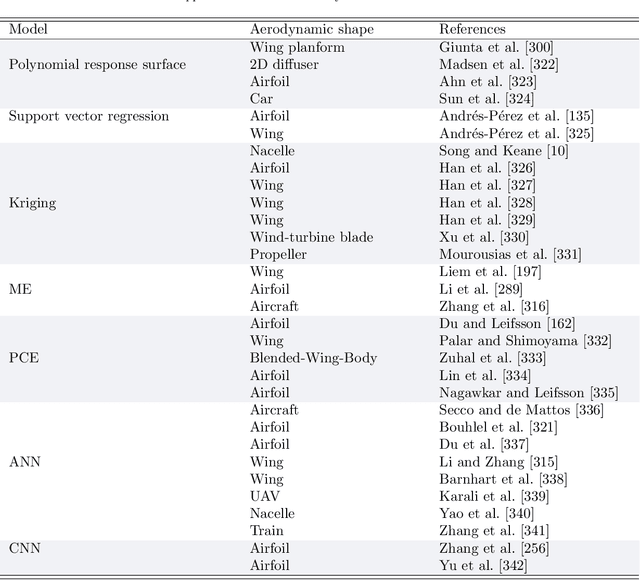
Large volumes of experimental and simulation aerodynamic data have been rapidly advancing aerodynamic shape optimization (ASO) via machine learning (ML), whose effectiveness has been growing thanks to continued developments in deep learning. In this review, we first introduce the state of the art and the unsolved challenges in ASO. Next, we present a description of ML fundamentals and detail the ML algorithms that have succeeded in ASO. Then we review ML applications contributing to ASO from three fundamental perspectives: compact geometric design space, fast aerodynamic analysis, and efficient optimization architecture. In addition to providing a comprehensive summary of the research, we comment on the practicality and effectiveness of the developed methods. We show how cutting-edge ML approaches can benefit ASO and address challenging demands like interactive design optimization. However, practical large-scale design optimizations remain a challenge due to the costly ML training expense. A deep coupling of ML model construction with ASO prior experience and knowledge, such as taking physics into account, is recommended to train ML models effectively.

Adaptive Projected Residual Networks for Learning Parametric Maps from Sparse Data

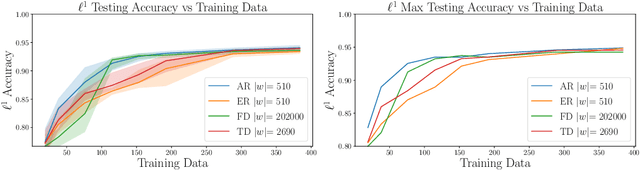
We present a parsimonious surrogate framework for learning high dimensional parametric maps from limited training data. The need for parametric surrogates arises in many applications that require repeated queries of complex computational models. These applications include such "outer-loop" problems as Bayesian inverse problems, optimal experimental design, and optimal design and control under uncertainty, as well as real time inference and control problems. Many high dimensional parametric mappings admit low dimensional structure, which can be exploited by mapping-informed reduced bases of the inputs and outputs. Exploiting this property, we develop a framework for learning low dimensional approximations of such maps by adaptively constructing ResNet approximations between reduced bases of their inputs and output. Motivated by recent approximation theory for ResNets as discretizations of control flows, we prove a universal approximation property of our proposed adaptive projected ResNet framework, which motivates a related iterative algorithm for the ResNet construction. This strategy represents a confluence of the approximation theory and the algorithm since both make use of sequentially minimizing flows. In numerical examples we show that these parsimonious, mapping-informed architectures are able to achieve remarkably high accuracy given few training data, making them a desirable surrogate strategy to be implemented for minimal computational investment in training data generation.