A Low-Dimensional Representation for Robust Partial Isometric Correspondences Computation

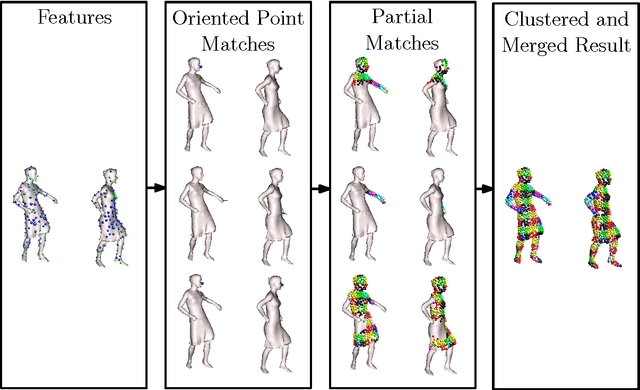
Intrinsic isometric shape matching has become the standard approach for pose invariant correspondence estimation among deformable shapes. Most existing approaches assume global consistency, i.e., the metric structure of the whole manifold must not change significantly. While global isometric matching is well understood, only a few heuristic solutions are known for partial matching. Partial matching is particularly important for robustness to topological noise (incomplete data and contacts), which is a common problem in real-world 3D scanner data. In this paper, we introduce a new approach to partial, intrinsic isometric matching. Our method is based on the observation that isometries are fully determined by purely local information: a map of a single point and its tangent space fixes an isometry for both global and the partial maps. From this idea, we develop a new representation for partial isometric maps based on equivalence classes of correspondences between pairs of points and their tangent spaces. From this, we derive a local propagation algorithm that find such mappings efficiently. In contrast to previous heuristics based on RANSAC or expectation maximization, our method is based on a simple and sound theoretical model and fully deterministic. We apply our approach to register partial point clouds and compare it to the state-of-the-art methods, where we obtain significant improvements over global methods for real-world data and stronger guarantees than previous heuristic partial matching algorithms.

Combinatorial Gradient Fields for 2D Images with Empirically Convergent Separatrices

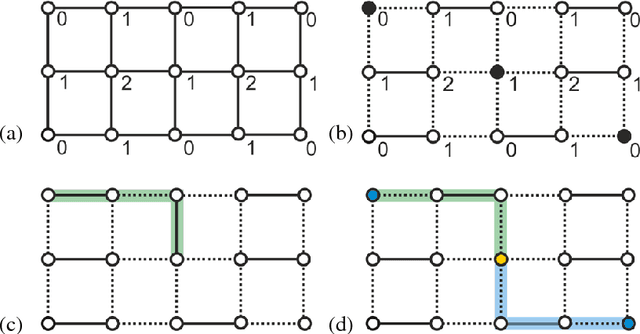
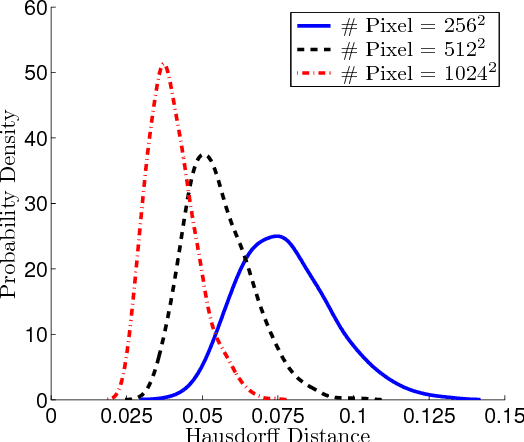
This paper proposes an efficient probabilistic method that computes combinatorial gradient fields for two dimensional image data. In contrast to existing algorithms, this approach yields a geometric Morse-Smale complex that converges almost surely to its continuous counterpart when the image resolution is increased. This approach is motivated using basic ideas from probability theory and builds upon an algorithm from discrete Morse theory with a strong mathematical foundation. While a formal proof is only hinted at, we do provide a thorough numerical evaluation of our method and compare it to established algorithms.